Search

Text Color

Margin Size

Font Type

Enable Dyslexic Font

3.8: El Microscopio Compuesto

Última actualización

30 oct 2022
Guardar como PDF
- 3.7: Oculares
- 3.9: El Telescopio

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\id}{\mathrm{id}}$ $\newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$ $\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$ $\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\id}{\mathrm{id}}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\kernel}{\mathrm{null}\,}$

$\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$

$\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$

$\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$ $\newcommand{\AA}{\unicode[.8,0]{x212B}}$

$\newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$\newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$\newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vectorC}[1]{\textbf{#1}}$

$\newcommand{\vectorD}[1]{\overrightarrow{#1}}$

$\newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}}$

$\newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}}$

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\avec}{\mathbf a}$

$\newcommand{\bvec}{\mathbf b}$

$\newcommand{\cvec}{\mathbf c}$

$\newcommand{\dvec}{\mathbf d}$

$\newcommand{\dtil}{\widetilde{\mathbf d}}$

$\newcommand{\evec}{\mathbf e}$

$\newcommand{\fvec}{\mathbf f}$

$\newcommand{\nvec}{\mathbf n}$

$\newcommand{\pvec}{\mathbf p}$

$\newcommand{\qvec}{\mathbf q}$

$\newcommand{\svec}{\mathbf s}$

$\newcommand{\tvec}{\mathbf t}$

$\newcommand{\uvec}{\mathbf u}$

$\newcommand{\vvec}{\mathbf v}$

$\newcommand{\wvec}{\mathbf w}$

$\newcommand{\xvec}{\mathbf x}$

$\newcommand{\yvec}{\mathbf y}$

$\newcommand{\zvec}{\mathbf z}$

$\newcommand{\rvec}{\mathbf r}$

$\newcommand{\mvec}{\mathbf m}$

$\newcommand{\zerovec}{\mathbf 0}$

$\newcommand{\onevec}{\mathbf 1}$

$\newcommand{\real}{\mathbb R}$

$\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$

$\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$

$\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$

$\newcommand{\laspan}[1]{\text{Span}\{#1\}}$

$\newcommand{\bcal}{\cal B}$

$\newcommand{\ccal}{\cal C}$

$\newcommand{\scal}{\cal S}$

$\newcommand{\wcal}{\cal W}$

$\newcommand{\ecal}{\cal E}$

$\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$

$\newcommand{\gray}[1]{\color{gray}{#1}}$

$\newcommand{\lgray}[1]{\color{lightgray}{#1}}$

$\newcommand{\rank}{\operatorname{rank}}$

$\newcommand{\row}{\text{Row}}$

$\newcommand{\col}{\text{Col}}$

$\renewcommand{\row}{\text{Row}}$

$\newcommand{\nul}{\text{Nul}}$

$\newcommand{\var}{\text{Var}}$

$\newcommand{\corr}{\text{corr}}$

$\newcommand{\len}[1]{\left|#1\right|}$

$\newcommand{\bbar}{\overline{\bvec}}$

$\newcommand{\bhat}{\widehat{\bvec}}$

$\newcommand{\bperp}{\bvec^\perp}$

$\newcommand{\xhat}{\widehat{\xvec}}$

$\newcommand{\vhat}{\widehat{\vvec}}$

$\newcommand{\uhat}{\widehat{\uvec}}$

$\newcommand{\what}{\widehat{\wvec}}$

$\newcommand{\Sighat}{\widehat{\Sigma}}$

$\newcommand{\lt}{<}$

$\newcommand{\gt}{>}$

$\newcommand{\amp}{&}$

$\definecolor{fillinmathshade}{gray}{0.9}$

Una lupa sola puede proporcionar un aumento muy alto solo a costa de aberraciones intolerables. El microscopio compuesto es una lupa de objetos cercanos con un aumento angular alto, generalmente más de $30 \times$ . Fue inventado por Zacharias Janssen en Middelburg en 1590 (esta afirmación se disputa). El primer elemento del microscopio compuesto es un objetivo (en la Figura $\PageIndex{1}$ una simple lente positiva) que realiza una imagen real, invertida y magnificada del objeto en el plano focal frontal de un ocular (donde también está el tope de campo). El ocular realizará una imagen virtual al infinito, como se explicó anteriormente. La potencia de aumento de todo el sistema es producto de la ampliación lineal transversal del objetivo $M_{T}$ y la ampliación angular del ocular $M_{A e}$ : $\mathrm{MP}=M_{T} M_{A e} . \nonumber$

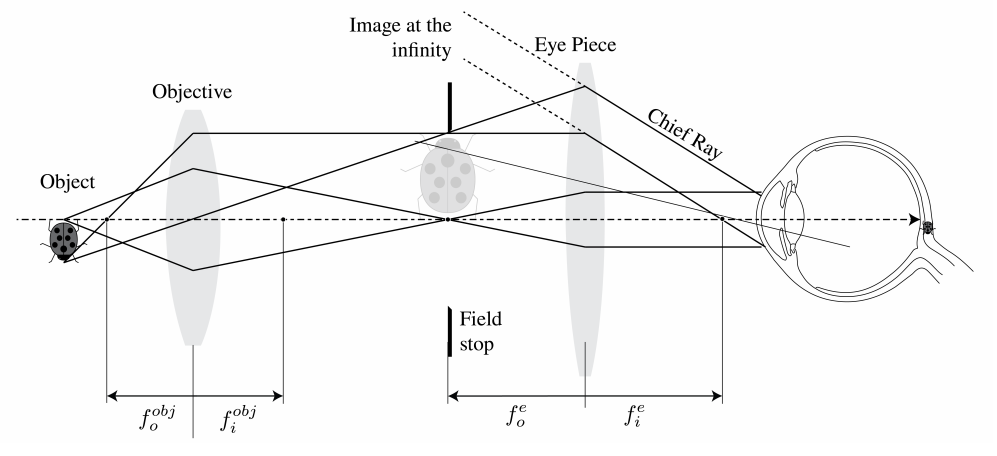
3.7.1.jpg — Figura $\PageIndex{1}$ : Microscopio compuesto simple. El objetivo forma una imagen real de un objeto cercano. El ocular agranda esta imagen intermedia. La imagen final puede ser más grande que el barril del dispositivo, ya que es virtual.

Según (2.5.51): $M_{T}=-x_{i} / f_{i}^{o b j}$ , donde $x_{i}$ está la distancia de la imagen realizada por el objetivo a su plano focal posterior con distancia focal $f_{i}^{\text {obj }}$ . Tenemos $x_{i}=L$ cual es la longitud del tubo, es decir, la distancia entre el segundo punto focal del objetivo y el primer punto focal del ocular. La longitud del tubo está estandarizada en $16 \mathrm{~cm}$ . Además, según (3.5.5), el aumento angular es para una imagen virtual al infinito $M_{A e}=d_{o} / f_{i}^{e}$ . De ahí que obtengamos: $\mathrm{MP}=\frac{-x_{i}}{f_{i}^{o b j}} \frac{d_{o}}{f_{i}^{e}}=\frac{-16}{f_{0}} \frac{25}{f_{e}}, \nonumber$ con el punto cercano estándar $d_{o}=25 \mathrm{~cm}$ . Como ejemplo, un objetivo de Amici da $40 \times$ y combinado con una pieza $10 \times$ ocular se obtiene $M P=400$ .

La apertura numérica de un microscopio es una medida de la capacidad de recoger luz del objeto. Se define por: $\mathrm{NA}=n_{i m} \sin \theta_{\max } \nonumber$ con $n_{\text {im }}$ el índice de refracción del medio de inmersión, generalmente aire, pero podría ser agua o aceite, y $\theta_{\max }$ , el medio ángulo del cono máximo de luz aceptado por la lente. La apertura numérica es el segundo número grabado en el barril del objetivo. Se extiende desde $0.07$ (objetivos de baja potencia) hasta objetivos $1.4$ de alta potencia. Tenga en cuenta que depende de la distancia del objeto. En el Capítulo 7 se explicará que NA es para la distancia de objeto dada proporcional al poder de resolución, que es la distancia transversal mínima entre dos puntos de objeto que pueden resolverse en la imagen.

Support Center

How can we help?