Apéndice 2: Elementos de Circuito Ideal

Resistor

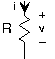
La resistencia es de lejos el elemento de circuito más simple. En una resistencia, el voltaje es proporcional a la corriente, con la constante de proporcionalidad RR, conocida como la resistencia. \[v(t) = R i(t)\]

La resistencia tiene unidades de ohmios, denotado por\(\mathrm{\Omega}\), llamado así por el científico eléctrico alemán Georg Ohm. En ocasiones, la\(v\text{-} i\) relación para la resistencia se escribe\(i = Gv\), con\(G\), la conductancia, igual a\(\frac{1}{R}\). Conductancia tiene unidades de Siemens\((\mathrm{S})\), y lleva el nombre del industrial alemán de electrónica Werner von Siemens.

Cuando la resistencia es positiva, como lo es en la mayoría de los casos, una resistencia consume energía. El consumo de energía instantáneo de una resistencia se puede escribir de dos maneras. \[p(t) = Ri^{2} (t) = \frac{1}{R} v^{2} (t)\]

A medida que la resistencia se acerca al infinito, tenemos lo que se conoce como un circuito abierto: no fluye corriente, pero puede aparecer un voltaje distinto de cero a través del circuito abierto. A medida que la resistencia se vuelve cero, el voltaje va a cero para un flujo de corriente distinto de cero. Esta situación corresponde a un cortocircuito. Un superconductor realiza físicamente un cortocircuito.

Capacitor

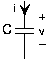
El condensador almacena carga y la relación entre la carga almacenada y el voltaje resultante es\(q = Cv\). La constante de proporcionalidad, la capacitancia, tiene unidades de faradios\((\mathrm{F})\), y lleva el nombre del físico experimental inglés Michael Faraday. Como corriente es la tasa de cambio de carga, la\(v \text{-} i\) relación se puede expresar en forma diferencial o integral. \[i(t) = C \frac{\text{d} v(t)}{\text{d}t}\]\[v(t) = \frac{1}{C} \int\limits_{-\infty}^{t} i (\alpha) \ \text{d} \alpha\]Si el voltaje a través de un condensador es constante, entonces la corriente que fluye hacia él es igual a cero. En esta situación, el condensador es equivalente a un circuito abierto. La potencia consumida/producida por una tensión aplicada a un condensador depende del producto de la tensión y su derivada:\[p(t) = C v(t) \frac{\text{d} v(t)}{\text{d}t}\]

Este resultado significa que el gasto total de energía de un condensador hasta el tiempo\(t\) viene dado de manera concisa por\[E(t) = \frac{1}{2} C v^{2} (t)\]

Esta expresión presume la suposición fundamental de la teoría de circuitos: todos los voltajes y corrientes en cualquier circuito eran cero en el pasado lejano\((t = -\infty)\).

Inductor

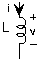
El inductor almacena flujo magnético, con inductores de mayor valor capaces de almacenar más flujo. La inductancia tiene unidades de henries\((\mathrm{H})\), y lleva el nombre del físico estadounidense Joseph Henry. Las formas diferencial e integral de la\(v \text{-} i\) relación del inductor son\[v(t) = L \frac{\text{d} i(t)}{\text{d}t}\]\[i(t) = \frac{1}{L} \int\limits_{-\infty}^{t} v (\alpha) \ \text{d} \alpha\] La potencia consumida/producida por un inductor depende del producto de la corriente del inductor y su derivada:\[p(t) = L i(t) \frac{\text{d} i(t)}{\text{d}t}\]

Su gasto energético total hasta el momento\(t\) viene dado por\[E(t) = \frac{1}{2} L i^{2} (t)\]

Fuentes

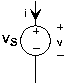a)

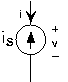b)

Las fuentes de voltaje y corriente también son elementos de circuito, pero no son lineales en el sentido estricto de los sistemas lineales. Por ejemplo, la\(v \text{-} i\) relación de la fuente de voltaje es\(v = v_{s}\) independientemente de cuál sea la corriente. En cuanto a la fuente de corriente,\(i = -i_{s}\) independientemente del voltaje. Otro nombre para una fuente de voltaje de valor constante es una batería, y se puede comprar en cualquier supermercado. Las fuentes actuales, por otro lado, son mucho más difíciles de adquirir; más adelante aprenderemos por qué.