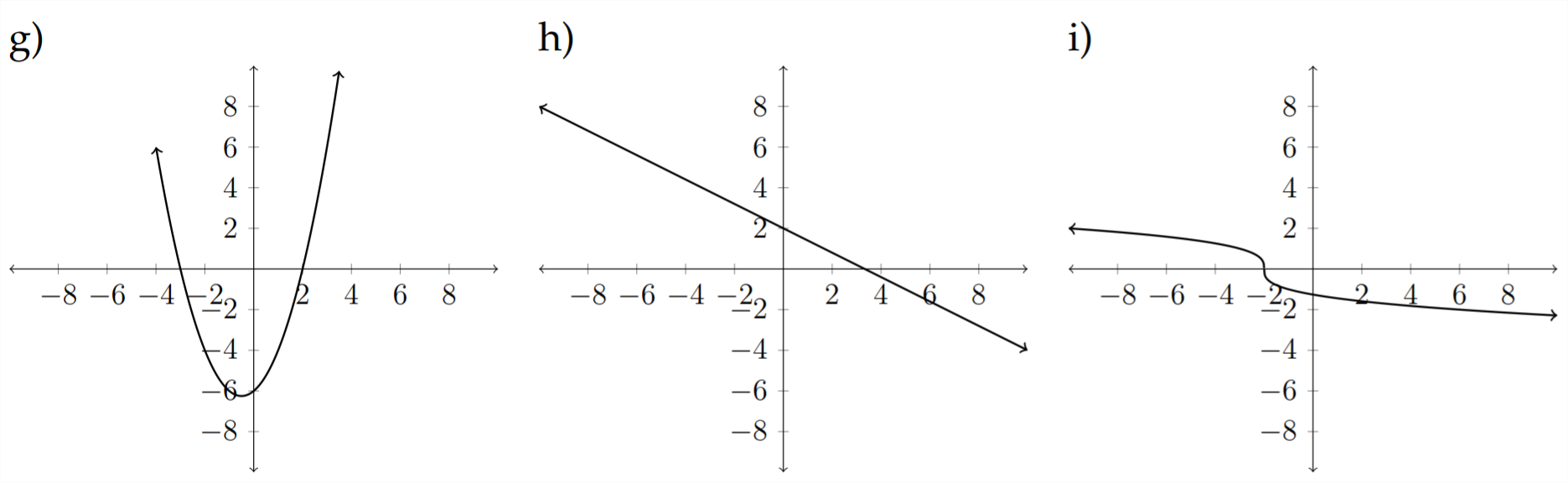4.5: Funciones de Toolbox

Aprender las formas generales de algunas familias de funciones comunes puede ser útil para analizar diversos problemas. Esto también puede ser útil para aplicar las ideas de regresión estadística. La regresión estadística suele recopilar una colección de puntos de datos e intenta ajustar una función matemática a los puntos de datos. Elegir el tipo de función que mejor se ajuste a los datos es un paso importante para determinar una función de regresión adecuada.
A continuación se muestra un ejemplo de una Regresión

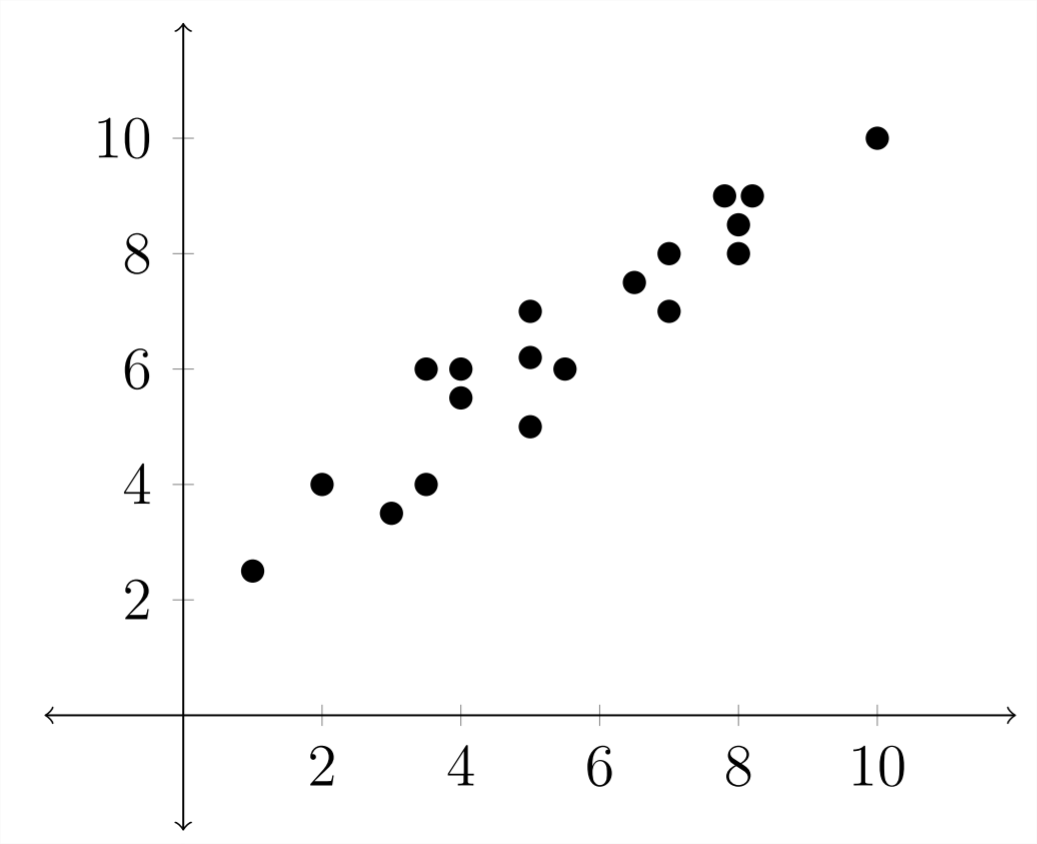
Lineal: El siguiente gráfico es un ejemplo de una Regresión Cuadrática:

Estar familiarizado con la forma típica de las diversas familias de funciones puede ayudar a analizar datos experimentales.
Las familias de funciones estándar son:




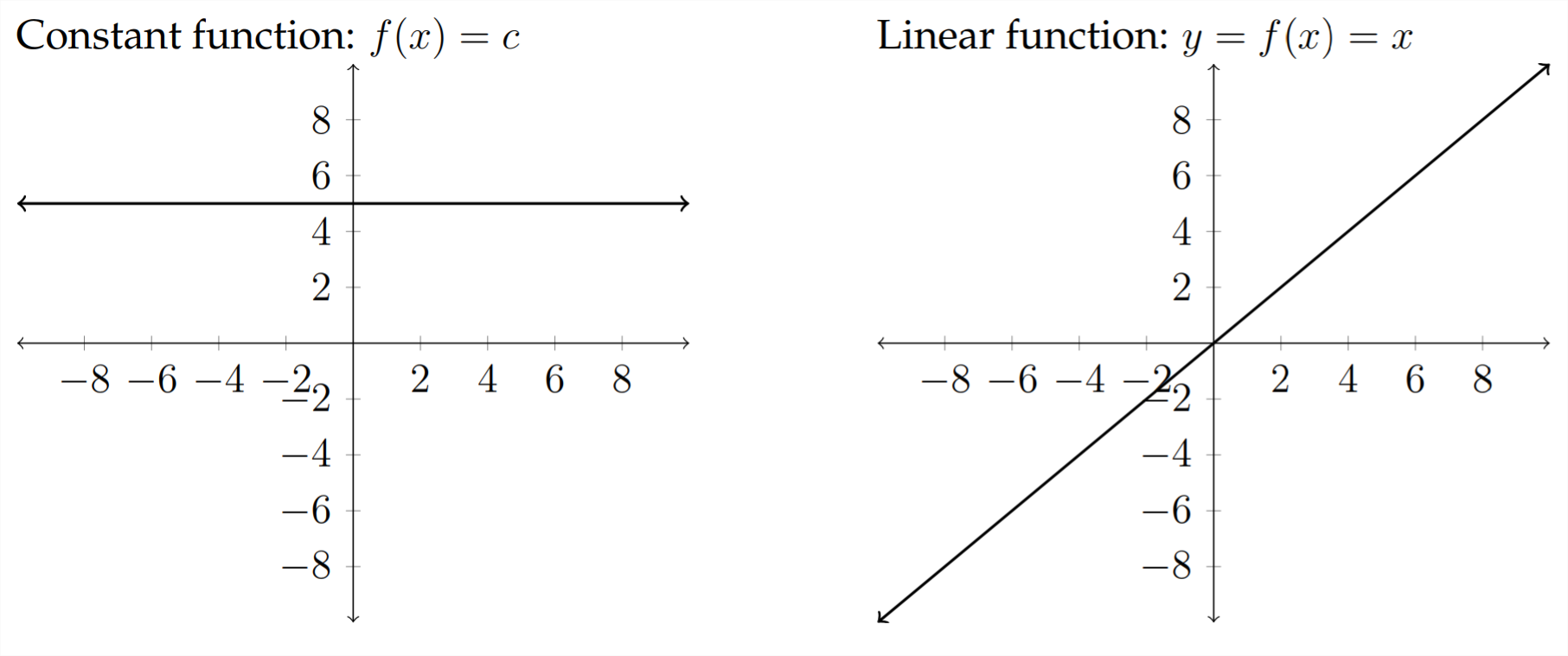
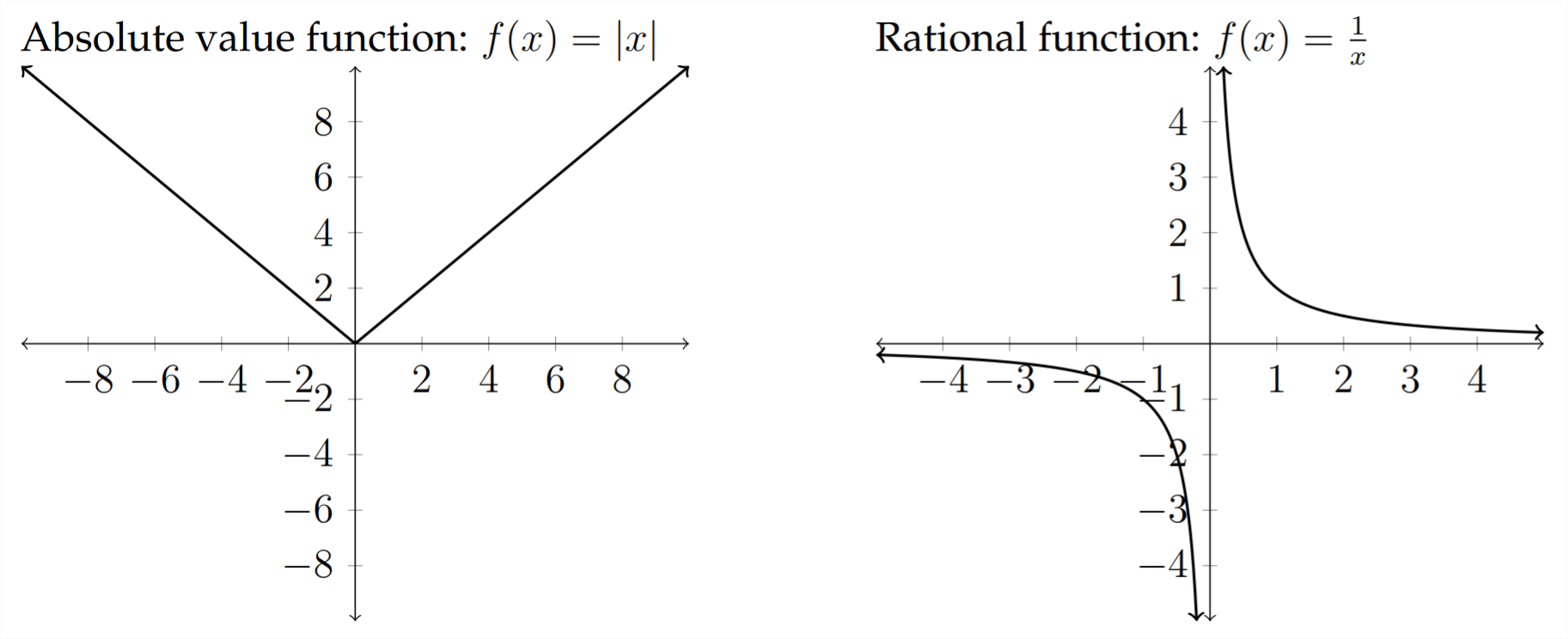
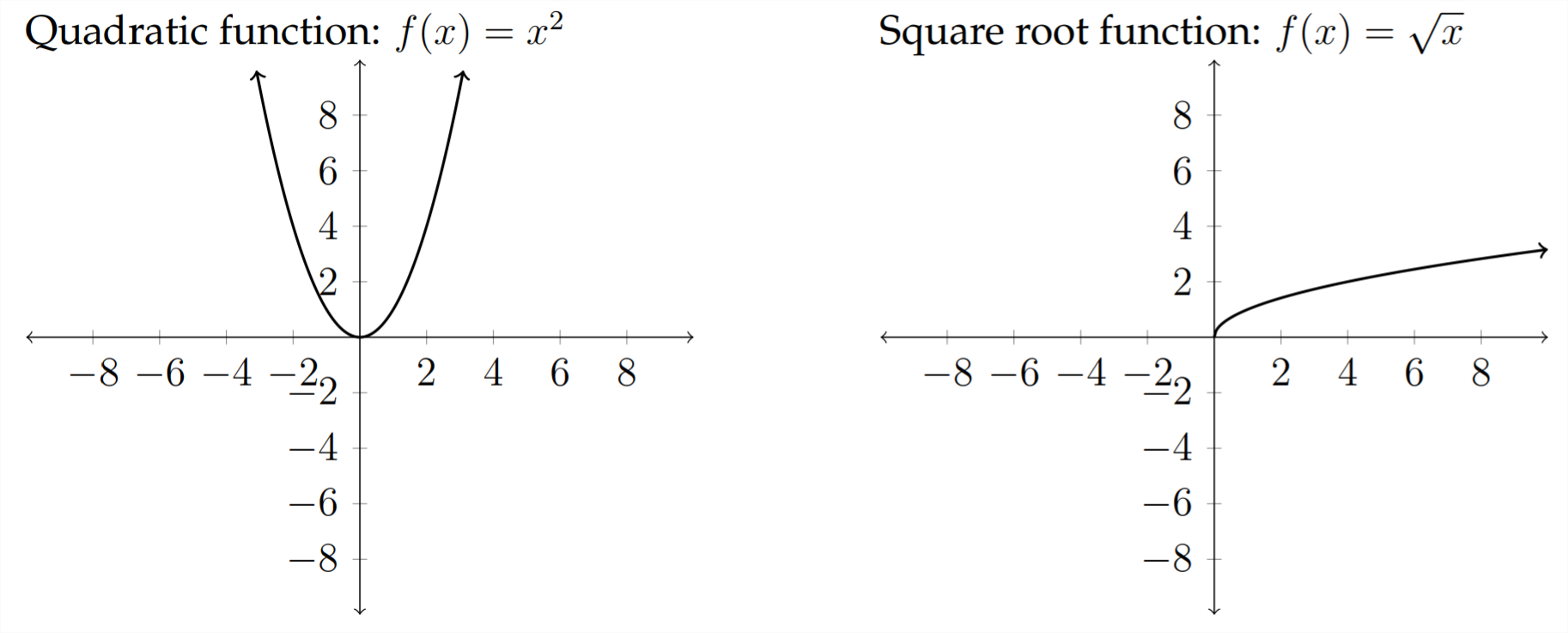
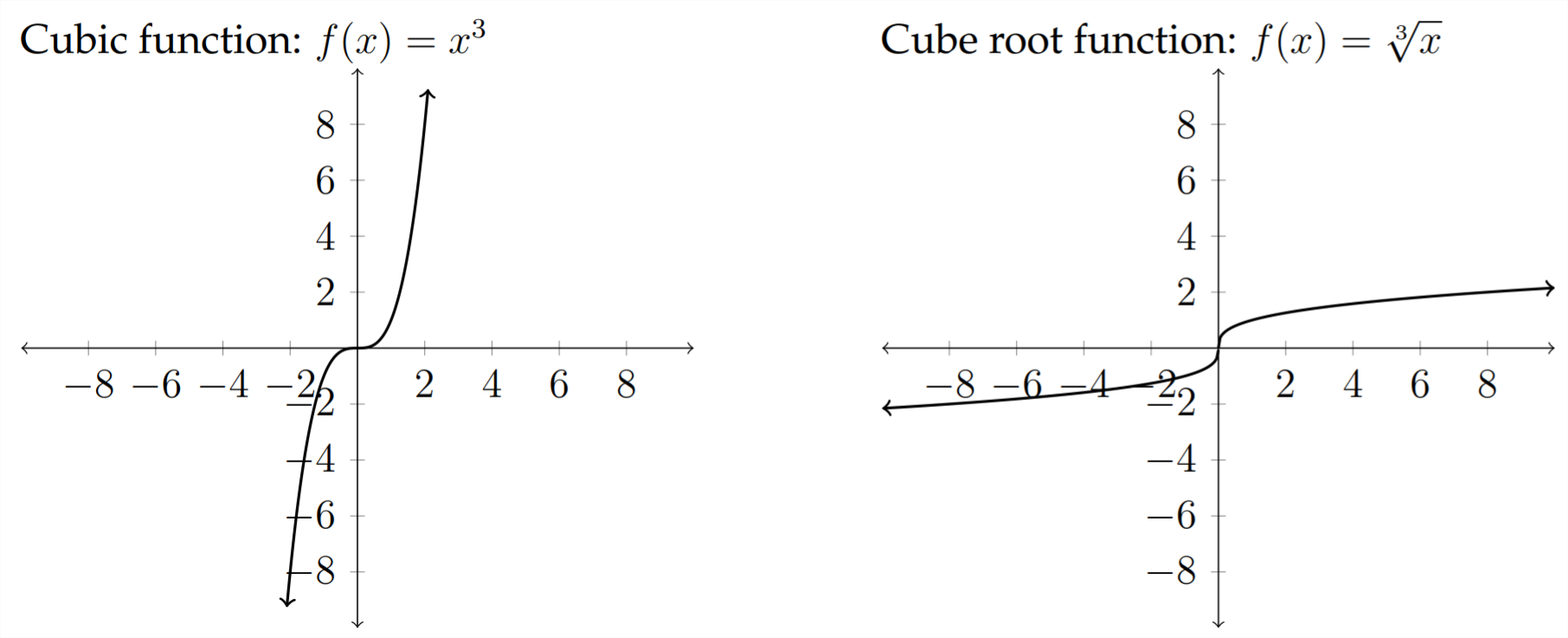
Algunas otras familias de funciones que no discutiremos son: Función

exponencial:$\quad f(x)=a^{x}$
Función logarítmica: Función$f(x)=\log _{b} x$
trigonométrica:$\quad f(x)=\sin x$

Ejercicios 4.5
Esbozar la gráfica para cada una de las siguientes transformaciones.
1)$\quad f(x)=x^{2}+3$
2)$\quad f(x)=x^{2}-4$
3)$\quad f(x)=(x-5)^{2}+3$
4)$\quad f(x)=(x+1)^{2}-4$
5)$\quad f(x)=|x|-2$
6)$\quad f(x)=|x|+5$
7)$\quad f(x)=|x+3|-2$
8)$\quad f(x)=|x-1|+5$

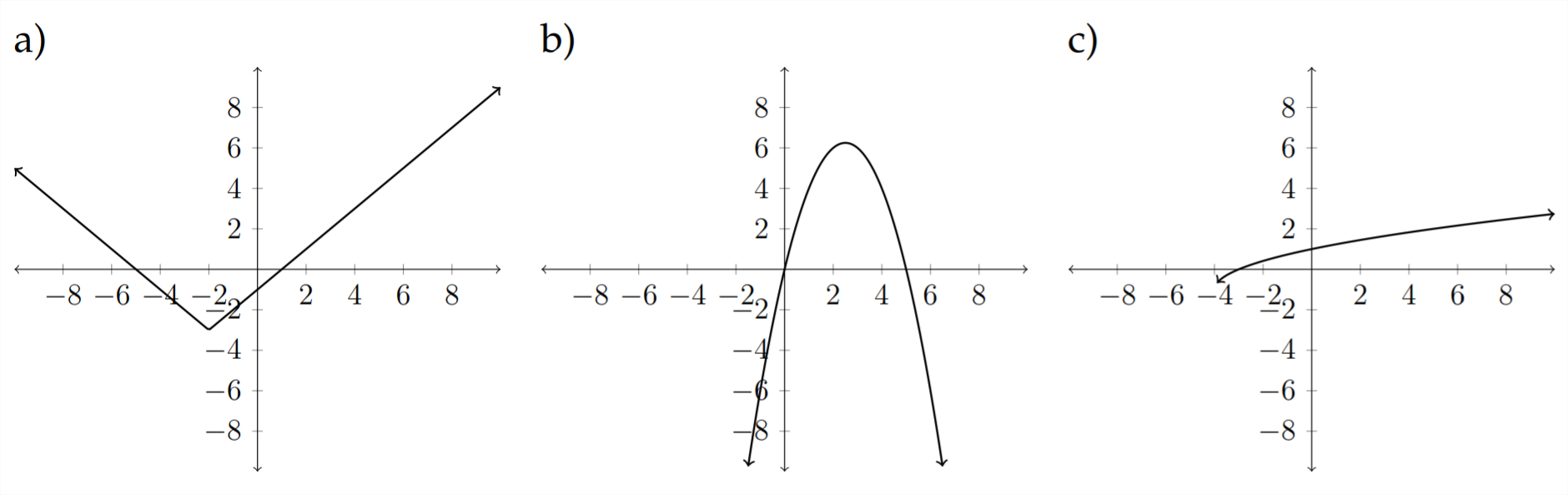
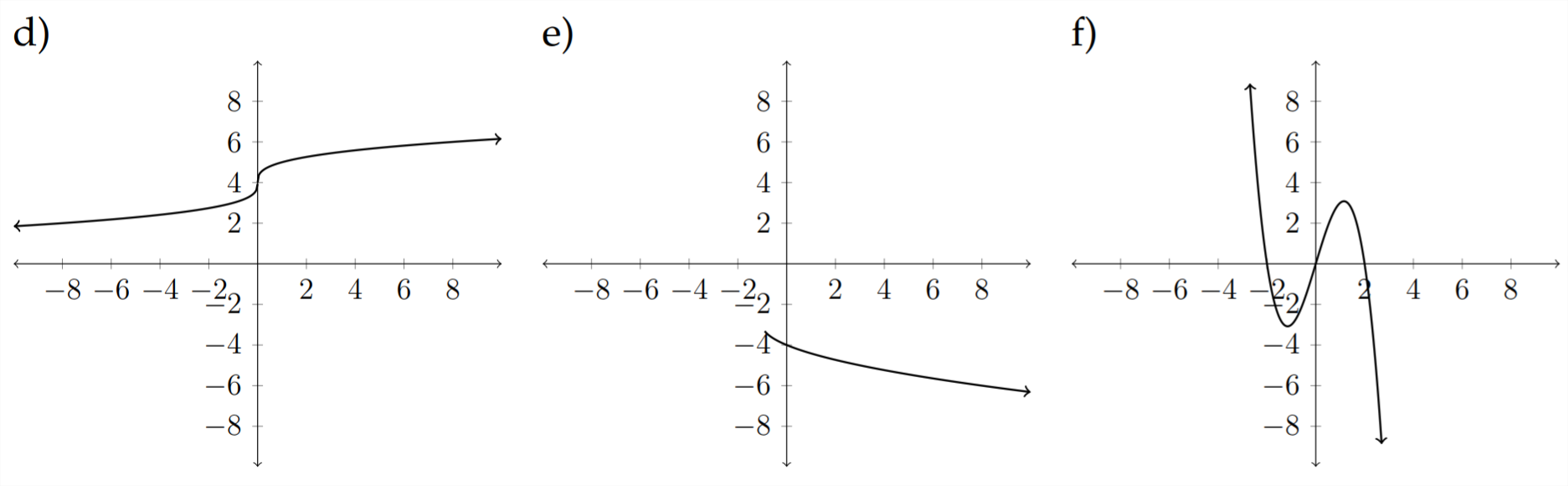
Haga coincidir cada una de las siguientes ecuaciones con la gráfica apropiada.
9)$\quad f(x)=\sqrt{x+4}-1$
10)$\quad h(x)=x^{2}+x-6$
11)$\quad g(x)=|x+2|-3$
12)$\quad f(x)=4 x-x^{2}$
13)$\quad h(x)=-|x-2|+1$
14)$\quad g(x)=-\frac{3}{5} x+2$
15)$\quad h(x)=\sqrt[3]{x}+4$
16)$\quad f(x)=x^{3}-3 x^{2}+3 x+2$
17)$\quad g(x)=-\sqrt[3]{x+2}$
18) $\quad h(x)=-\sqrt{x+1}-3$
19)$\quad f(x)=\frac{5}{4} x-2$
20)$\quad f(x)=4 x-x^{3}$