16.9: Suplemento de ejercicio
( \newcommand{\kernel}{\mathrm{null}\,}\)
Suplemento de ejercicio
Símbolos y Notaciones
Para los siguientes problemas, simplifique las expresiones.
12+7(4+3)
- Responder
-
61
9(4−2)+6(8+2)−3(1+4)
6[1+8(7+2)]
- Responder
-
438
26÷2−10
(4+17+1)+414−1
- Responder
-
2
51÷3÷7
(4+5)(4+6)−(4+7)
- Responder
-
79
8(2⋅12÷13)+2⋅5⋅11−[1+4(1+2)]
34+112(34−12)
- Responder
-
3747
48−3[1+176]
29+116−1
- Responder
-
8
8811+999+1549−2211
8⋅62+9⋅9310⋅45
- Responder
-
43
Para los siguientes problemas, escriba el símbolo de relación apropiado (=, <, >) en lugar de la ∗.
22∗6
9[4+3(8)]∗6[1+8(5)]
- Responder
-
252>246
3(1.06+2.11)∗4(11.01−9.06)
2∗0
- Responder
-
2>0
Para los siguientes problemas, indicar si las letras o símbolos son iguales o diferentes.
<y≱
>y\not <
- Responder
-
Diferente
a = byb = a
Representar la suma dec yd dos formas diferentes.
- Responder
-
c + d;d + c
Para los siguientes problemas, use anotación algebraica.
8más9
62dividido porf
- Responder
-
\dfrac{62}{f}o62 \div f
8tiempos(x + 4)
6vecesx, menos2
- Responder
-
6x - 2
x + 1dividido porx - 3
y + 11dividido pory + 10, menos12
- Responder
-
(y + 11) \div (y + 10) - 12o\dfrac{y + 11}{y + 10} - 12
cero menosa vecesb
La línea numérica real y los números reales
¿Cada número natural es un número entero?
- Responder
-
Sí
¿Cada número racional es un número real?
Para los siguientes problemas, ubique los números en una recta numérica colocando un punto en su posición (aproximada).
2
- Responder
-
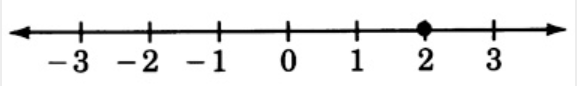
3.6
-1\dfrac{3}{8}
- Responder
-

0
-4\dfrac{1}{2}
- Responder
-
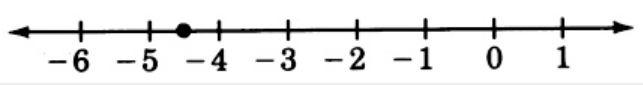
Dibuja una recta numérica que se extienda de 10 a 20. Coloque un punto en todos los enteros impares.
Dibuja una recta numérica que se extienda de−10 a10. Coloque un punto en todos los enteros impares negativos y en todos los enteros positivos pares.
- Responder
-
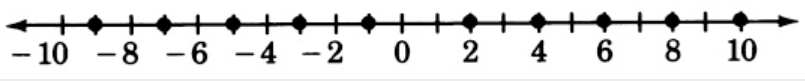
Dibuja una recta numérica que se extienda de−5 a10. Coloque un punto en todos los enteros que sean mayores entonces o iguales−2 pero estrictamente menores que5.
Dibuja una recta numérica que se extienda de−10 a10. Colocar un punto en todos los números reales que sean estrictamente mayores que−8 pero menores o iguales a7.
- Responder
-
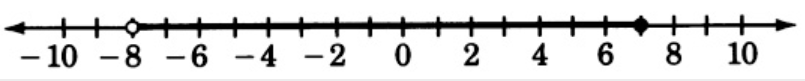
Dibuja una recta numérica que se extienda de−10 a10. Coloque un punto en todos los números reales entre e incluyendo−6 y4.
Para los siguientes problemas, escriba el símbolo de relación apropiado (=, <, >).
-30
- Responder
-
-3 < 0
-11
-8-5
- Responder
-
-8 < -5
-5-5\dfrac{1}{2}
¿Hay un número entero de dos dígitos más pequeño? Si es así, ¿qué es?
- Responder
-
Sí,-99
¿Hay un número real de dos dígitos más pequeño? Si es así, ¿qué es?
Para los siguientes problemas, ¿qué enteros pueden sustituir a x para que las declaraciones sean verdaderas?
4 \le x \le 7
- Responder
-
4, 5, 6o7
-3 \le x < 1
-30
- Responder
-
-3 < 0
-3 < x \le 2
- Responder
-
-2, -1, 0, 1, o2
La temperatura hoy en Los Ángeles era de ochenta y dos grados. Representar esta temperatura por número real.
La temperatura hoy en Marbelhead era de seis grados bajo cero. Representar esta temperatura por número real.
- Responder
-
-6°
En la recta numérica, ¿cuántas unidades entre-3 y2?
- Responder
-
-3 < 0
En la recta numérica, ¿cuántas unidades entre-4 y0?
- Responder
-
4
Propiedades de los números reales
a + b = b + aes una ilustración de la propiedad de adición.
st = tses una ilustración de la _________ propiedad de __________.
- Responder
-
conmutativo, multiplicación
Utilice las propiedades conmutativas de suma y multiplicación para escribir expresiones equivalentes para los siguientes problemas.
y + 12
a + 4b
- Responder
-
4b + a
6x
2(a-1)
- Responder
-
(a-1)2
(-8)(4)
(6)(-9)(-2)
- Responder
-
(-9)(6)(-2)o(-9)(-2)(6) o(6)(-2)(-9) o(-2)(-9)(6)
(x + y)(x - y)
△ \cdot ⋄
- Responder
-
⋄\cdot △
Simplifique los siguientes problemas utilizando la propiedad conmutativa de la multiplicación. No es necesario utilizar la propiedad distributiva.
8x3y
16ab2c
- Responder
-
32abc
4axyc4d4e
3(x+2)5(x−1)0(x+6)
- Responder
-
0
8b(a−6)9a(a−4)
Para los siguientes problemas, utilice la propiedad distributiva para expandir las expresiones.
3(a + 4)
- Responder
-
3a + 12
a(b + 3c)
2g(4h + 2k
- Responder
-
8gh+4gk
(8m+5n)6p
3y(2x+4z+5w)
- Responder
-
6xy+12yz+15wy
(a+2)(b+2c)
(x+y)(4a+3b)
- Responder
-
4ax+3bx+4ay+3by
10a_z(b_z + c)
Exponentes
Para los siguientes problemas, escriba las expresiones usando notación exponencial.
xa la quinta.
- Responder
-
x^5
y + 2en cubos.
(a+2b)al cuadrado menos(a+3b) al cuarto.
- Responder
-
(a + 2b)^2 - (a + 3b)^4
xcubos más2 veces(y−x) hasta el séptimo.
aaaaaaa
- Responder
-
a^7
2 \cdot 2 \cdot 2 \cdot 2
(−8)(−8)(−8)(−8)xxxyyyyy
- Responder
-
(-8)^4x^3y^5
(x-9)(x-9) + (3x + 1)(3x + 1)(3x + 1)
2zzyzyyy + 7zzyz(a - 6)^2(a-6)
- Responder
-
2y^4z^3 + 7yz^3(a-6)^3
Para los siguientes problemas, ampliar los términos para que no aparezcan exponentes.
x^3
3x^3
- Responder
-
3xxx
7^3x^2
(4b)^2
- Responder
-
4b \cdot 4b
(6a^2)^3(5c-4)^2
(x^3+7)^2(y^2-3)^3(z+10)
- Responder
-
(xxx+7)(xxx+7)(yy−3)(yy−3)(yy−3)(z+10)
Elija valores paraa yb para mostrar que:
a. no siemprea+b)^2 es igual aa^2 + b^2
b.(a+b)^2 Puede ser igual aa^2 + b^2
Elija el valorx para mostrar eso
a. no siempre(4x)^2 es igual a4x^2.
b.(4x)^2 Puede ser igual a4x^2
- Responder
-
(a) cualquier valor excepto cero
(b) solo cero
Reglas de exponentes - Las reglas de poder para exponentes
Simplifica los siguientes problemas.
4^2 + 8
6^3 + 5(30)
- Responder
-
366
1^8 + 0^{10} + 3^2(4^2 + 2^3)
12^2 + 0.3(11)^2
- Responder
-
180.3
\dfrac{3^4 + 1}{2^2 + 4^2 + 3^2}
\dfrac{6^2 + 3^2}{2^2 + 1} + \dfrac{(1+4)^2 - 2^3 - 1^4}{2^5-4^2}
- Responder
-
10
a^4a^3
2b^52b^3
- Responder
-
4b^8
4a^3b^2c^8 \cdot 3ab^2c^0
(6x^4y^{10})(xy^3)
- Responder
-
6x^5y^{13}
(3xyz^2)(2x^2y^3)(4x^2y^2z^4)
(3a)^4
- Responder
-
81a^4
(10xy)^2
(x^2y^4)^6
- Responder
-
x^{12}y^{24}
(a^4b^7c^7z^{12})^9
(\dfrac{3}{4}x^8y^6z^0a^{10}b^{15})^2
- Responder
-
\dfrac{9}{16}x^{16}y^{12}a^{20}b^{30}
\dfrac{14a^4b^6c^7}{2ab^3c^2}
- Responder
-
7a^3b^3c^5
\dfrac{11x^4}{11x^4}
x^4 \cdot \dfrac{x^{10}}{x^3}
- Responder
-
x^{11}
a^3b^7 \cdot \dfrac{a^9b^6}{a^5b^{10}}
\dfrac{(x^4y^6z^{10})^4}{(xy^5z^7)^3}
- Responder
-
x^{13}y^9z^{19}
\dfrac{(2x-1)^{13}(2x+5)^5}{(2x-1)^{10}(2x+5)}
(\dfrac{3x^2}{4y^3})^2
- Responder
-
\dfrac{9x^4}{16y^6}
\dfrac{(x+y)^9(x-y)^4}{(x+y)^3}
x^n \cdot x^m
- Responder
-
x^{n+m}
a^{n+2}a^{n+4}
6b^{2n+7} \cdot 8b^{5n+2}
- Responder
-
48b^{7n+9}
\dfrac{18x^{4n+9}}{2x^{2n+1}}
(x^{5t}y^{4r})^7
- Responder
-
x^{35t}y^{28r}
(a^{2n}b^{3m}c^{4p})^{6r}
\dfrac{u^w}{u^k}
- Responder
-
u^{w-k}


