26.7: A.7- Funciones especiales (valor absoluto, enésima raíz, etc.)

Última actualización
Guardar como PDF

Page ID: 117836

Thomas Tradler and Holly Carley
CUNY New York City College of Technology via New York City College of Technology at CUNY Academic Works

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $ $ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $$\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$ $\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$$\newcommand{\AA}{\unicode[.8,0]{x212B}}$

Ahora señalamos algunas funciones importantes en el menú MATH ($\boxed{\text{math}}$) y en el menú LIST ($\boxed{\text{2nd}}$$\boxed{\text{stat}}$), que se utilizan en este curso.

Fracciones

Supongamos que en la pantalla principal evalúas$4/6$. Su calculadora se mostrará$.66666666667$. Si quisieras ver esto como una fracción escribirías$\boxed{\text{math}}$$\boxed{\text{enter}}$$\boxed{\text{enter}}$. Ahora verá que$2/3$ se muestra.

Valor absoluto

Para evaluar$|-4|$ escribirías en la pantalla principal$\boxed{\text{math}}$$\boxed{\triangleright}$$\boxed{\text{enter}}$$\boxed{\text{(-)}}$$\boxed{\text{4}}$$\boxed{\text{enter}}$. Se$4$ debe mostrar la respuesta. Para graficar$|x-2|$, vaya a la pantalla Y= ($\boxed{y=}$) e ingrese en el espacio Y1 (por ejemplo)$\boxed{\text{math}}$$\boxed{\triangleright}$$\boxed{\text{enter}}$$\boxed{\text{X,T,}\theta,n}$$\boxed{\text{-}}$$\boxed{\text{2}}$$\boxed{\triangleright}$$\boxed{\text{enter}}$.

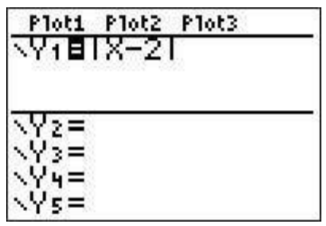
$clipboard_e9bde1ace956cd3e8402f7cebe8f58604.png$

Ahora puedes ir a la pantalla gráfica para ver la gráfica.

$n$-ésima raíz

Para calcular la raíz cubo de$8$. Presione en la pantalla principal$\boxed{\text{3}}$$\boxed{\text{math}}$$\boxed{\text{5}}$ (o seleccione la raíz x)$\boxed{\text{8}}$$\boxed{\text{enter}}$.

$clipboard_eba2991b42ed37fde034d5285d203bfd6.png$

Puede calcular cualquier raíz de esta manera (por ejemplo, la raíz$5$ th de$7$). Para la raíz cubo también se puede utilizar$\boxed{\text{math}}$$\boxed{\text{4}}$ en su lugar. Recordemos también la definición algebraica de la raíz$n$ th,

\[\sqrt[n]{x}=x^{\frac{1}{n}} \nonumber \]

para que la raíz$5$ th de también se$7$ pueda calcular presionando$\boxed{\text{7}}$$\boxed{\wedge}$$\boxed{\text{(}}$$\boxed{\text{1}}$$\boxed{\div}$$\boxed{\text{5}}$$\boxed{\text{)}}$.

Factoriales

Para calcular$5!$, por ejemplo, en la pantalla principal escriba$\boxed{\text{5}}$$\boxed{\text{math}}$ luego mover el cursor hacia la derecha tres veces (o una vez a la izquierda) para que se muestre el menú 'PRB'. La opción 4 es '$!$' así que presiona$\boxed{\text{4}}$ o mueve el cursor para resaltar esta opción y presiona$\boxed{\text{enter}}$. Finalmente presione$\boxed{\text{enter}}$ y$120$ se mostrará la respuesta.

$clipboard_e2e178d1289538f1b0de9557e24addc05.png$

Combinaciones y permutaciones

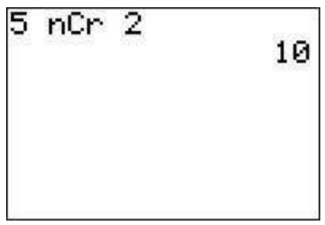
Para calcular$_{5} C_{2}=\dbinom{5}{2}$, en el tipo de menú principal$\boxed{\text{5}}$. Después vaya al menú de probabilidad matemática presionando$\boxed{\text{math}}$ luego la flecha derecha (o izquierda) hasta que se resalte 'PRB'. Verás que la tercera opción es$_nC_r$ tan presionada$\boxed{\text{3}}$. Esto te devolverá a la pantalla principal. Prensa$\boxed{\text{2}}$$\boxed{\text{enter}}$. Se$10$ mostrará la respuesta.

$clipboard_eb518cfa3d49bb14140f7232bdba2c448.png$

Las permutaciones$_nP_r$ se manejan de la misma manera excepto que es la segunda opción bajo el menú matemática-probabilidad en lugar de la tercera.

Secuencias y series

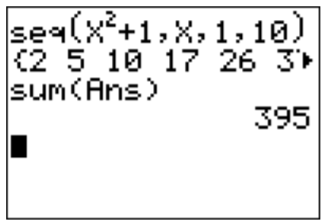
Una secuencia$a_1,a_2,a_3,\dots$ que viene dada por una fórmula se puede agregar a través del menú 'LISTA' (presione$\boxed{\text{2nd}}$$\boxed{\text{stat}}$). Por ejemplo, para ingresar los primeros$10$ términos$a_1,a_2,\dots, a_{10}$ de la secuencia$a_n=n^2+1$, tenemos que escribir$seq(x^2+1,x,1,10)$. Aquí, el comando 'sequence' toma cuatro entradas, primero la asignación$x^2+1$, segundo la variable independiente$x$, tercero el índice$1$ inicial y cuarto el índice final$10$. Esta expresión se ingresa a la calculadora presionando$\boxed{\text{2nd}}$$\boxed{\text{stat}}$$\boxed{\triangleright}$$\boxed{\text{5}}$$\boxed{\text{X,T,}\theta,n}$$\boxed{x^2}$$\boxed{\text{+}}$$\boxed{\text{1}}$$\boxed{\text{,}}$$\boxed{\text{X,T,}\theta,n}$$\boxed{\text{,}}$$\boxed{\text{1}}$$\boxed{\text{,}}$$\boxed{\text{1}}$$\boxed{\text{0}}$$\boxed{\text{)}}$ y luego se confirma presionando$\boxed{\text{enter}}$. Obtenemos:

$clipboard_e94ce16d279929b2c01e928753648dc23.png$

Para agregar los diez números$a_1+\dots +a_{10}$ de la secuencia, utilizamos el comando 'sum' en el menú LIST-MATH. Presione$\boxed{\text{2nd}}$$\boxed{\text{stat}}$$\boxed{\triangleleft}$$\boxed{\text{5}}$ para ingresar la expresión$sum($ ''. Luego usando la respuesta previamente ingresada presionando$\boxed{\text{2nd}}$$\boxed{\text{(-)}}$, y terminando con$\boxed{\text{)}}$$\boxed{\text{enter}}$, ahora calculamos la suma deseada.

$clipboard_e45e5e22522f43bdbbadd970b5ef60192.png$

Fracciones

Valor absoluto

\(n\)-ésima raíz

Factoriales

Combinaciones y permutaciones

Secuencias y series