1.10:45-45-90 Triángulos Rectos
- Page ID
- 107698
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Los tiempos de pierna\(\sqrt{2}\) son iguales a hipotenusa
45-45-90 Triángulos Recto
Un triángulo rectángulo con patas congruentes y ángulos agudos es un triángulo rectángulo isósceles. Este triángulo también se llama triángulo 45-45-90 (llamado así por las medidas del ángulo).
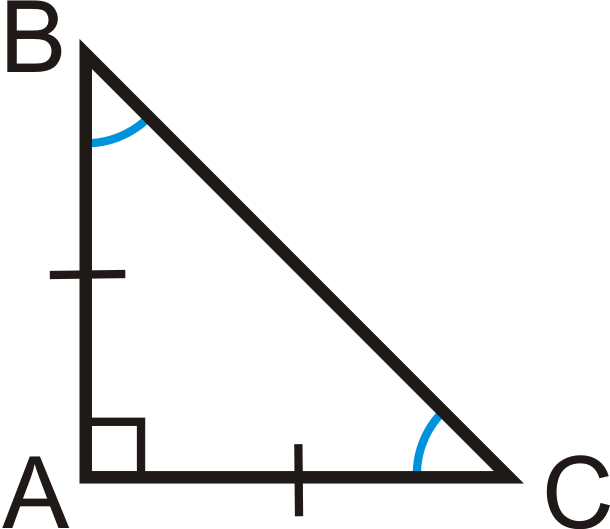
\(\Delta ABC\)es un triángulo rectángulo con\(m\angle A=90^{\circ}\),\(\overline{AB} \cong \overline{AC}\) y\(m\angle B=m\angle C=45^{\circ}\).
45-45-90 Teorema: Si un triángulo rectángulo es isósceles, entonces sus lados están en la proporción\(x:x:x\sqrt{2}\). Para cualquier triángulo rectángulo isósceles, las piernas son\(x\) y la hipotenusa es siempre\(x\sqrt{2}\).
¿Y si te dieran un triángulo rectángulo isósceles y la longitud de uno de sus lados? ¿Cómo pudiste averiguar las longitudes de sus otros lados?
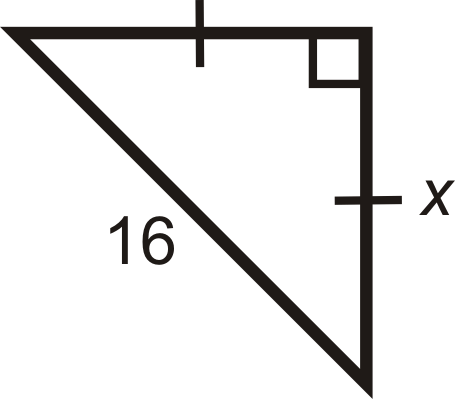
Encuentra la longitud de\(x\).
Solución
Usa la\(x:x:x\sqrt{2}\) relación.
Aquí, se nos da la hipotenusa. Resolver para\(x\) en la proporción.
\(\begin{aligned} x\sqrt{2} =16\\ x=16\sqrt{2}\cdot \dfrac{\sqrt{2}}{\sqrt{2}}=\dfrac{16\sqrt{2}}{2}=8\sqrt{2} \end{aligned}\)
Encuentra la longitud de\(x\), donde\(x\) esta la hipotenusa de un triángulo 45-45-90 con longitudes de pierna de\(5\sqrt{3}\).
Solución
Usa la\(x:x:x\sqrt{2}\) relación.
\(x=5\sqrt{3}\cdot\sqrt{2}=5\sqrt{6}\)
Encuentra la longitud del lado faltante.
Solución
Usa la\(x:x:x\sqrt{2}\) relación. \(TV=6\)porque es igual a\(ST\). Entonces,\(SV=6 \cdot \sqrt{2}=6\sqrt{2}\).
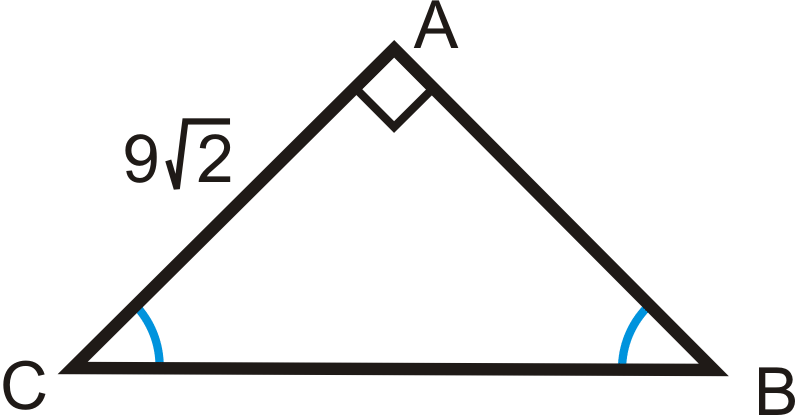
Encuentra la longitud del lado faltante.
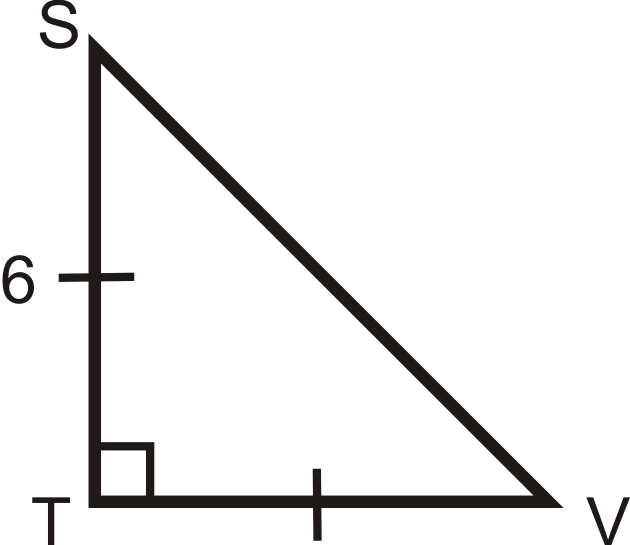
Solución
Usa la\(x:x:x\sqrt{2}\) relación. \(AB=9\sqrt{2}\)porque es igual a\(AC\). Entonces,\(BC=9\sqrt{2}\cdot\sqrt{2}=9\cdot 2=18\).
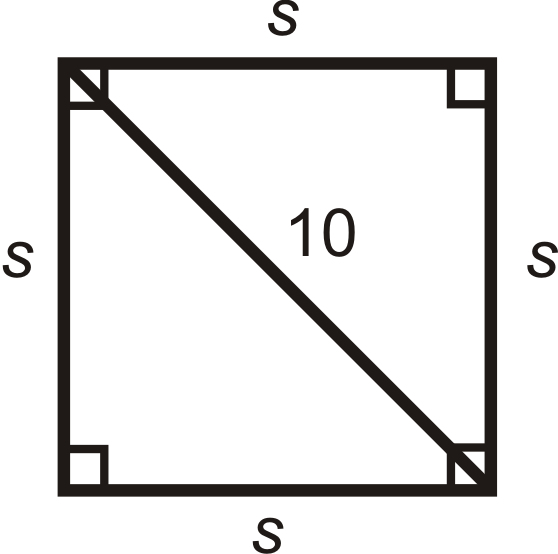
Un cuadrado tiene una diagonal con longitud 10, ¿cuáles son las longitudes de los lados?
Solución
Dibuja un cuadro.
Sabemos que la mitad de un cuadrado es un triángulo 45-45-90, entonces\(10=s\sqrt{2}\).
\(\begin{aligned} s\sqrt{2}&=10 \\ s&=10\sqrt{2}\cdot \dfrac{\sqrt{2}}{\sqrt{2}}=\dfrac{10\sqrt{2}}{2}=5\sqrt{2} \end{aligned}\)
Revisar
- En un triángulo rectángulo isósceles, si una pierna es 4, entonces la hipotenusa es __________.
- En un triángulo rectángulo isósceles, si una pata es x, entonces la hipotenusa es __________.
- Un cuadrado tiene lados de longitud 15. ¿Cuál es la longitud de la diagonal?
- La diagonal de un cuadrado es 22. ¿Cuál es la longitud de cada lado?
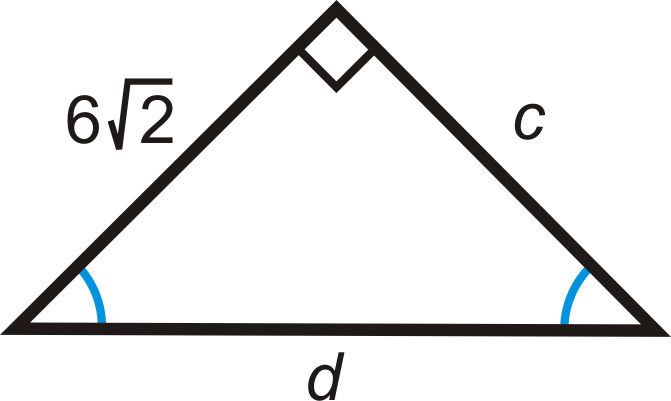
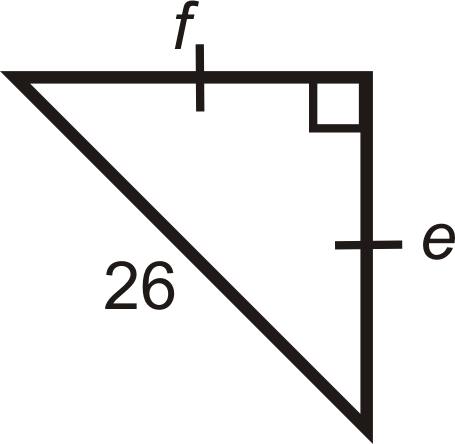
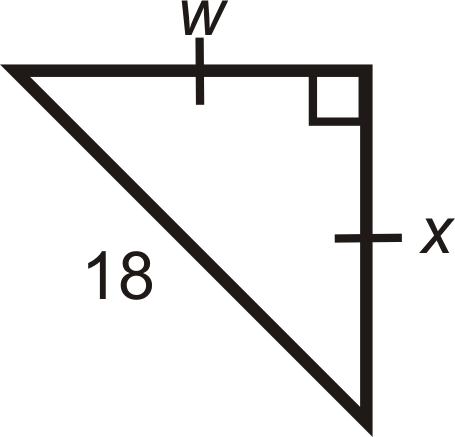
Para las preguntas 5-11, encuentra los largos de los lados faltantes. Simplifica todos los radicales.
-
Figura\(\PageIndex{5}\) -
Figura\(\PageIndex{6}\) -
Figura\(\PageIndex{7}\) -
Figura\(\PageIndex{8}\) -
Figura\(\PageIndex{9}\) -
Figura\(\PageIndex{10}\) -
Figura\(\PageIndex{11}\)
Reseña (Respuestas)
Para ver las respuestas de Revisar, abra este archivo PDF y busque la sección 8.5.
Recursos
vocabulario
| Término | Definición |
|---|---|
| Teorema 45-90 | Para cualquier triángulo rectángulo isósceles, si las patas son x unidades de largo, la hipotenusa es siempre\(x\sqrt{2}\). |
| 45-45-90 Triángulo | Un triángulo 45-45-90 es un triángulo rectángulo especial con ángulos de\(45^{\circ}\),\(45^{\circ}\), y\(90^{\circ}\). |
| Hipotenusa | La hipotenusa de un triángulo rectángulo es el lado más largo del triángulo rectángulo. Está frente al ángulo recto. |
| Patas de un Triángulo Recto | Las patas de un triángulo rectángulo son los dos lados más cortos del triángulo rectángulo. Las patas están adyacentes al ángulo recto. |
| Radical | El signo √, o raíz cuadrada. |
Recursos adicionales
Video: Resolviendo triángulos rectos especiales
Actividades: 45-45-90 Triángulos Rectos Preguntas de Discusión
Ayudas de estudio: Guía de estudio de triángulos rectos especiales
Práctica: 45-45-90 Triángulos Rectos
Mundo real: Combatiendo la guerra contra las drogas usando geometría y triángulos especiales