24.2: Péndulo Físico
- Page ID
- 125598
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Un péndulo físico consiste en un cuerpo rígido que se somete a rotación de eje fijo alrededor de un punto fijo\(S\) (Figura 24.2).
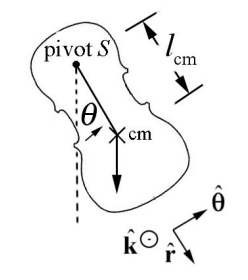
La fuerza gravitacional actúa en el centro de masa del péndulo físico. Denote la distancia del centro de masa al punto de pivote\(S\) por\(l_{\mathrm{cm}}\). El análisis de par es casi idéntico al péndulo simple. El par sobre el punto de pivote\(S\) viene dado por
\[\vec{\tau}_{S}=\overrightarrow{\mathbf{r}}_{S, \mathrm{cm}} \times m \overrightarrow{\mathrm{g}}=l_{\mathrm{cm}} \hat{\mathbf{r}} \times m g(\cos \theta \hat{\mathbf{r}}-\sin \theta \hat{\boldsymbol{\theta}})=-l_{\mathrm{cm}} m g \sin \theta \hat{\mathbf{k}} \nonumber \]
Siguiendo los mismos pasos que llevaron de la Ecuación (24.1.1) a la Ecuación (24.1.4), la ecuación rotacional para el péndulo físico es
\[-m g l_{\mathrm{cm}} \sin \theta=I_{S} \frac{d^{2} \theta}{d t^{2}} \nonumber \]
donde\(I_{s}\) el momento de inercia sobre el punto de pivote\(S\). Al igual que con el péndulo simple, para ángulos pequeños\(\sin \theta \approx \theta\), la ecuación (24.2.2) se reduce a la ecuación del oscilador armónico simple
\[\frac{d^{2} \theta}{d t^{2}} \simeq-\frac{m g l_{\mathrm{cm}}}{I_{S}} \theta \nonumber \]
La ecuación para el ángulo\(\theta(t)\) viene dada por
\[\theta(t)=A \cos \left(\omega_{0} t\right)+B \sin \left(\omega_{0} t\right) \nonumber \]
donde la frecuencia angular viene dada por
\[\omega_{0} \simeq \sqrt{\frac{m g l_{\mathrm{cm}}}{I_{S}}} \quad(\text { physical pendulum }) \nonumber \]
y el periodo es
\[T=\frac{2 \pi}{\omega_{0}} \simeq 2 \pi \sqrt{\frac{I_{S}}{m g l_{\mathrm{cm}}}} \quad(\text { physical pendulum }) \nonumber \]
Sustituir el teorema del eje paralelo,\(I_{S}=m l_{\mathrm{cm}}^{2}+I_{\mathrm{cm}}\) en la Ecuación (24.2.6) con el resultado de que
\[T \simeq 2 \pi \sqrt{\frac{l_{\mathrm{cm}}}{g}+\frac{I_{\mathrm{cm}}}{m g l_{\mathrm{cm}}}} \quad(\text { physical pendulum }) \nonumber \]
Así, si el objeto es “pequeño” en el sentido de que\(I_{\mathrm{cm}}<<m l_{\mathrm{c}}^{2}\), las expresiones para el péndulo físico se reducen a las del péndulo simple. El componente z de la velocidad angular viene dado por
\[\omega_{z}(t)=\frac{d \theta}{d t}(t)=-\omega_{0} A \sin \left(\omega_{0} t\right)+\omega_{0} B \cos \left(\omega_{0} t\right) \nonumber \]
Los coeficientes A y B se pueden determinar a partir de las condiciones iniciales estableciendo t = 0 en las Ecuaciones (24.2.4) y (24.2.8) dando como resultado las condiciones que
\ [\ begin {array} {l}
A=\ theta (t=0)\ equiv\ theta_ {0}\\
B=\ frac {\ omega_ {z} (t=0)} {\ omega_ {0}}\ equiv\ frac {\ omega_ {z, 0}} {\ omega_ {0}}
\ end {nonarray}\ umber\]
Por lo tanto, las ecuaciones para el ángulo\(\theta(t) \text { and } \omega_{z}(t)=\frac{d \theta}{d t}(t)\) vienen dadas por
\ [\ begin {array} {c}
\ theta (t) =\ theta_ {0}\ cos\ izquierda (\ omega_ {0} t\ derecha) +\ frac {\ omega_ {z, 0}} {\ omega_ {0}}\ sin\ izquierda (\ omega_ {0} t\ derecha)\
\ omega_ {z} (t)\ frac {d\ theta} {d t} (t) =-\ omega_ {0}\ theta_ {0}\ sin\ izquierda (\ omega_ {0} t\ derecha) +\ omega_ {z, 0}\ cos\ izquierda (\ omega_ {0} t\ derecha)
\ end {array}\ nonumber\]


