19.4: Variaciones
- Page ID
- 131510
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)En las Secciones 19.1,2,3, imaginamos que el cicloide fue generado por un círculo que rodaba en sentido antihorario a lo largo de la línea\(y = 2a\). También podemos imaginar variaciones como el círculo rodando en el sentido de las agujas del reloj\(y = 0\), o podemos comenzar con P en la parte superior del círculo en lugar de en la parte inferior. Resumo en esta sección cuatro variaciones. La distinción entre\( \psi \) y\( \theta \) es la siguiente. El ángulo que hace la tangente al cicloide con el\(x\) eje dirigido de manera positiva es\( \psi \); es decir,\( dx/dy = tan \psi \) .El círculo rueda a través de un ángulo\(2 \theta \). Existe una relación simple entre\( \psi \) y\( \theta \), que es diferente para cada caso.
En cada figura,\(x\) y\(y\) se trazan en unidades de\(a\). La altura vertical entre vértices y cúspides es\(2a\), la distancia horizontal entre una cúspide y el siguiente vértice es\( \pi a\), y la longitud del arco entre una cúspide y el siguiente vértice es\(4a\).
I. Círculo rueda en sentido antihorario a lo largo\(y = 2a\). P comienza en la parte inferior. Las cúspides están arriba. Un vértice está en el origen.
\[\begin{align*} x &= a(2 \theta + \sin 2 \theta ) \\[4pt] y &= 2 a \sin^2 \theta \label{19.4.2}\tag{19.4.2} \\[4pt] s &= 4a \sin \theta \label{19.4.3}\tag{19.4.3} \\[4pt] ^2 &= 8 ay \label{19.4.4}\tag{19.4.4} \\[4pt] \psi &= \theta . \label{19.4.5}\tag{19.4.5} \end{align*} \]
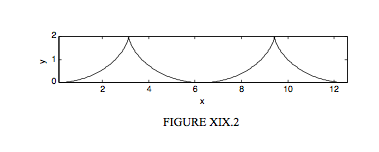
II. Círculo rueda hacia la derecha a lo largo\(y = 0\). P comienza en la parte inferior. Las cúspides están abajo. Una cúspide está en el origen.
\[ x = a(2\theta - sin 2\theta ) \label{19.4.6}\tag{19.4.6} \]
\[ y = 2a sin^2 \theta \label{19.4.7}\tag{19.4.7} \]
\[ s = 4a(1-cos \theta) \label{19.4.8}\tag{19.4.8} \]
\[ s^2 = 8a(y-s) \label{19.4.9}\tag{19.4.9} \]
\[ \psi = 90 \circ - \theta. \label{19.4.10}\tag{19.4.10} \]

III. Círculo rueda hacia la derecha a lo largo\(y = 0\). P comienza en la parte superior. Las cúspides están abajo. Un vértice está en\(x = 0\).
\[ x = a( 2 \theta + \sin 2 \theta \label{19.4.11}\tag{19.4.11} \]
\[ y = 2 a \cos^2 \theta \label{19.4.12}\tag{19.4.12} \]
\[ s = 4 a \sin \theta \label{19.4.13}\tag{19.4.13} \]
\[ s^2 = 8a(2a-y) \label{19.4.14}\tag{19.4.14} \]
\[ \psi = 180 \circ - \theta . \label{19.4.15}\tag{19.4.15} \]
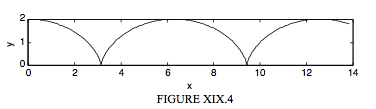
IV. Círculo rueda en sentido antihorario a lo largo\(y = 2a\). P comienza en la parte superior. Las cúspides están arriba. Una cúspide está en\(x = 0\).
\[ x = a (2 \theta - \sin 2 \theta) \label{19.4.16}\tag{19.4.16} \]
\[ y = 2a \cos^2 \theta \label{19.4.17}\tag{19.4.17} \]
\[ s = 4a (1-\cos \theta) \label{19.4.18}\tag{19.4.18} \]
\[ s^2 - 8as + 8a(2a - y) = 0 \label{19.4.19}\tag{19.4.19} \]
\[ \psi = 90 \circ + \theta \label{19.4.20}\tag{19.4.20} \]



