2.8: Recuento de Borda
- Page ID
- 110535
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
Borda Count es otro método de votación, llamado así por Jean-Charles de Borda, quien desarrolló el sistema en 1770.
En este método, los puntos se asignan a los candidatos en función de su clasificación; 1 punto por última opción, 2 puntos para la penúltima elección, y así sucesivamente. Se suman los valores de puntos para todas las boletas, y el candidato con el mayor total de puntos es el ganador.
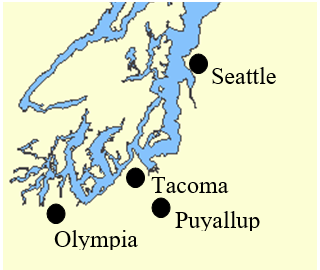Un grupo de matemáticos se están reuniendo para una conferencia. Los integrantes provienen de cuatro ciudades: Seattle, Tacoma, Puyallup y Olympia. Sus ubicaciones aproximadas en un mapa se muestran a la derecha.
Los votos para dónde celebrar la conferencia fueron:
\ (\ begin {array} {|l|l|l|l|l|}
\ hline & 51 & 25 & 10 & 14\\
\ hline 1^ {\ texto {st}}\ texto {elección} &\ texto {Seattle} &\ texto {Tacoma} &\ texto {Puyallup} &\ texto {Olympia}\
\ hline 2^ {\ texto {nd}}\ text {choice} &\ text { Tacoma} &\ texto {Puyallup} &\ texto {Tacoma} &\ texto {Tacoma}\
\ hline 3^ {\ texto {rd}}\ texto {elección} &\ texto {Olimpia} &\ texto {Olimpia} &\ texto {Olimpia} &\ texto {Puyallup}\
\ hline 4^ {\ texto {th}}\ texto {elección} &\ texto { Puyallup} &\ text {Seattle} &\ text {Seattle} &\ text {Seattle}\
\ hline
\ end {array}\)
Solución
En cada una de las 51 boletas que clasifican primero a Seattle, Puyallup recibirá 1 punto, Olympia 2 puntos, Tacoma 3 puntos y Seattle 4 puntos. Multiplicar los puntos por voto por el número de votos nos permite calcular los puntos otorgados:
\ (\ begin {array} {|l|l|l|l|l|}
\ hline & 51 & 25 & 10 & 14\\
\ hline 1^ {\ text {st choice}} &\ text {Seattle} &\ text {Tacoma} &\ text {Puyallup} &\ text {Olympia}\\
4\ text {puntos} & 4\ cdot 51=204 y 4\ cdot 25= 100 & amp; 4\ cdot 10=40 & 4\ cdot 14=56\
\ hline 2^ {\ text {nd choice}} &\ text {Tacoma} &\ text {Puyallup} &\ text {Tacoma} &\ text {Tacoma}\\
3\ texto {puntos} & 3\ cdot 51=153 & 3\ cdot 25=75 & 3\ cdot 10=30 & 3\ cdot 14=42\\
\ hline 3^ {\ texto {rd}}\ texto {elección} &\ texto {Olimpia} &\ texto {Olimpia} &\ texto {Olimpia} &\ texto {Puyallup}\\
2\ texto {puntos} & 2\ cdot 51=102 & 2\ cdot 25=50 & 2\ cdot 10=20 & 2\ cdot 14=28\
\ hline 4^ {\ texto {th}}\ texto { elección} &\ texto {Puyallup} &\ texto {Seattle} &\ texto {Seattle} &\ texto {Seattle}\\
1\ texto {punto} & 1\ cdot 51=51 & 1\ cdot 25=25 & 1\ cdot 10=10 & 1\ cdot 14=14\
\ hline
\ end {array}\)
Sumando los puntos:
- Seattle:\(204 + 25 + 10 + 14 = 253\) puntos
- Tacoma:\(153 + 100 + 30 + 42 = 325\) puntos
- Puyallup:\(51 + 75 + 40 + 28 = 194\) puntos
- Olympia:\(102 + 50 + 20 + 56 = 228\) puntos
Bajo el método Borda Count, Tacoma es el ganador de esta votación.
Considera nuevamente la elección de Pruébalo Ahora 1. Encuentra al ganador usando Borda Count. Ya que tenemos algunas boletas de preferencia incompletas, por simplicidad, darle a cada candidato no clasificado 1 punto, los puntos que normalmente obtendrían para el último lugar.
\ (\ begin {array} {|l|l|l|l|l|l|l|l|l|}
\ hline & 44 & 14 & 20 & 70 & 22 & 80 & 39\
\\ hline 1^ {\ text {st}}\ text {choice} &\ mathrm {G} &\ mathrm {G} &\ mathrm {G} &\ mathrm {M} &\ mathrm {M} &\ mathrm {B} &\ mathrm {B}\\
\ hline 2^ {\ text {nd}}\ text {choice} &\ mathrm {M} &\ mathrm {B} & &\ mathrm {G} &\ mathrm {B} &\ mathrm {M} &\\ hline 3^ {\ text {rd}}
\ text {choice} &\ mathrm {B} &\ mathrm {M} &\ mathrm {B} &\ mathrm {B} &\ mathrm {B} & &\ mathrm {B}} &\ mathrm {G} &\ mathrm {G} &\\
\ hline
\ end {array}\)
- Contestar
-
Uso de Borda Count:
Damos 1 punto por el 3er lugar, 2 puntos por el 2do lugar, y 3 puntos por el 1er lugar.
\ (\ begin {array} {|l|l|l|l|l|l|l|l|l|}
\ hline & 44 & 14 & 20 & 70 & 22 & 80 & 39\
\\ hline 1^ {\ text {st}}\ text {choice} &\ mathrm {G} &\ mathrm {G} &\ mathrm {G} &\ mathrm {M} &\ mathrm {M} &\ mathrm {B} &\ mathrm {B}\\
& 132\ mathrm {pt} & 42\ mathrm {pt} & 60\ mathrm {pt} & 210\ mathrm {pt} & 66\ mathrm {pt} & 240\ mathrm {pt} & 117\ mathrm {pt}\
\ hline 2^ {\ text {nd}}\ text {elección} &\ mathrm {M} &\ mathrm {B} &\ mathrm {G} &\ mathrm {B} &\ mathrm {M} &\\
& 88\ mathrm {pt} & 28\ mathrm {pt} & & 140\ mathrm {pt} & 44\ mathrm {pt} & 160\ mathrm {pt} &\
\ hline 3^ {\ text {rd}}\ text {choice} &\ mathrm {B} &\ mathrm {M} &\ mathrm {M} 20\ mathrm {pt} &\ mathrm {B} &\ mathrm {G} &\ mathrm {G} &\ mathrm {M} 39\ mathrm {pt}\\
& 44\ mathrm {pt} & 14\ mathrm {pt} &\ mathrm {B} 20\ mathrm {pt} & 70\ mathrm {pt} & 22\ mathrm {pt} & 80\ mathrm {pt} &\ mathrm {G} 39\ mathrm {pt}\\
\ hline
\ end {array}\)G:\(132+42+60+140+22+80+39 = 515\) pts
M:\(88+14+20+210+66+160+39 = 597\) pts
B:\(44+28+20+70+44+240+117 = 563\) puntos
McCarthy (M) sería el ganador usando Borda Count.