7.2: Círculos
( \newcommand{\kernel}{\mathrm{null}\,}\)
Recordemos de Geometría que un círculo se puede determinar fijando un punto (llamado centro) y un número positivo (llamado radio) de la siguiente manera.
Un círculo con centro(h,k) y radior>0 es el conjunto de todos(x,y) los puntos del plano cuya distancia a(h,k) esr.
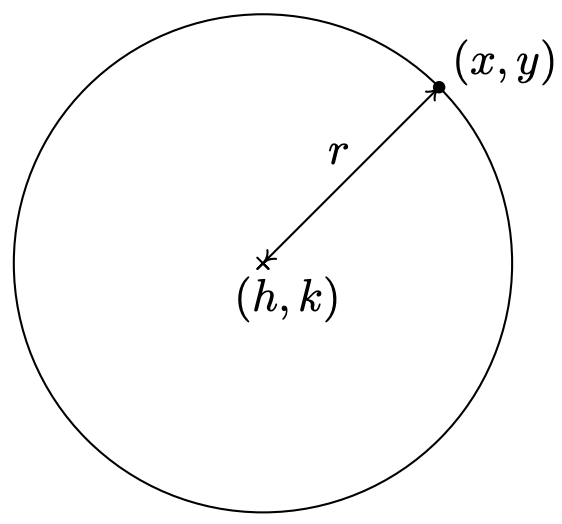
De la imagen, vemos que un punto(x,y) está en el círculo si y sólo si su distancia a(h,k) esr. Expresamos esta relación algebraicamente usando la Fórmula de Distancia, Ecuación 1.1, comor=√(x−h)2+(y−k)2
La ecuación estándar de un círculo: La ecuación de un círculo con centro(h,k) y radior>0 es(x−h)2+(y−k)2=r2.
Escribe la ecuación estándar del círculo con centro(−2,3) y radio5.
Solución
Aquí,(h,k)=(−2,3) yr=5, así conseguimos
(x−(−2))2+(y−3)2=(5)2(x+2)2+(y−3)2=25
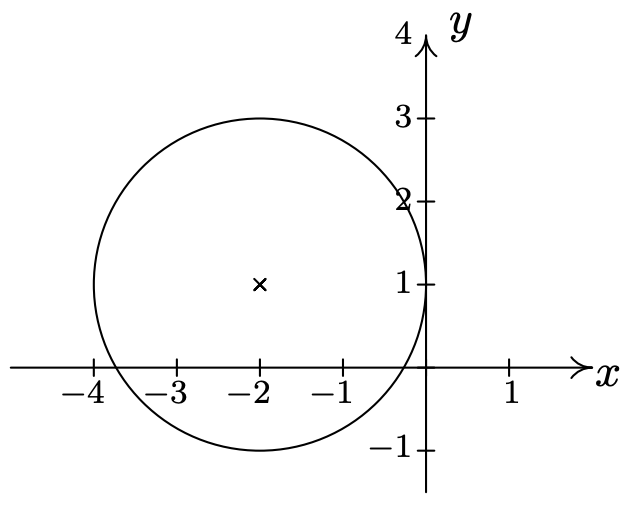
Gráfica(x+2)2+(y−1)2=4. Encuentra el centro y el radio.
Solución
De la forma estándar de un círculo, la Ecuación 7.1, tenemos quex+2 esx−h, asíh=−2 yy−1 esy−k asík=1. Esto nos dice que nuestro centro es(−2,1). Además,r2=4, entoncesr=2. Así tenemos un círculo centrado en(−2,1) con un radio de2. Graficar nos da
Si tuviéramos que expandir la ecuación en el ejemplo anterior y reunir como términos, en lugar de los fácilmente reconocibles(x+2)2+(y−1)2=4, estaríamos contendiendo conx2+4x+y2−2y+1=0. Si nos dan tal ecuación, podemos completar el cuadrado en cada una de las variables para ver si se ajusta a la forma dada en la Ecuación 7.1 siguiendo los pasos que se indican a continuación.
Para escribir la ecuación de un círculo en forma estándar
- Agrupe las mismas variables en un lado de la ecuación y posicione la constante en el otro lado.
- Completar el cuadrado en ambas variables según sea necesario.
- Divide ambos lados por el coeficiente de los cuadrados. (Para los círculos, serán los mismos.)
Completa el cuadrado para encontrar el centro y el radio de3x2−6x+3y2+4y−4=0.
Solución.
3x2−6x+3y2+4y−4=03x2−6x+3y2+4y=4add 4 to both sides3(x2−2x)+3(y2+43y)=4factor out leading coefficients[10pt]3(x2−2x+1_)+3(y2+43y+49__)=4+3(1)_+3(49)__complete the square in x, y[10pt]3(x−1)2+3(y+23)2=253factor[10pt](x−1)2+(y+23)2=259divide both sides by 3
A partir de la Ecuación 7.1, identificamosx−1 comox−hh=1, así, yy+23 comoy−k, asík=−23. De ahí que el centro sea(h,k)=(1,−23). Además, vemos quer2=259 así es el radior=53.
Es posible obtener ecuaciones como(x−3)2+(y+1)2=0 o(x−3)2+(y+1)2=−1, ninguna de las cuales describe un círculo. (¿Ves por qué no?) Se anima al lector a pensar qué puntos, en su caso, se encuentran en las gráficas de estas dos ecuaciones. El siguiente ejemplo utiliza la Fórmula de Punto Medio, Ecuación 1.2, en conjunto con las ideas presentadas hasta el momento en esta sección.
Escribe la ecuación estándar del círculo que tiene(−1,3) y(2,4) como los puntos finales de un diámetro.
Recordamos que un diámetro de un círculo es un segmento de línea que contiene el centro y dos puntos en el círculo. Trazar los rendimientos de datos dados
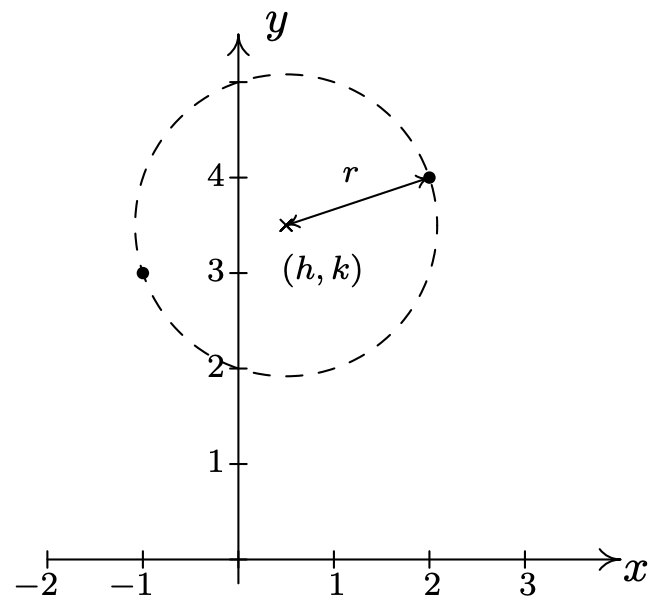
Dado que los puntos dados son puntos finales de un diámetro, sabemos que su punto medio(h,k) es el centro del círculo. La ecuación 1.2 nos da
(h,k)=(x1+x22,y1+y22)[8pt]=(−1+22,3+42)[8pt]=(12,72)
El diámetro del círculo es la distancia entre los puntos dados, por lo que sabemos que la mitad de la distancia es el radio. Por lo tanto,
r=12√(x2−x1)2+(y2−y1)2[8pt]=12√(2−(−1))2+(4−3)2[8pt]=12√32+12[8pt]=√102
Por último, ya que(√102)2=104, nuestra respuesta se convierte en(x−12)2+(y−72)2=104
Cerramos esta sección con el círculo 1 más importante en todas las matemáticas: el Círculo de Unidades.
El Círculo de Unidad es el círculo centrado en(0,0) con un radio de1. La ecuación estándar del Círculo Unitario esx2+y2=1.
Encuentra los puntos en el círculo unitario cony coordenada√32.
Solución
Reemplazamosy con√32 en la ecuaciónx2+y2=1 para obtener
x2+y2=1x2+(√32)2=1[13pt]34+x2=1x2=14x=±√14[7pt]x=±12
Nuestras respuestas finales son(12,√32) y(−12,√32).
7.2.1. Ejercicios
En los Ejercicios 1 - 6, encuentra la ecuación estándar del círculo y luego graficarla.
- Centro(−1,−5), radio10
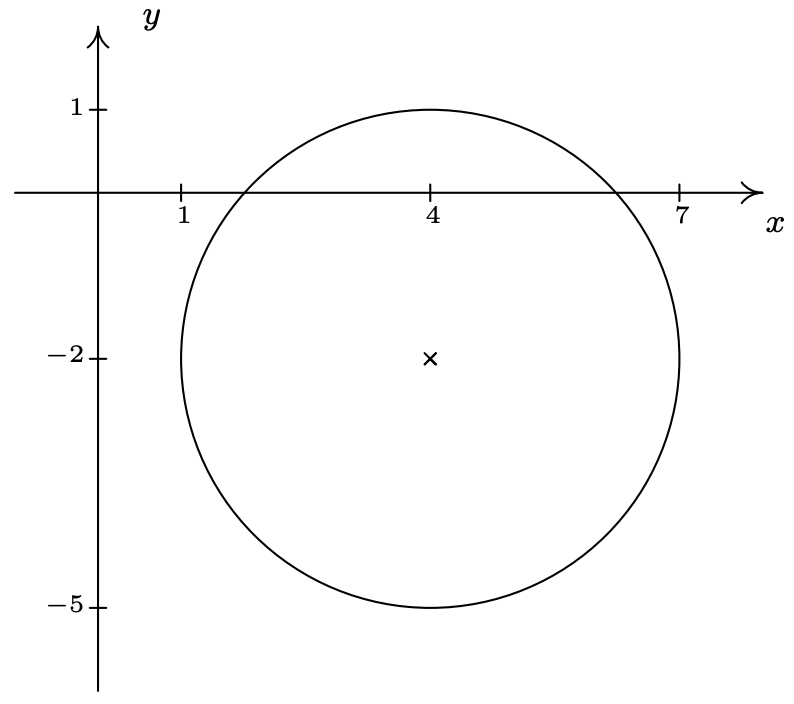
- Centro(4,−2), radio3
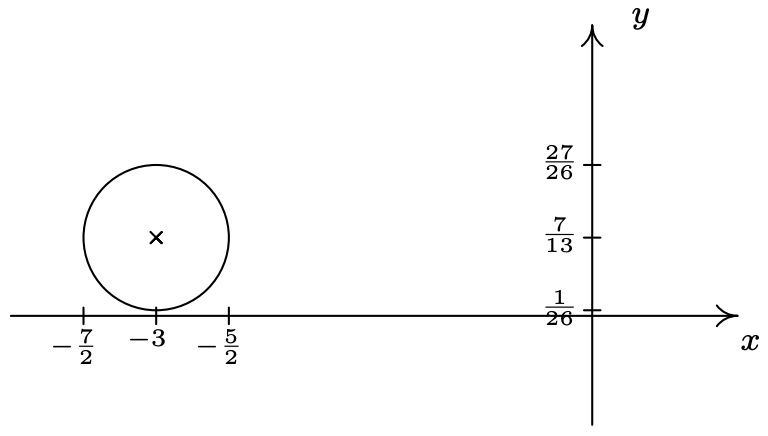
- Centro(−3,713), radio12
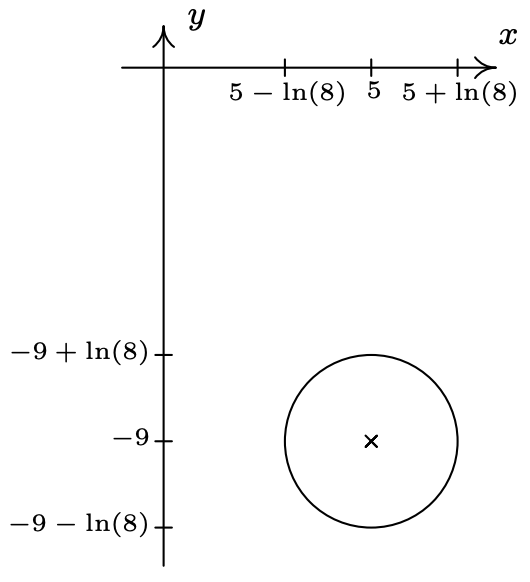
- Centro(5,−9), radioln(8)
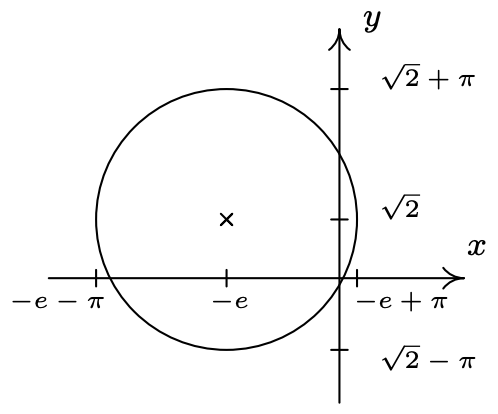
- Centro(−e,√2), radioπ
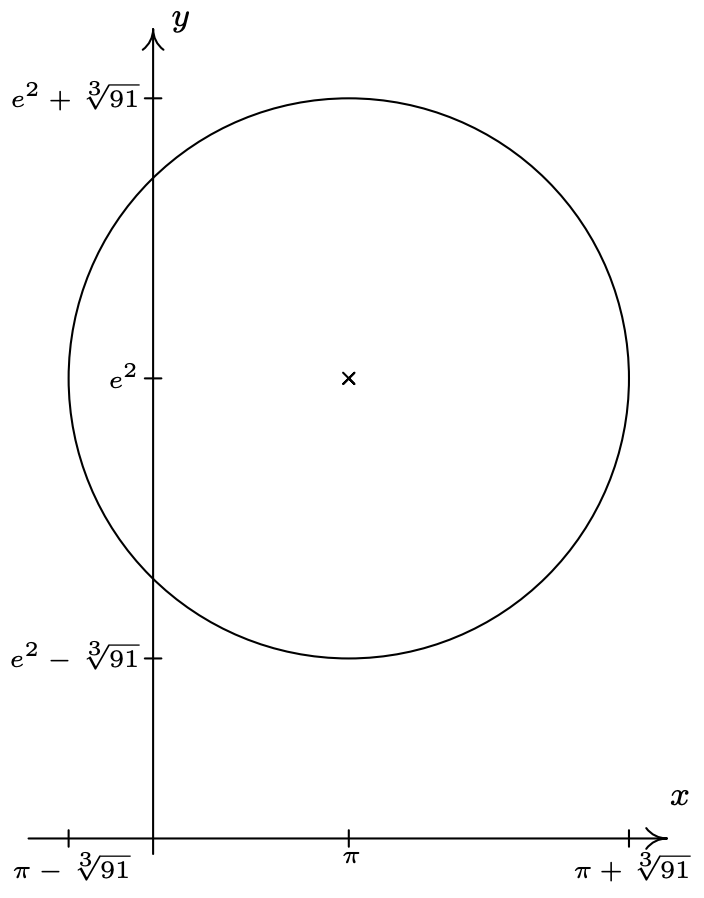
- Centro(π,e2), radio3√91
En los Ejercicios 7 - 12, complete el cuadrado para poner la ecuación en forma estándar. Identificar el centro y el radio o explicar por qué la ecuación no representa un círculo.
- x2−4x+y2+10y=−25
- −2x2−36x−2y2−112=0
- x2+y2+8x−10y−1=0
- x2+y2+5x−y−1=0
- 4x2+4y2−24y+36=0
- x2+x+y2−65y=1
En los Ejercicios 13 - 16, encuentra la ecuación estándar del círculo que satisface los criterios dados.
- centro(3,5), pasa a través(−1,−2)
- centro(3,6), pasa a través(−1,4)
- puntos finales de un diámetro:(3,6) y(−1,4)
- puntos finales de un diámetro:(12,4),(32,−1)
- La Rueda Gigante en Cedar Point es un círculo con 128 pies de diámetro que se asienta sobre una plataforma de 8 pies de altura haciendo que su altura total sea de 136 pies. 2 Encuentra una ecuación para la rueda asumiendo que su centro se encuentra en ely eje -y que el suelo es elx eje -eje.
- Verificar que los siguientes puntos se encuentran en el Círculo de Unidad:(±1,0),(0,±1),(±√22,±√22),(±12,±√32) y(±√32,±12)
- Discuta con sus compañeros de clase cómo obtener la ecuación estándar de un círculo, Ecuación 7.1, a partir de la ecuación del Círculo de Unidad,x2+y2=1 utilizando las transformaciones discutidas en la Sección 1.7. (Así, cada círculo está a solo unas transformaciones de distancia del Círculo de Unidad.)
- Encuentra una ecuación para la función representada gráficamente por la mitad superior del Círculo de Unidades. Explique cómo las transformaciones de la Sección 1.7 pueden ser utilizadas para producir una función cuya gráfica sea la parte superior o inferior de un círculo arbitrario.
- Encuentra una función uno a uno cuya gráfica sea la mitad de un círculo. (Pista: Piense poco a poco.)
7.2.2. RESPUESTAS
- (x+1)2+(y+5)2=100
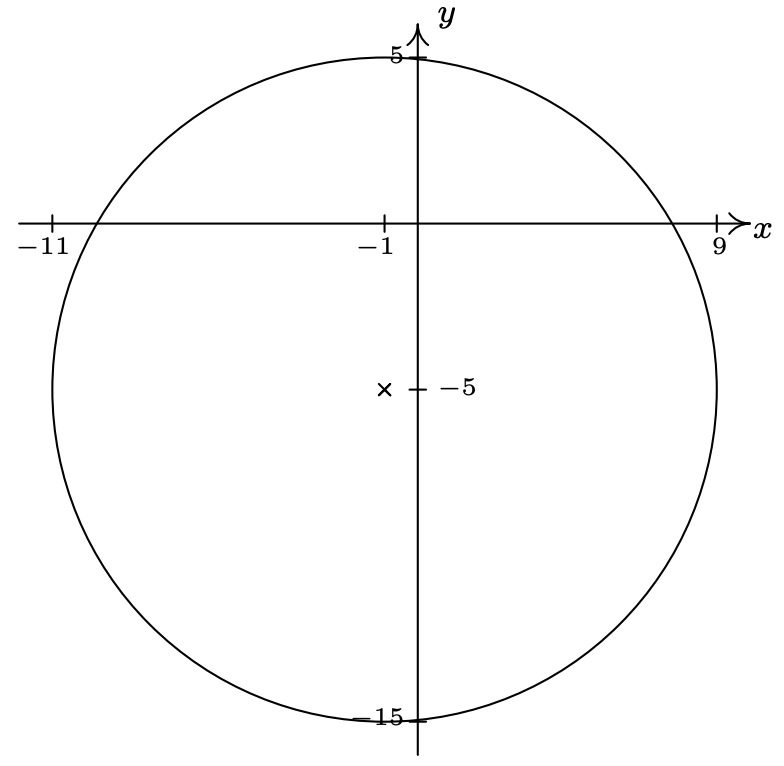
- (x−4)2+(y+2)2=9
- (x+3)2+(y−713)2=14
- (x−5)2+(y+9)2=(ln(8))2
- (x+e)2+(y−√2)2=π2
- (x−π)2+(y−e2)2=9123
-
(x−2)2+(y+5)2=4
Centro(2,−5), radior=2 -
(x+9)2+y2=25
Centro(−9,0), radior=5 -
(x+4)2+(y−5)2=42
Centro(−4,5), radior=√42 -
(x+52)2+(y−12)2=304
Centro(−52,12), radior=√302 -
x2+(y−3)2=0
Esto no es un círculo. -
(x+12)2+(y−35)2=161100
Centro(−12,35), radior=√16110 - (x−3)2+(y−5)2=65
- (x−3)2+(y−6)2=20
- (x−1)2+(y−5)2=5
- (x−1)2+(y−32)2=132
- x2+(y−72)2=4096
Referencia
1 Si bien esto puede parecer una opinión, efectivamente es un hecho. Consulte los Capítulos 10 y 11 para más detalles.
2 Fuente: Página web de Cedar Point.


