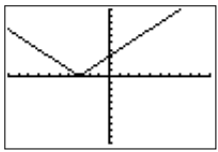
4.3: Ejercicios

Última actualización
Guardar como PDF

Page ID: 117644

Thomas Tradler and Holly Carley
CUNY New York City College of Technology via New York City College of Technology at CUNY Academic Works

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $ $ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $$\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$ $\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$$\newcommand{\AA}{\unicode[.8,0]{x212B}}$

Ejercicio$\PageIndex{1}$

Grafica la ecuación en la ventana estándar.

$y=3x-5$
$y=x^2-3x-2$
$y= x^4-3x^3+2x-1$
$y=\sqrt{x^2-4}$
$y=\dfrac 1 {x+2}$
$y=|x+3|$

Para el último ejercicio, el valor absoluto se obtiene presionando$\boxed {\text{math}}\boxed {\triangleright } \boxed {\text{enter}}$.

Responder

$clipboard_ec28580a0cb475221ae74f65a7b875a56.png$
$clipboard_e9639052f0518480c0a6772d864862076.png$
$clipboard_ea1d7f62ea6557b37425bd10c02a0af69.png$
$clipboard_e315786a025fabfb35de4054820a7eb18.png$
$clipboard_e1ec1c2b8561b7e8da1309cb0bed4db69.png$
$clipboard_e6d7f2f5f1bd0d3db032f5a0fb5a50e62.png$

Ejercicio$\PageIndex{2}$

Resuelve la ecuación para$y$ y grafica todas las ramas en una misma ventana.

$x^2+y^2=4$
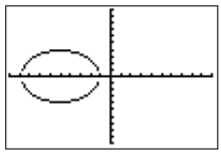
$(x+5)^2+y^2=15$
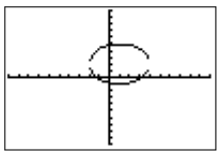
$(x-1)^2+(y-2)^2 = 9$
$y^2+x^2-8x-14=0$
$y^2=x^2+3$
$y^2=-x^2+77$

Responder

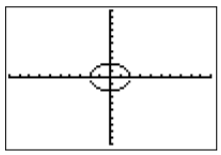
$y_{1}=\sqrt{4-x^{2}}$,$y_{2}=-\sqrt{4-x^{2}}$, $clipboard_e555203ea583554191525d0fc203c89b5.png$
$y_{1}=\sqrt{15-(x+5)^{2}}$,$y_{2}=-\sqrt{15-(x+5)^{2}}$, $clipboard_e036464d3ffa47eb8f571fee5ffbbeeba.png$
$y_1=2+\sqrt{9-(x-1)^{2}}$,$y_{2}=2-\sqrt{9-(x-1)^{2}}$, $clipboard_e0158735c0a9f6b3678a74ddb74b6c1ef.png$
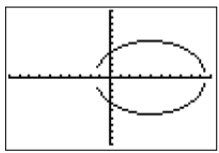
$y_{1}=\sqrt{-x^{2}+8 x+14}$,$y_2=-\sqrt{-x^{2}+8 x+14}$, $clipboard_e4d4e8cce97279d973b5dc08559a13d96.png$
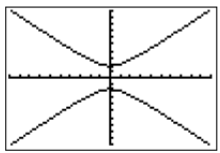
$y_{1}=\sqrt{x^{2}+3}$,$y_{2}=-\sqrt{x^{2}+3}$, $clipboard_e5e95ef043f4e9dc013b8e7d9a601f39e.png$
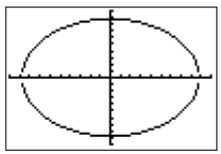
$y_{1}=\sqrt{-x^{2}+77}$,$y_{2}=-\sqrt{-x^{2}+77}$, $clipboard_e3af58a942e94955990b7dad10906a5f5.png$

Ejercicio$\PageIndex{3}$

Encuentra todos los ceros de la función dada. Redondee su respuesta a la centésima más cercana.

$f(x)=x^2+3x+1$
$f(x)=x^4-3x^2+2$
$f(x)=x^3+2x-6$
$f(x)=x^5-11x^4+20x^3+88x^2-224x+1$
$f(x)=x^3-5x^2+2x+3$
$f(x)=\sqrt{2^x-3}-2x+3$
$f(x)=0.04 x^3-0.02x^2-0.5174x+0.81$
$f(x)=0.04 x^3-0.02x^2-0.5175x+0.81$
$f(x)=0.04 x^3-0.02x^2-0.5176x+0.81$

Responder

$x \approx-2.62, x \approx-0.38$
$x=\pm 1, x=\pm \sqrt{2} \approx \pm 1.41$
$x \approx 1.46$
$x \approx-2.83, x \approx 0.01, x \approx 2.82, x \approx 4.01, x \approx 7.00$
$x \approx-0.578, x \approx 1.187, x \approx 4.388$
$x \approx 1.61, x=2, x \approx 6.91$
$x \approx-4.00$
$x=-4, x=2.25$
$x \approx-4.00, x \approx 2.22, x \approx 2.28$

Ejercicio$\PageIndex{4}$

Encuentra todas las soluciones de la ecuación. Redondee su respuesta a la milésima más cercana.

$x^3+3=x^5+7$
$4x^3+6x^2-3x-2=0$
$\dfrac{2x}{x-3}=\dfrac{x^2+2}{x+1}$
$5^{3x+1}=x^5+6$
$x^3+x^2=x^4-x^2+x$
$3x^2=x^3-x^2+3x$

Responder

$x \approx-1.488$
$x \approx-1.764, x \approx-0.416, x \approx 0.681$
$x \approx 5.220$
$x \approx-1.431, x \approx 0.038$
$x \approx-1.247, x=0, x \approx 0.445, x \approx 1.802$
$x=0, x=1, x=3$

Ejercicio$\PageIndex{5}$

Grafica la ecuación. Determine cuántos máximos y mínimos tiene la gráfica. Para ello, redimensiona la ventana gráfica (a través de las funciones de zoom, zoom y zoom-box de la calculadora) para acercar los máximos o mínimos del gráfico.

$y=x^2-4x+13$
$y=-x^2+x-20$
$y=2x^3 -5x^2+3x$
$y=x^4-5x^3+8x^2-5x+1$

Responder

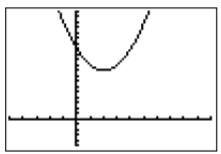
Hay un mínimo. Aleje el zoom para la gráfica. $clipboard_ea89937a4d3facf7eac223808ff617276.png$
Hay un máximo. Redimensiona la ventana a Ymin= −100. $clipboard_e3a05feccdf66f88d72b6bb4706e4b099.png$
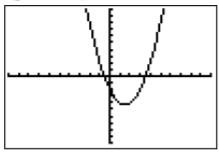
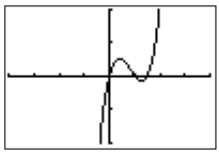
Hay un máximo local y un mínimo local. La gráfica con Xmin=−4, Xmax= 4, Ymin= −2, Ymax= 2 está abajo. $clipboard_e89ccc66825465c44830ab3e3a4ec160b.png$
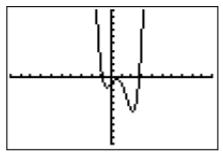
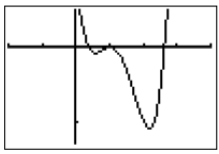
Al hacer zoom en la gráfica se revelan dos mínimos locales y un máximo local. Gráficamos la función con Xmin=−2, Xmax= 4, Ymin= −1.3, Ymax= 0.5. $clipboard_e679223dd8983c143a017fe9d69087df9.png$

Ejercicio$\PageIndex{6}$

Aproximar los máximos y mínimos (locales) de la gráfica. Redondee su respuesta a la décima más cercana.

$y=x^3+2x^2-x+1$
$y=x^3-5x^2+8x-3$
$y=-x^4+3x^3+x^2+2$
$y=x^4-x^3-4x^2+6x+2$
$y=x^4-x^3-4x^2+8x+2$
$y=x^4-x^3-4x^2+7x+2$

Responder

$clipboard_e29efa53019b5b1a94e684e58e5a6141f.png$
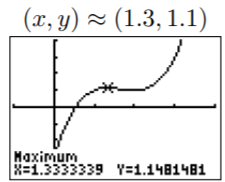
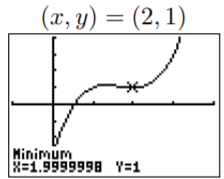
$clipboard_ed9a91c9ecd139448b4f5e54556561d68.png$ $clipboard_e82043561f8991ff0f6c44a6fe382b8a9.png$
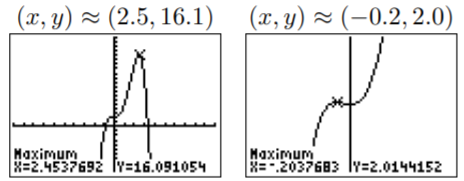
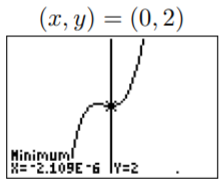
$clipboard_e9923bffd128206319d4a7da325631da7.png$ $clipboard_e1c88d6a252006a41c1b172532849a6a1.png$
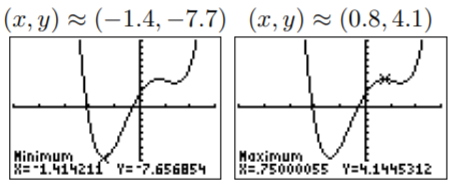
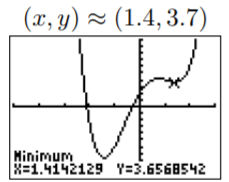
$clipboard_e1077580760809416dee5913010b0f7da.png$ $clipboard_e39115538cd162dcd08681b7867facf4b.png$
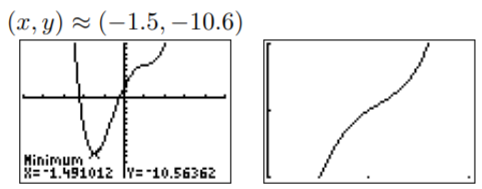$clipboard_eab01b98f8b96d5ca97de38f9ed227764.png$ (solo hay un mínimo y ningún máximo en la parte (e))

Ejercicio\(\PageIndex{1}\)

Ejercicio\(\PageIndex{2}\)

Ejercicio\(\PageIndex{3}\)

Ejercicio\(\PageIndex{4}\)

Ejercicio\(\PageIndex{5}\)

Ejercicio\(\PageIndex{6}\)