11.3: Ejercicios

Última actualización
Guardar como PDF

Page ID: 117682

Thomas Tradler and Holly Carley
CUNY New York City College of Technology via New York City College of Technology at CUNY Academic Works

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $ $ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $$\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$ $\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$$\newcommand{\AA}{\unicode[.8,0]{x212B}}$

Ejercicio$\PageIndex{1}$

Encontrar el dominio, las asíntotas verticales y las discontinuidades removibles de las funciones.

$f(x)=\dfrac{2}{x-2}$
$f(x)=\dfrac{x^2+2}{x^2-6x+8}$
$f(x)=\dfrac{3x+6}{x^3-4x}$
$f(x)=\dfrac{(x-2)(x+3)(x+4)}{(x-2)^2(x+3)(x-5)}$
$f(x)=\dfrac{x-1}{x^3-1}$
$f(x)=\dfrac{2}{x^3-2x^2-x+2}$

Contestar

dominio$D = \mathbb{R} − \{2\}$, asíntota vertical en$x = 2$, sin discontinuidades removibles
$D = \mathbb{R}−\{2, 4\}$, asíntesis vertical. a$x = 2$ y$x = 4$, no descont removible.
$D = \mathbb{R}− \{−2, 0, 2\}$, asíntesis vertical. a$x = 0$ y$x = 2$, descontíon removible.$x = −2$
$D = \mathbb{R} − \{−3, 2, 5\}$, asíntesis vertical. a$x = 2$ y$x = 5$, descontíon removible.$x = −3$
$D = \mathbb{R} − \{1\}$, sin asíntesis vertical., descont. removible a$x = 1$
$D = \mathbb{R} − \{−1, 1, 2\}$, asíntesis vertical. a$x = −1$ y$x = 1$ y$x = 2$, no descont removible.

Ejercicio$\PageIndex{2}$

Encuentra las asíntotas horizontales de las funciones.

$f(x)=\dfrac{8x^2+2x+1}{2x^2+3x-2}$
$f(x)=\dfrac{1}{(x-3)^2}$
$f(x)=\dfrac{x^2+3x+2}{x-1}$
$f(x)=\dfrac{12x^3-4x+2}{-3x^3+2x^2+1}$

Contestar

$y = 4$
$y = 0$
sin asíntota horizontal (comportamiento asintótico$y = x + 4$)
$y = −4$

Ejercicio$\PageIndex{3}$

Encuentra las$x$ - y$y$ -intercepciones de las funciones.

$f(x)=\dfrac{x-3}{x-1}$
$f(x)=\dfrac{x^3-4x}{x^2-8x+15}$
$f(x)=\dfrac{(x-3)(x-1)(x+4)}{(x-2)(x-5)}$
$f(x)=\dfrac{x^2+5x+6}{x^2+2x}$

Contestar

$x$-interceptar en$x = 3$,$y$ -interceptar en$y = 3$
$x$-intercepta en$x = 0$$x = −2$ y y$x = 2$$y$ -interceptar en$y = 0$
$x$-intercepta en$x = −4$$x = 1$ y y$x = 3$$y$ -interceptar en$y = \dfrac{6}{5}$
$x$-intercept at$x = −3$ (pero no at$x = −2$ ya que no$f(−2)$ está definido), no$y$ -intercept ya que no$f(0)$ está definido

Ejercicio$\PageIndex{4}$

Esbozar la gráfica de la función$f$ utilizando el dominio de$f$, las asíntotas horizontales y verticales, las singularidades removibles, las$x$ - y$y$ -intercepciones de la función, junto con un boceto de la gráfica obtenida del calculadora.

$f(x)=\dfrac{6x-2}{2x+4}$
$f(x)=\dfrac{x-3}{x^3-3x^2-6x+8}$
$f(x)=\dfrac{x^4-10x^2+9}{x^2-3x+2}$
$f(x)=\dfrac{x^3-3x^2-x+3}{x^3-2x^2}$

Contestar

$D = \mathbb{R} − \{2\}$, asíntesis horizontal. $y = 3$, asíntesis vertical. $x = −2$, no descont removible.,$x$ -interceptar en$x = \dfrac 1 3$,$y$ -interceptar en$y = \dfrac{-1}{2}$, graficar:

$clipboard_ecbbba7ef054ee5a490309ff6ad0fbf4b.png$

$f(x)=\dfrac{x-3}{(x-4)(x-1)(x+2)}$tiene dominio$D = \mathbb{R} − \{−2, 1, 4\}$, asínmpta horizontal. $y = 0$, asíntesis vertical. $x = −2$y$x = 1$ y$x = 4$, no descont removible.,$x$ -interceptar en$x = 3$,$y$ -interceptar en$y = \dfrac{-3}{8} = −0.375$, graficar:

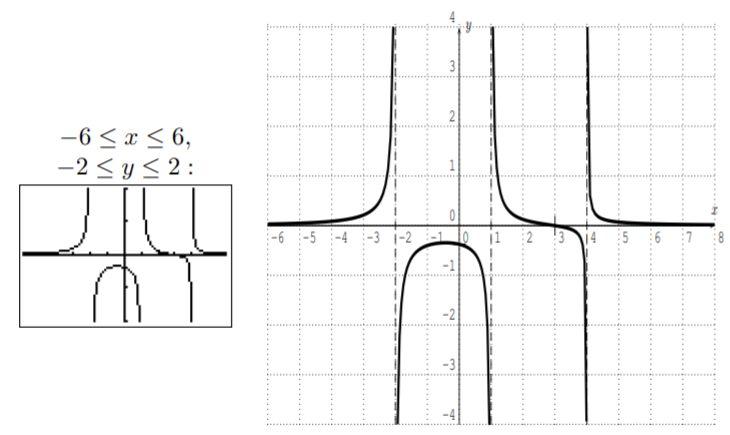
$clipboard_e0d6db6e49d48f2bad98ab2b86fb86e1b.png$

$f(x)=\dfrac{(x-3)(x+3)(x-1)(x+1)}{(x-2)(x-1)}$tiene dominio$D = \mathbb{R} − \{1, 2\}$, sin asíntesis horizontal, asínptica vertical. $x = 2$, descont. removible en$x = 1$,$x$ -interceptar en$x = −3$ y$x = −1$ y$x = 3$,$y$ -interceptar en$y = \dfrac 9 2 = 4.5$, graficar:

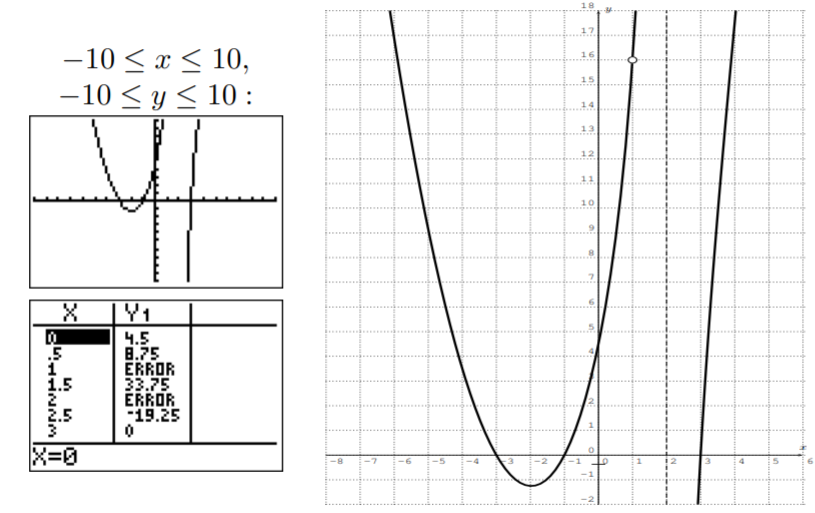
$clipboard_e3ce9e8190cd29dd861506874a33ab2d1.png$

$f(x)=\dfrac{(x-3)(x-1)(x+1)}{x^{2}(x-2)}$tiene dominio$D = \mathbb{R} − \{0, 2\}$, asínmpta horizontal. $y = 1$, asíntesis vertical. $x = 0$y$x = 2$, sin descont removible.,$x$ -intercepta en$x = −1$ y$x = 1$ y$x = 3$, no$y$ -interceptar ya que no$f(0)$ está definido, graph:

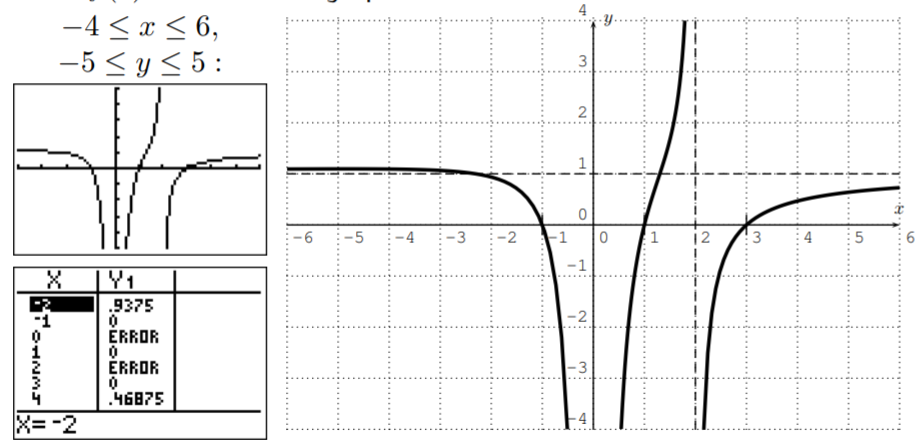
$clipboard_e46938e7afbc22f2db2e88901bdcc36c6.png$

Tenga en cuenta que la gráfica cruza la asíntota horizontal aproximadamente$x \approx-2.3$ y se acerca$y = 1$ a la asíntota desde arriba

Ejercicio$\PageIndex{5}$

Encuentra una función racional$f$ que satisfaga todas las propiedades dadas.

asíntota vertical en$x=4$ y asíntota horizontal$y=0$
asíntotas verticales en$x=2$$x=3$ y asíntota horizontal$y=5$
singularidad removible en$x=1$ y sin asíntota horizontal

Contestar

por ejemplo$f(x)=\dfrac{1}{x-4}$
por ejemplo$f(x)=\dfrac{5 x^{2}}{x^{2}-5 x+6}$
por ejemplo$f(x)=\dfrac{x^{2}-x}{x-1}$

Ejercicio\(\PageIndex{1}\)

Ejercicio\(\PageIndex{2}\)

Ejercicio\(\PageIndex{3}\)

Ejercicio\(\PageIndex{4}\)

Ejercicio\(\PageIndex{5}\)