2.2.4: Resolver triángulos rectos
( \newcommand{\kernel}{\mathrm{null}\,}\)
Usando las funciones trigonométricas inversas para resolver la falta de información sobre los triángulos rectos.
Ratios trigonométricos inversos
En matemáticas, la palabra inversa significa “deshacer”. Por ejemplo, la suma y la resta son inversas entre sí porque una deshace a la otra. Cuando utilizamos las relaciones trigonométricas inversas, podemos encontrar medidas de ángulo agudo siempre y cuando se nos den dos lados.
Tangente inversa: Etiquetadotan−1, el “-1” significa inverso.
tan−1(ba)=m∠Bytan−1(ab)=m∠A.
Seno Inversa: Etiquetadosin−1.
sin−1(bc)=m∠Bysin−1(ac)=m∠A.
Coseno inverso: Etiquetadocos−1.
cos−1(ac)=m∠Bycos−1(bc)=m∠A.
En la mayoría de los problemas, para encontrar la medida de los ángulos necesitarás usar tu calculadora. En la mayoría de las calculadoras científicas y gráficas, los botones se ven como[sin−1],[cos−1], y[\(tan−1]. También es posible que tengas que presionar un botón de turno o segundo para acceder a estas funciones.
Ahora que conoces tanto las relaciones trigonométricas como las relaciones trigonométricas inversas puedes resolver un triángulo rectángulo. Para resolver un triángulo rectángulo, necesitas encontrar todos los lados y ángulos en él. Usualmente usarás seno, coseno o tangente; seno inverso, coseno inverso o tangente inversa; o el teorema de Pitágoras.
¿Y si te dijeran que la tangente de∠Z es 0.6494? ¿Cómo podrías encontrar la medida de∠Z?
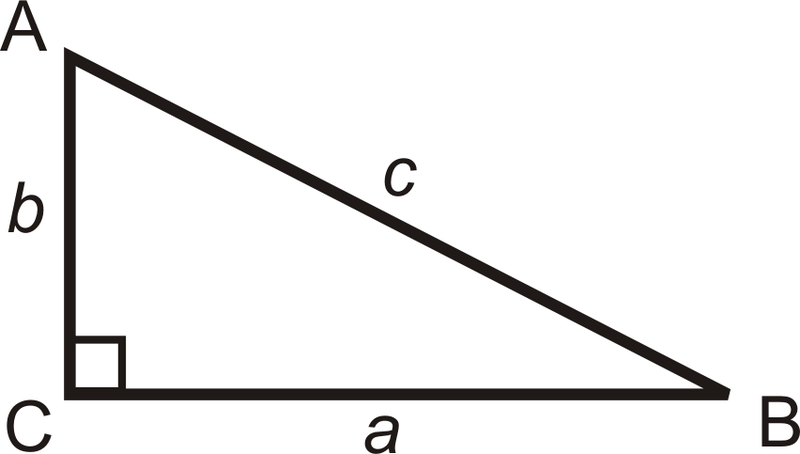
Resuelve el triángulo rectángulo.
Solución
Los dos ángulos agudos son congruentes, haciéndolos ambos45∘. Se trata de un triángulo 45-45-90. Se pueden utilizar las relaciones trigonométricas o las relaciones especiales de triángulo rectángulo.
Relaciones trigonométricas
\ (\ begin {array} {rlrl}
\ tan 45^ {\ circ} & =\ dfrac {15} {B C} &\ sin 45^ {\ circ} & =\ dfrac {15} {A C}\\
B C & =\ dfrac {15} {\ tan 45^ {\ circ}} =15 & A C & =\ dfrac {15} {\ sin 45^ {\ circ}}\ aprox 21.21
\ fin {array}\)
Relaciones de triángulo 45-45-90
BC=AB=15, AC=15√2≈21.21
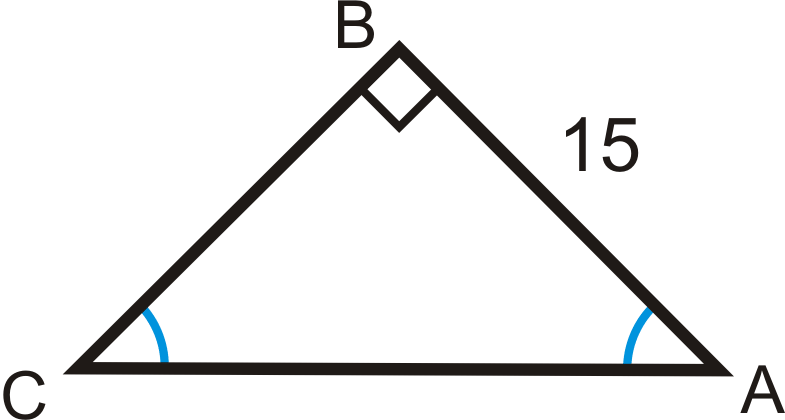
Usa los lados del triángulo y tu calculadora para encontrar el valor de∠A. Redondee su respuesta al décimo de grado más cercano.

Solución
En referencia a∠A, se nos da la pierna opuesta y la pierna adyacente. Esto significa que debemos usar la relación tangente.
tanA=2025=45. Entonces,tan−145=m∠A. Ahora, usa tu calculadora.
Si estás usando un TI-83 o 84, las pulsaciones de teclas serían: [2nd] [TAN] (45) [ENTER] y la pantalla se ve así:

m∠A≈38.7∘
∠Aes un ángulo agudo en un triángulo rectángulo. Encontrarm∠A al décimo de grado más cercano parasinA=0.68,cosA=0.85, ytanA=0.34.
Solución
m∠A=sin−10.68≈42.8∘m∠A=cos−10.85≈31.8∘m∠A=tan−10.34≈18.8∘
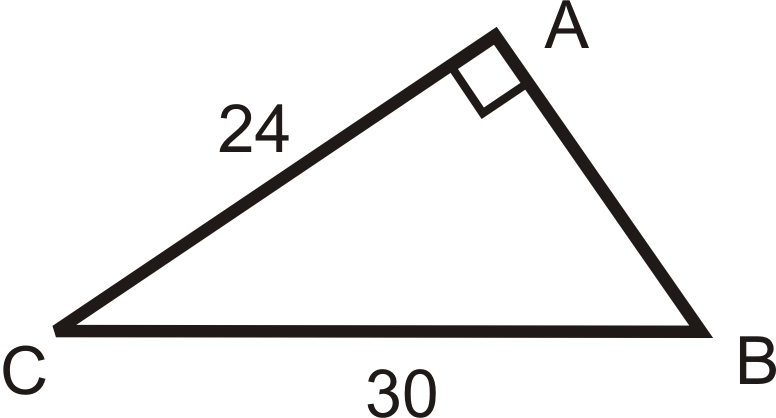
Resuelve el triángulo rectángulo.
Solución
Para resolver este triángulo rectángulo, necesitamos encontrarAB,m∠C ym∠B. Usa solo los valores que te den.
AB_: Use the Pythagorean Theorem.
242+AB2=302576+AB2=900AB2=324AB=√324=18
m∠B_: Use the inverse sine ratio.
sinB=2430=45sin−1(45)≈53.1∘=m∠B
m∠C_: Use the inverse cosine ratio.
cosC=2430=45→cos−1(45)≈36.9∘=m∠C
¿Cuándo usarías el pecado y cuándo usaríassin−1?
Solución
Usarías pecado cuando te dan un ángulo y estás resolviendo por un lado faltante. Lo usaríassin−1 cuando te dan lados y estás resolviendo un ángulo faltante.
Revisar
Usa tu calculadora para encontrarm∠A al décimo de grado más cercano.
-
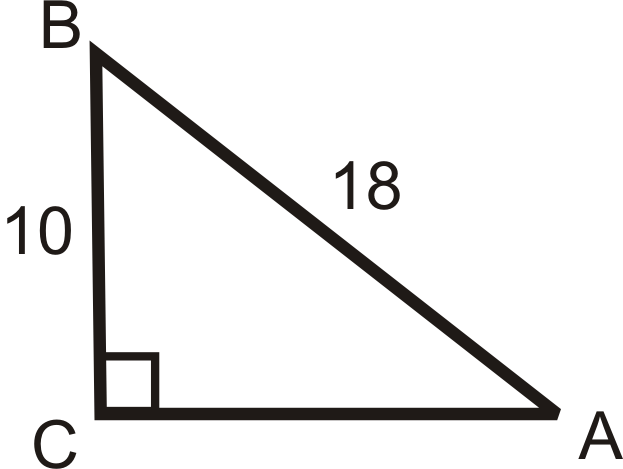
Figura2.2.4.6 -
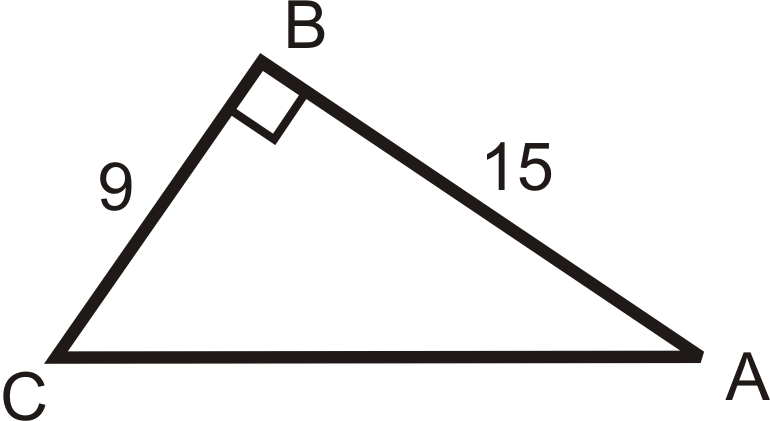
Figura2.2.4.7 -
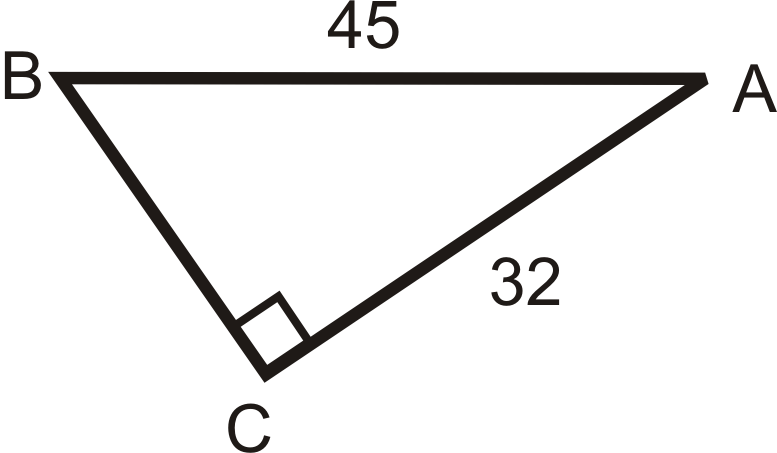
Figura2.2.4.8 -
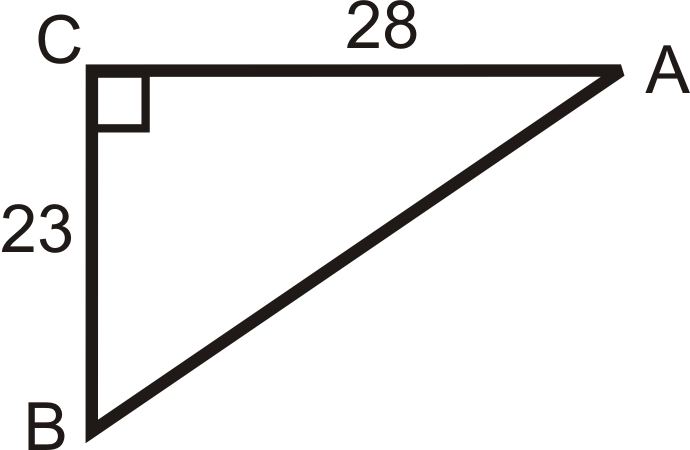
Figura2.2.4.9 -
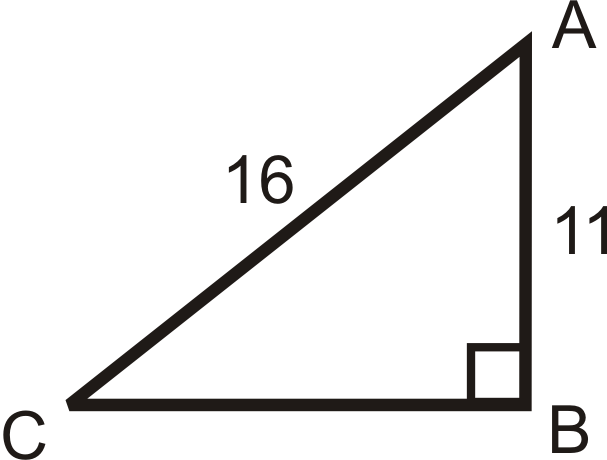
Figura2.2.4.10 -
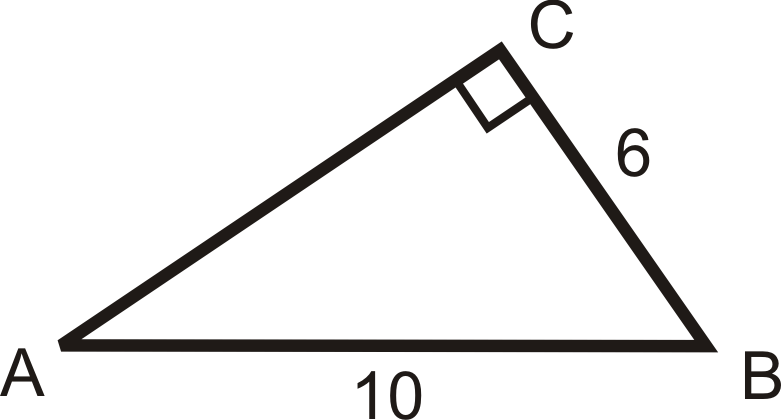
Figura2.2.4.11
Dejar∠A ser un ángulo agudo en un triángulo rectángulo. Encuentram∠A al décimo de grado más cercano.
- sinA=0.5684
- cosA=0.1234
- tanA=2.78
- cos−10.9845
- tan−115.93
- sin−10.7851
Resolviendo los siguientes triángulos rectos. Encuentra todos los lados y ángulos faltantes. Redondear cualquier respuesta decimal a la décima más cercana.
-
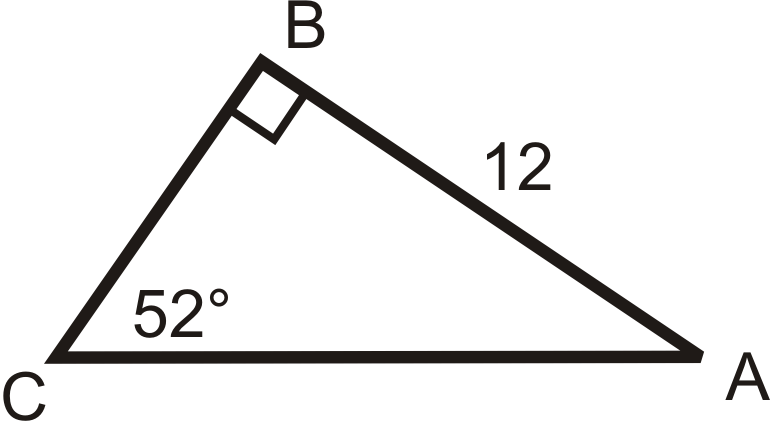
Figura2.2.4.12 -
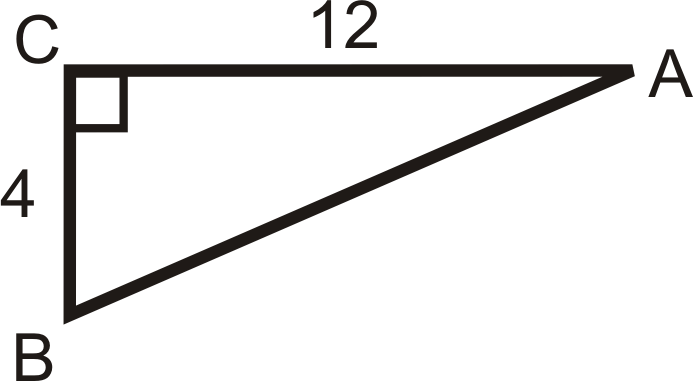
Figura2.2.4.13 -
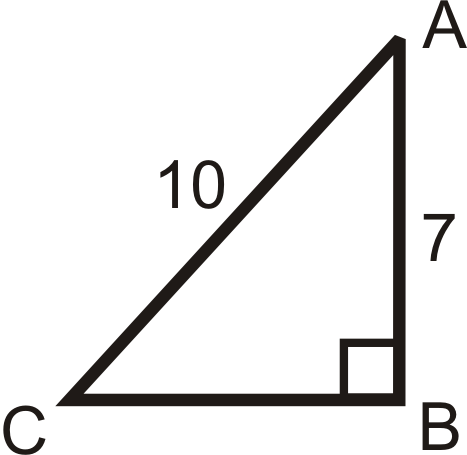
Figura2.2.4.14 -
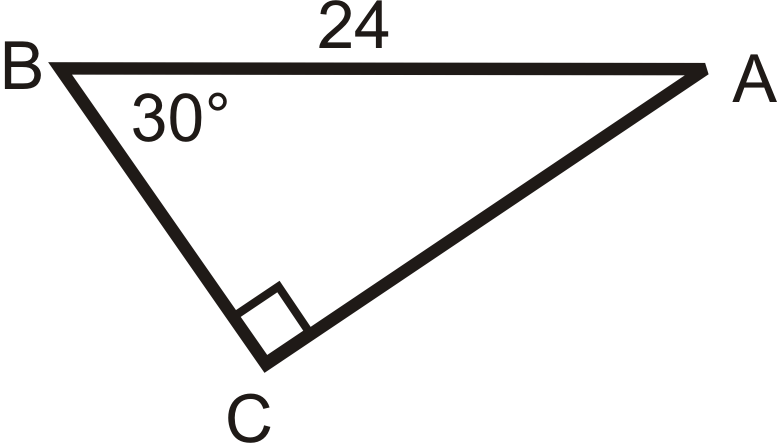
Figura2.2.4.15 -

Figura2.2.4.16 -
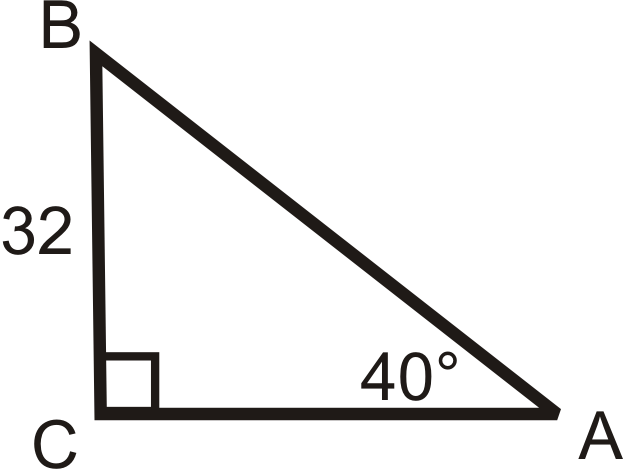
Figura2.2.4.17 -
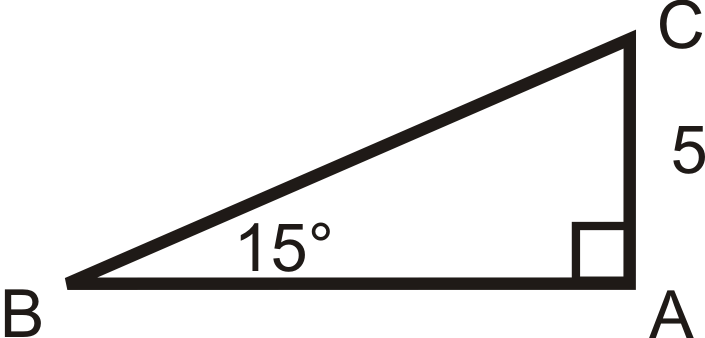
Figura2.2.4.18 -
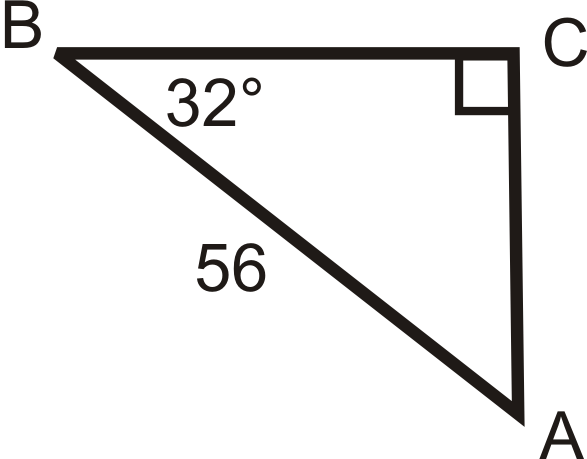
Figura2.2.4.19 -

Figura2.2.4.20
Reseña (Respuestas)
Para ver las respuestas de Revisar, abra este archivo PDF y busque la sección 8.10.
Recursos adicionales
Video: Introducción a las funciones trigonométricas inversas
Actividades: Ratios trigonométricos inversos Preguntas de discusión
Ayudas de estudio: Guía de estudio de relaciones trigonométricas
Práctica: Resolver triángulos rectos


