10.8: Graficar soluciones cuadráticas
- Page ID
- 112069
Parabolas
Ahora estudiaremos las gráficas de ecuaciones cuadráticas en dos variables con forma general
\(y = ax^2 + bx + c, a \not= 0\). \(a, b, c\)son números reales.
Parábola
Todas esas gráficas tienen una forma similar. La gráfica de una ecuación cuadrática de este tipo Parábola se denomina parábola y asumirá una de las siguientes formas.
Vértice
El punto alto o punto bajo de una parábola se llama el vértice de la parábola.
Construyendo Gráficas de Parábola
Construiremos la gráfica de una parábola eligiendo varios valores x, calculando para encontrar los valores y correspondientes, trazando estos pares ordenados, luego dibujando una curva suave a través de ellos.
Conjunto de Muestras A
Gráfica\(y = x^2\). Construir una tabla para exhibir varios pares ordenados.
| \(x\) | \(y=x^2\) |
| 0 | 0 |
| 1 | 1 |
| 2 | 4 |
| 3 | 9 |
| −1 | 1 |
| −2 | 4 |
| −3 | 9 |
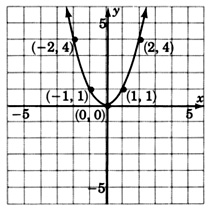
Esta es la parábola más básica. Si bien otras parabolas pueden ser más anchas, más estrechas, movidas hacia arriba o hacia abajo, movidas hacia la izquierda o hacia la derecha, o invertidas, todas tendrán esta misma forma básica. Tendremos que trazar tantos pares ordenados como sea necesario para asegurar esta forma básica.
Gráfica\(y = x^2 - 2\). Construir una tabla de pares ordenados.
| \(x\) | \(y=x^2 -2\) |
| 0 | −2 |
| 1 | −1 |
| 2 | 2 |
| 3 | 7 |
| −1 | −1 |
| −2 | 2 |
| −3 | 7 |
Observe que la gráfica de\(y = x^2 - 2\) es precisamente la gráfica de\(y = x^2\) pero tradujo 2 unidades hacia abajo. Comparar las ecuaciones de\(y = x^2\) y\(y = x^2 - 2\). ¿Ves qué causa la traducción descendente de 2 unidades?
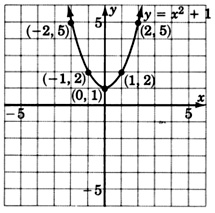
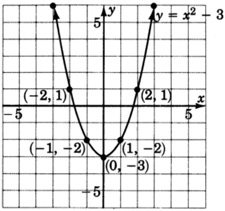
Conjunto de práctica A
Usa la idea sugerida en el Conjunto de Muestras A para bosquejar (de forma rápida y quizás no perfectamente precisa) las gráficas de
\(y = x^2 + 1\)y\(y = x^2 - 3\)
- Contestar
-
Conjunto de Muestras B
Gráfica\(y = (x + 2)^2\).
¿Esperamos que la gráfica sea similar a la gráfica de\(y = x^2\)? Hacer una mesa de pares ordenados.
| \(x\) | \(y\) |
| 0 | 4 |
| 1 | 9 |
| −1 | 1 |
| −2 | 0 |
| −3 | 1 |
| −4 | 4 |
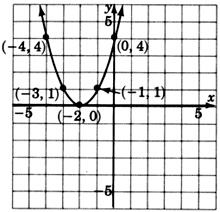
Observe que la gráfica de\(y = (x + 2)^2\) es precisamente la gráfica de\(y = x^2\) pero traducida 2 unidades a la izquierda. El\(+2\) interior de los paréntesis mueve\(y = x^2\) dos unidades hacia la izquierda. Un valor negativo dentro de los paréntesis hace un movimiento hacia la derecha.
Set de práctica B
Usa la idea sugerida en el Conjunto de Muestras B para esbozar las gráficas de
\(y = (x-3)^2\)y\(y = (x + 1)^2\)
- Contestar
-
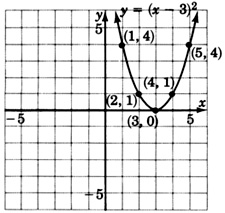
Gráfica\(y = (x-2)^2 + 1\)
- Contestar
-
Ejercicios
Para los siguientes problemas, graficar las ecuaciones cuadráticas.
\(y = x^2\)
- Contestar
-
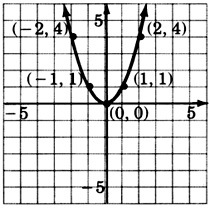
\(y = -x^2\)
\(y = (x-1)^2\)
- Contestar
-
\(y = (x-2)^2\)
\(y = (x + 3)^2\)
\(y = (x + 3)^2\)
- Contestar
-
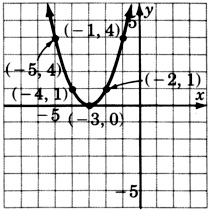
\(y = (x + 1)^2\)
\(y = x^2 - 3\)
- Contestar
-
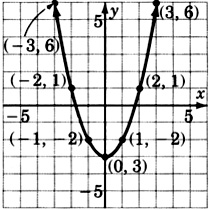
\(y = x^2 - 1\)
\(y = x^2 + 2\)
- Contestar
-
\(y = x^2 + \dfrac{1}{2}\)
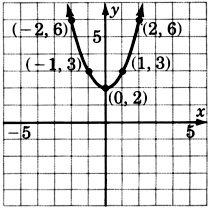
\(y = x^2 - \dfrac{1}{2}\)
- Contestar
-
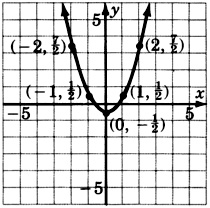
\(y = -x^2 + 1\)(comparar con el problema 2)
\(y = -x^2 - 1\)(comparar con el problema 1)
- Contestar
-
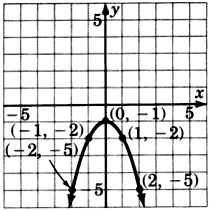
\(y = (x-1)^2 - 1\)
\(y = (x + 3)^2 + 2\)
- Contestar
-
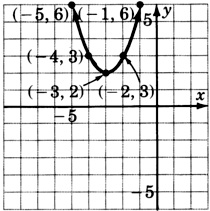
\(y = -(x + 1)^2\)
\(y = -(x + 3)^2\)
- Contestar
-
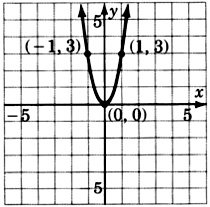
\(y = 2x^2\)
\(y = 3x^2\)
- Contestar
-
\(y = \dfrac{1}{2}x^2\)
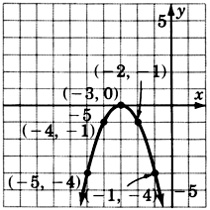
Para los siguientes problemas, trate de adivinar la ecuación cuadrática que corresponde a la gráfica dada.
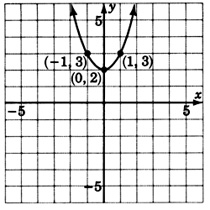
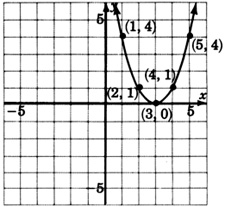
- Contestar
-
\(y = (x-3)^2\)
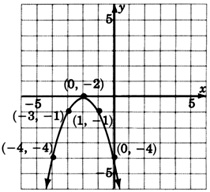
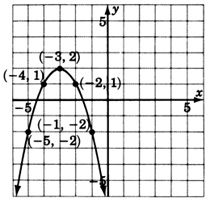
- Contestar
-
\(y = -(x + 3)^2 + 2\)
Ejercicios para revisión
Simplifica y escribe\((x^{-4}y^5)^{-3}(x^{-6}y^4)^2\) para que solo aparezcan exponentes positivos.
Factor\(y^2 - y - 42\)
- Contestar
-
\((y+6)(y−7)\)
Encuentra la suma:\(\dfrac{2}{a - 3} + \dfrac{3}{a + 3} + \dfrac{18}{a^2 - 9}\)
Simplificar\(\dfrac{2}{4 + \sqrt{5}}\)
- Contestar
-
\(\dfrac{8 - 2\sqrt{5}}{11}\)
Cuatro se agrega a un entero y esa suma se duplica. Cuando este resultado se multiplica por el entero original, el producto es\(-6\). Encuentra el entero.