12.9: Trigonometría hiperbólica
( \newcommand{\kernel}{\mathrm{null}\,}\)
En esta sección damos fórmulas para h-distancia usando funciones hiperbólicas. Una de estas fórmulas se utilizará en la prueba del teorema hiperbólico de Pitágoras (Teorema 13.6.1).
Recordemos que\cosh\sinh, y\tanh denotan coseno hiperbólico, seno hiperbólico y tangente hiperbólica; es decir, las funciones definidas por
\cosh x := \dfrac{e^x + e^{-x}}{2},\sinh x := \dfrac{e^x - e^{-x}}{2},
\tanh x := \dfrac{\sinh x}{\cosh x}.
Estas funciones hiperbólicas son análogas a seno y coseno y tangente.
Demostrar las siguientes identidades:
\cosh' x=\sinh x;\sinh'x=\cosh x;(\cosh x)^2-(\sinh x)^2=1.
Las identidades
\cosh (2 \cdot x) = (\cosh x)^2 + (\sinh x)^2y\sinh (2 \cdot x) = 2 \cdot \sinh x \cdot \cosh x
mantener por cualquier valor realx.
- Prueba
-
\begin{array} {rcl} {(\sinh x)^2 + (\cosh x)^2} & = & {(\dfrac{e^x - e^{-x}}{2})^2 + (\dfrac{e^x + e^{-x}}{2})^2 =} \\ {} & = & {\dfrac{e^{2 \cdot x} + e^{-2 \cdot x}}{2} =} \\ {} & = & {\cosh (2 \cdot x);} \end{array}
\begin{array} {rcl} {2 \cdot \sinh x \cdot cosh x} & = & {2 \cdot (\dfrac{e^x - e^{-x}}{2}) \cdot (\dfrac{e^x + e^{-x}}{2})} \\ {} & = & {\dfrac{e^{2 \cdot x} - e^{-2 \cdot x}}{2}} \\ {} & = & {\cosh (2 \cdot x).} \end{array}
DejarP yQ ser dos h-poins distintos del centro de absoluto. Denotar porP' yQ' las inversas deP yQ en lo absoluto.
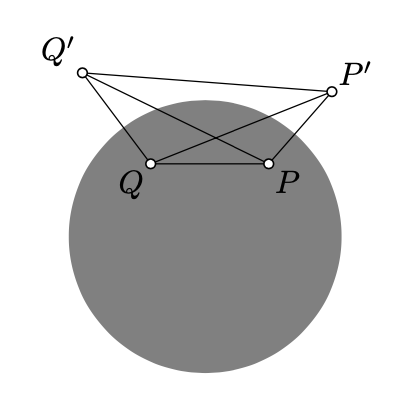
(a)\cosh [\dfrac{1}{2} \cdot PQ_h] = \sqrt{\dfrac{PQ' \cdot P'Q}{PP' \cdot QQ'}};
b)\sinh [\dfrac{1}{2} \cdot PQ_h] = \sqrt{\dfrac{PQ \cdot P'Q'}{PP' \cdot QQ'}};
c)\tanh [\dfrac{1}{2} \cdot PQ_h] = \sqrt{\dfrac{PQ \cdot P'Q'}{PQ' \cdot P'Q}};
d)\cosh PQ_h = \dfrac{PQ \cdot P'Q' + PQ' \cdot P'Q}{PP' \cdot QQ'}.
- Insinuación
-
Por Corolario 10.6.1 y Teorema 10.2.1, los lados derechos en las identidades sobreviven bajo una inversión en un círculo perpendicular a lo absoluto.
Como de costumbre suponemos que el absoluto es un círculo unitario. Supongamos queO denota el punto medio h de[PQ]_h. Por la observación principal (Teorema 12.3.1) podemos suponer queO es el centro de lo absoluto. En este caso tambiénO es el punto medio euclidiano de[P Q]. (En cambio, podemos movernosQ al centro de lo absoluto. En este caso las derivaciones son más sencillas. Pero desde entoncesQ'Q = Q'P = QP = \infty, uno tiene que justificar eso\dfrac{\infty}{\infty} = 1 cada vez.)
Seta = OP = OQ; en este caso tenemos
\begin{array} {rcl} {PQ} & = & {2 \cdot a,} \\ {P'Q'} & = & {2 \cdot \dfrac{1}{a},} \end{array}\begin{array} {l} {PP' = QQ' = \dfrac{1}{a} - a,} \\ {PQ' = QP' = \dfrac{1}{a} + a.} \end{array}
y
PQ_h = \ln \dfrac{(1 + a)^2}{(1 - a)^2} = 2 \cdot \ln \dfrac{1 + a}{1 - a}.
Por lo tanto
\begin{array} {rcl} {\cosh [\dfrac{1}{2} \cdot PQ_h]} & = & {\dfrac{1}{2} \cdot (\dfrac{1 + a}{1 - a} + \dfrac{1 - a}{1 + a})=} \\ {} & = & {\dfrac{1 + a^2}{1 - a^2};} \end{array}\begin{array} {rcl} {\sqrt{\dfrac{PQ' \cdot P'Q}{PP' \cdot QQ'}}} & = & {\dfrac{\dfrac{1}{a} + a}{\dfrac{1}{a} - a} =} \\ {} & = & {\dfrac{1 + a^2}{1 - a^2}.} \end{array}
De ahí que siga la parte a). Del mismo modo,
\begin{array} {rcl} {\sinh [\dfrac{1}{2} \cdot PQ_h]} & = & {\dfrac{1}{2} \cdot (\dfrac{1 + a}{1 - a} - \dfrac{1 - a}{1 + a}) =} \\ {} & = & {\dfrac{2 \cdot a}{1 - a^2};} \end{array}\begin{array} {rcl} {\sqrt{\dfrac{PQ \cdot P'Q'}{PP' \cdot QQ'}}} & = & {\dfrac{2}{\dfrac{1}{a} - a} =} \\ {} & = & {\dfrac{2 \cdot a}{1 - a^2}.} \end{array}
De ahí que siga la parte b).
Las partes (c) y (d) siguen de (a), (b), la definición de tangente hiperbólica, y la identidad de doble argumento para el coseno hiperbólico, ver Teorema\PageIndex{1}.

