1.8: Una interpretación geométrica de las derivadas
- Page ID
- 117180
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Recordemos que si\(y=f(x),\) entonces, para cualquier número real\(\Delta x\),
\[\frac{\Delta y}{\Delta x}=\frac{f(x+\Delta x)-f(x)}{\Delta x}\]es la tasa promedio de cambio de\(y\) con respecto a\(x\) sobre el intervalo\([x, x+\Delta x]\) (ver\((1.2 .7)) .\) Ahora si la gráfica de\(y\) es una línea recta, es decir, si\(f(x)=m x+b\) para algunos números reales\(m\) y\(b,\) luego\((1.8 .1)\) es\(m,\) la pendiente de la línea. De hecho, una línea recta se caracteriza porque el hecho\((1.8 .1)\) es el mismo para cualquier valor de\(x\) y\(\Delta x .\) Además,\((1.8 .1)\) permanece igual cuando\(\Delta x\) es infinitesimal; es decir, la derivada de\(y\) con respecto a\(x\) es la pendiente de la línea. Para otras funciones diferenciables\(f,\) el valor de\((1.8 .1)\) depende de ambos\(x\) y\(\Delta x .\) Sin embargo, para los valores infinitesimales de\(\Delta x,\) la sombra de\((1.8 .1),\) eso es, la derivada\(\frac{d y}{d x},\) depende\(x\) solo de. De ahí que sea razonable pensar en la pendiente de la curva\(y=f(x)\) en un punto\(x .\) Mientras que la pendiente de una recta es constante de punto a punto, para otras funciones diferenciables el valor de la pendiente de la curva variará de punto a punto.\(\frac{d y}{d x}\) Si\(f\) es diferenciable en un punto\(a,\) llamamos a la línea con pendiente que\(f^{\prime}(a)\) pasa por\((a, f(a))\) la línea tangente a la gráfica de\(f\) at Es\((a, f(a)) .\) decir, la línea tangente a la gráfica de\(y=f(x)\) at\(x=a\) es la línea con ecuación\[y=f^{\prime}(a)(x-a)+f(a) .\] De ahí un línea tangente a la gráfica de una función\(f\) es una línea a través de un punto en la gráfica\(f\) cuya pendiente es igual a la pendiente de la gráfica en ese punto.
Ejemplo\(\PageIndex{1}\)
Si\(f(x)=x^{5}-6 x^{2}+5,\) entonces
\[f^{\prime}(x)=5 x^{4}-12 x .\]En particular,\(f^{\prime}\left(-\frac{1}{2}\right)=\frac{101}{16},\) y así la ecuación de la línea tangente a la gráfica de\(f\) at\(x=-\frac{1}{2}\) es\[y=\frac{101}{6}\left(x+\frac{1}{2}\right)+\frac{111}{32} .\] Ver Figura 1.8.1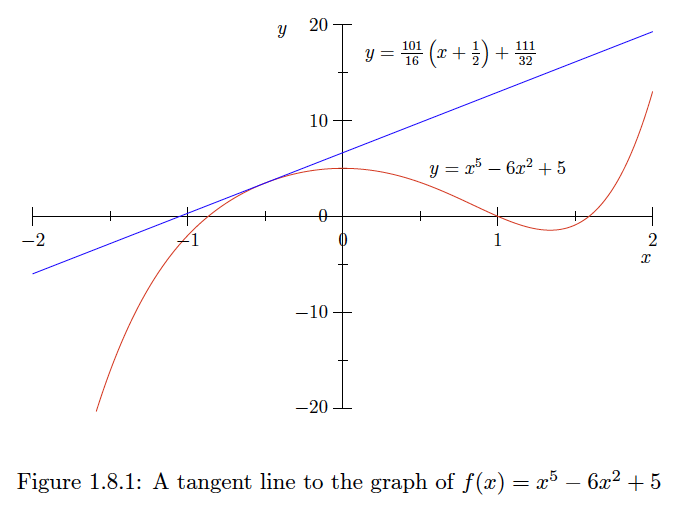
Ejercicio\(\PageIndex{1}\)
Encuentra una ecuación para la línea tangente a la gráfica de
\[f(x)=3 x^{4}-6 x+3\]en\(x=2\).- Contestar
-
\(y=90(x-2)+39\)
Ejercicio\(\PageIndex{2}\)
Encuentra una ecuación para la línea tangente a la gráfica de
\[y=3 \sin ^{2}(x)\]en\(x=\frac{\pi}{4}\).- Contestar
-
\(y=3\left(t-\frac{\pi}{4}\right)+\frac{3}{2}\)


