Search

Text Color

Margin Size

Font Type

Enable Dyslexic Font

10.3: Ejercicios

Última actualización

30 oct 2022
Guardar como PDF
- 10.2: El teorema fundamental del álgebra
- 11: Funciones racionales

Thomas Tradler and Holly Carley
CUNY New York City College of Technology via New York City College of Technology at CUNY Academic Works

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\id}{\mathrm{id}}$ $\newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$ $\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$ $\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\id}{\mathrm{id}}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\kernel}{\mathrm{null}\,}$

$\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$

$\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$

$\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$ $\newcommand{\AA}{\unicode[.8,0]{x212B}}$

$\newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$\newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$\newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vectorC}[1]{\textbf{#1}}$

$\newcommand{\vectorD}[1]{\overrightarrow{#1}}$

$\newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}}$

$\newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}}$

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\avec}{\mathbf a}$

$\newcommand{\bvec}{\mathbf b}$

$\newcommand{\cvec}{\mathbf c}$

$\newcommand{\dvec}{\mathbf d}$

$\newcommand{\dtil}{\widetilde{\mathbf d}}$

$\newcommand{\evec}{\mathbf e}$

$\newcommand{\fvec}{\mathbf f}$

$\newcommand{\nvec}{\mathbf n}$

$\newcommand{\pvec}{\mathbf p}$

$\newcommand{\qvec}{\mathbf q}$

$\newcommand{\svec}{\mathbf s}$

$\newcommand{\tvec}{\mathbf t}$

$\newcommand{\uvec}{\mathbf u}$

$\newcommand{\vvec}{\mathbf v}$

$\newcommand{\wvec}{\mathbf w}$

$\newcommand{\xvec}{\mathbf x}$

$\newcommand{\yvec}{\mathbf y}$

$\newcommand{\zvec}{\mathbf z}$

$\newcommand{\rvec}{\mathbf r}$

$\newcommand{\mvec}{\mathbf m}$

$\newcommand{\zerovec}{\mathbf 0}$

$\newcommand{\onevec}{\mathbf 1}$

$\newcommand{\real}{\mathbb R}$

$\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$

$\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$

$\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$

$\newcommand{\laspan}[1]{\text{Span}\{#1\}}$

$\newcommand{\bcal}{\cal B}$

$\newcommand{\ccal}{\cal C}$

$\newcommand{\scal}{\cal S}$

$\newcommand{\wcal}{\cal W}$

$\newcommand{\ecal}{\cal E}$

$\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$

$\newcommand{\gray}[1]{\color{gray}{#1}}$

$\newcommand{\lgray}[1]{\color{lightgray}{#1}}$

$\newcommand{\rank}{\operatorname{rank}}$

$\newcommand{\row}{\text{Row}}$

$\newcommand{\col}{\text{Col}}$

$\renewcommand{\row}{\text{Row}}$

$\newcommand{\nul}{\text{Nul}}$

$\newcommand{\var}{\text{Var}}$

$\newcommand{\corr}{\text{corr}}$

$\newcommand{\len}[1]{\left|#1\right|}$

$\newcommand{\bbar}{\overline{\bvec}}$

$\newcommand{\bhat}{\widehat{\bvec}}$

$\newcommand{\bperp}{\bvec^\perp}$

$\newcommand{\xhat}{\widehat{\xvec}}$

$\newcommand{\vhat}{\widehat{\vvec}}$

$\newcommand{\uhat}{\widehat{\uvec}}$

$\newcommand{\what}{\widehat{\wvec}}$

$\newcommand{\Sighat}{\widehat{\Sigma}}$

$\newcommand{\lt}{<}$

$\newcommand{\gt}{>}$

$\newcommand{\amp}{&}$

$\definecolor{fillinmathshade}{gray}{0.9}$

Ejercicio $\PageIndex{1}$

Encuentra todas las raíces racionales de $f(x)=2x^3-3x^2-3x+2$ .
Encuentra todas las raíces racionales de $f(x)=3x^3-x^2+15x-5$ .
Encuentra todas las raíces racionales de $f(x)=6x^3+7x^2-11x-12$ .
Encuentra todas las raíces reales de $f(x)=6x^4+25x^3+8x^2-7x-2$ .
Encuentra todas las raíces reales de $f(x)=4x^3+9x^2+26x+6$ .

Contestar

$x=-1, x=2, x=\dfrac{1}{2}$
$x=\dfrac{1}{3}$
$x=\dfrac{-3}{2}, x=-1, x=\dfrac{4}{3},$
$x=\dfrac{1}{2}, x=\dfrac{-2}{3}, x=-2+\sqrt{3}, x=-2-\sqrt{3}$
$x=-\dfrac{1}{4}$

Ejercicio $\PageIndex{2}$

Encuentra una raíz del polinomio adivinando posibles candidatos de la raíz.

$f(x)=x^5-1$
$f(x)=x^4-1$
$f(x)=x^3-27$
$f(x)=x^3+1000$
$f(x)=x^4-81$
$f(x)=x^3-125$
$f(x)=x^{5}+32$
$f(x)=x^{777}-1$
$f(x)=x^2+64$

Contestar

$x = 1$
$x = 1$ o $x = −1$
$x = 3$
$x = −10$
$x = 3$ o $x = −3$
$x = 5$
$x = −2$
$x = 1$
$x = 8i$ o $x = −8i$

Ejercicio $\PageIndex{3}$

Encuentra las raíces del polinomio y úsalo para facturar el polinomio por completo.

$f(x)=x^3-7x+6$
$f(x)=x^3-x^2-16x-20$
$f(x)=x^4-5x^2+4$
$f(x)=x^3+x^2-5x-2$
$f(x)=2x^3+x^2-7x-6$
$f(x)=12x^3+49x^2-2x-24$
$f(x)=x^4-1$
$f(x)=x^5-6x^4+8x^3+6x^2-9x$
$f(x)=x^3-27$
$f(x)=x^4+2x^2-15$

Contestar

$f(x)=(x-2)(x-1)(x+3)$
$f(x)=(x-5)(x+2)^{2}$
$f(x)=(x-1)(x+1)(x-2)(x+2)$
$f(x)=(x-2)\left(x-\dfrac{-3+\sqrt{5}}{2}\right)\left(x-\dfrac{-3-\sqrt{5}}{2}\right)$
$f(x)=2\left(x+\dfrac{3}{2}\right)(x+1)(x-2)$
$f(x)=12\left(x-\dfrac{2}{3}\right)\left(x+\dfrac{3}{4}\right)(x+4)$
$f(x)=(x-1)(x+1)(x-i)(x+i)$
$f(x)=x(x-1)(x+1)(x-3)^{2}$
$f(x)=(x-3)\left(x-\dfrac{-3+3 \sqrt{3} \cdot i}{2}\right)\left(x-\dfrac{-3-3 \sqrt{3} \cdot i}{2}\right)$
$f(x)=(x-\sqrt{3})(x+\sqrt{3})(x-\sqrt{5} \cdot i)(x+\sqrt{5} \cdot i)$

Ejercicio $\PageIndex{4}$

Encuentra las raíces exactas del polinomio; escribe las raíces en forma radical más simple, si es necesario. Esboce una gráfica del polinomio con todas las raíces claramente marcadas.

$f(x)=x^3-2x^2-5x+6$
$f(x)=x^3+5x^2+3x-4$
$f(x)=-x^3+5x^2+7x-35$
$f(x)=x^3+7x^2+13x+7$
$f(x)=2x^3-8x^2-18x-36$
$f(x)=x^4-4x^2+3$
$f(x)=-x^4+x^3+24x^2-4x-80$
$f(x)=7x^3-11x^2-10x+8$
$f(x)=-15x^3+41x^2+15x-9$
$f(x)=x^4-6x^3+6x^2+4x$

Contestar

$clipboard_e9e588e98b98c48bdd3c8195ca7fda394.png$
$clipboard_e574aa6bb305523aeb7a8835375039c1b.png$
$clipboard_e47ad297c187d82fbadad1c9688721edf.png$
$clipboard_e59028f639f84e64c9024e4e4142b2e36.png$
$clipboard_eb5414325803b7b2e37c982f979c093f5.png$
$clipboard_ec5eb742ef834999cda4b878539fb60e7.png$
$clipboard_ec084b5d66298a5770cc7b41c351558fe.png$
$clipboard_e7da7ee7eb592a36fee8cfd42ab317601.png$
$clipboard_e852419d382a7b16d294c52a0e6f3156d.png$
$clipboard_e3b847d557ffa6b84a9439be562ff9f5d.png$

Ejercicio $\PageIndex{5}$

Encuentra un polinomio $f$ que se ajuste a los datos dados.

$f$ tiene grado $3$ . Las raíces de $f$ son precisamente $2$ , $3$ , $4$ . El coeficiente principal de $f$ es $2$ .
$f$ tiene grado $4$ . Las raíces de $f$ son precisamente $-1$ , $2$ , $0$ , $-3$ . El coeficiente principal de $f$ es $-1$ .
$f$ tiene grado $3$ . $f$ tiene raíces $-2$ , $-1$ , $2$ , y $f(0)=10$ .
$f$ tiene grado $4$ . $f$ tiene raíces $0$ , $2$ , $-1$ , $-4$ , y $f(1)=20$ .
$f$ tiene grado $3$ . Los coeficientes de $f$ son todos reales. Las raíces de $f$ son precisamente $2+5i$ , $2-5i$ , $7$ . El coeficiente principal de $f$ es $3$ .
$f$ tiene grado $3$ . Los coeficientes de $f$ son todos reales. $f$ tiene raíces $i$ , $3$ , y $f(0)=6$ .
$f$ tiene grado $4$ . Los coeficientes de $f$ son todos reales. $f$ tiene raíces $5+i$ y $5-i$ de multiplicidad $1$ , la raíz $3$ de la multiplicidad $2$ , y $f(5)=7$ .
$f$ tiene grado $4$ . Los coeficientes de $f$ son todos reales. $f$ tiene raíces $i$ y $3+2i$ .
$f$ tiene grado $6$ . $f$ tiene coeficientes complejos. $f$ tiene raíces $1+i$ , $2+i$ , $4-3i$ de multiplicidad $1$ y raíz $-2$ de multiplicidad $3$ .
$f$ tiene grado $5$ . $f$ tiene coeficientes complejos. $f$ tiene raíces $i$ , $3$ , $-7$ (y posiblemente otras raíces).
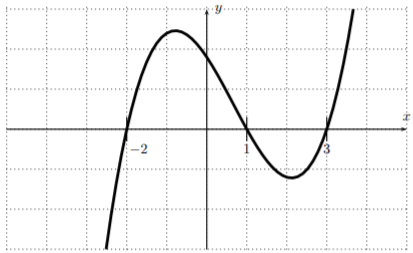
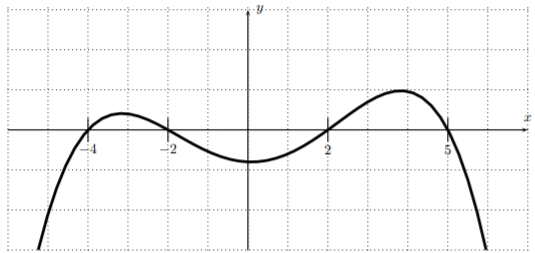
$f$ tiene grado $3$ . Las raíces de $f$ están determinadas por su gráfica:

$clipboard_ed02f942d9bdafc3eedf9e98f36a5ac5d.png$

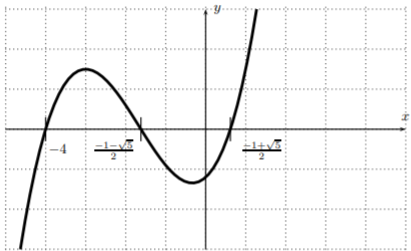
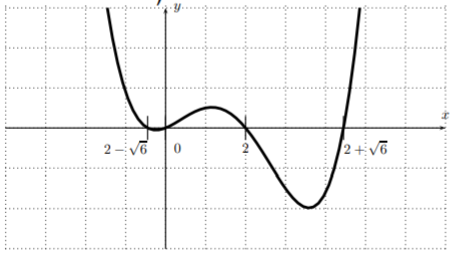
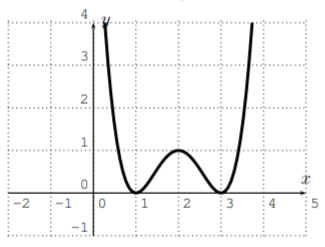
$f$ tiene grado $4$ . Los coeficientes de $f$ son todos reales. El coeficiente principal de $f$ es 1. Las raíces de $f$ están determinadas por su gráfica: (ver Sección 9.3).

$clipboard_e627fb6f60fa1dd609266bab42e4f6a6c.png$

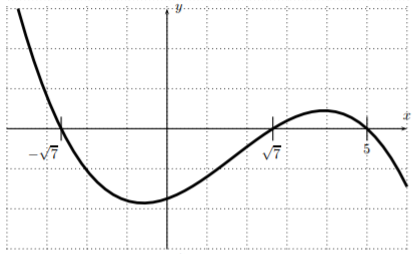
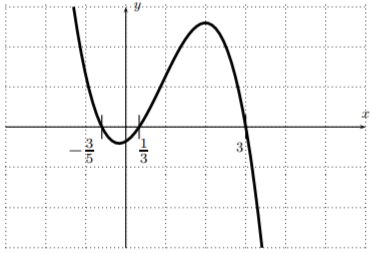
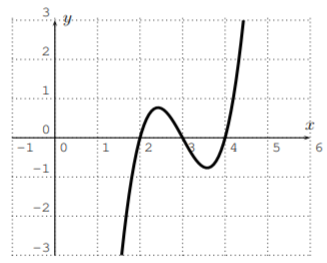
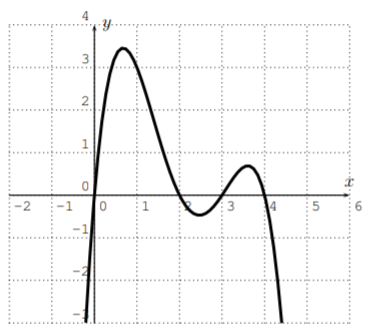
$f$ tiene grado $4$ . Los coeficientes de $f$ son todos reales. $f$ tiene la siguiente gráfica:

$clipboard_e845de0dad18ac50917a919fc0918c97a.png$

Contestar

$f(x)=2(x-2)(x-3)(x-4)$
$f(x)=(-1) \cdot x(x-2)(x+1)(x+3)$
$f(x)=\left(-\dfrac{5}{2}\right) \cdot(x-2)(x+2)(x+1)$
$f(x)=-2 \cdot x(x-2)(x+1)(x+4)$
$f(x)=3(x-7)(x-(2+5 i))(x-(2-5 i))$
$f(x)=(-2) \cdot(x-i)(x+i)(x-3)$
$f(x)=\frac{7}{4} \cdot(x-(5+i))(x-(5-i))(x-3)^{2}$
$f(x)=(x-i)(x+i)(x-(3+2 i))(x-(3-2 i))$ (otras respuestas correctas son posibles, dependiendo de la elección del primer coeficiente)
$f(x)=(x-(1+i))(x-(2+i))(x-(4-3 i))(x+2)^{3}$ (otras respuestas correctas son posibles, dependiendo de la elección del primer coeficiente)
$f(x)=(x-i)(x-3)(x+7)^{2}$ (otras respuestas correctas son posibles, dependiendo de la elección del primer coeficiente y la cuarta raíz)
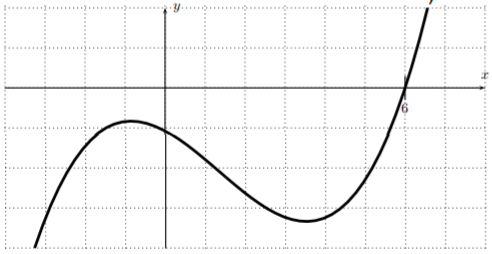
$f(x)=(x-2)(x-3)(x-4)$ (otras respuestas correctas son posibles, dependiendo de la elección del primer coeficiente)
$f(x)=(x-1)^{2}(x-3)^{2}$
$f(x)=-x(x-1)(x-3)(x-4)$ (otras respuestas correctas son posibles, dependiendo de la elección del primer coeficiente)

Ejercicio10.3.1\PageIndex{1}

Ejercicio10.3.2\PageIndex{2}

Ejercicio10.3.3\PageIndex{3}

Ejercicio10.3.4\PageIndex{4}

Ejercicio10.3.5\PageIndex{5}

Support Center

How can we help?

Ejercicio $\PageIndex{1}$

Ejercicio $\PageIndex{2}$

Ejercicio $\PageIndex{3}$

Ejercicio $\PageIndex{4}$

Ejercicio $\PageIndex{5}$