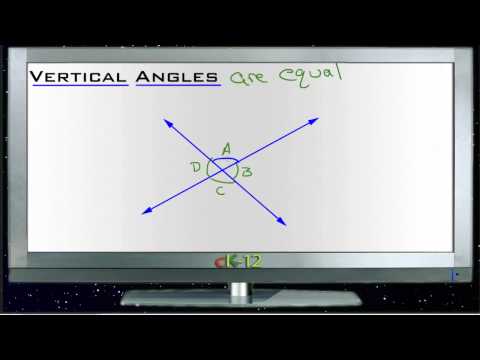
1.17: Ángulos Verticales

Última actualización
Guardar como PDF

Page ID: 107640

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

Entender que los ángulos verticales son opuestos entre sí y tienen la misma medida.

Los ángulos verticales son dos ángulos no adyacentes formados por líneas que se cruzan. \(\angle 1\)y\(\angle 3\) son ángulos verticales y\(\angle 2\) y\(\angle 4\) son ángulos verticales.

f-d_b1875593889aa9f44b649a8d51e5074fe39af4579f8553e621d7efc5+image_tiny+image_tiny.png — Figura\(\PageIndex{1}\)

El Teorema de Ángulos Verticales establece que si dos ángulos son ángulos verticales, entonces son congruentes.

¿Y si te dieran dos ángulos de tamaño desconocido y te dijeran que son ángulos verticales? ¿Cómo determinarías sus medidas de ángulo?

Ejemplo\(\PageIndex{1}\)

Encuentra el valor de\(x\).

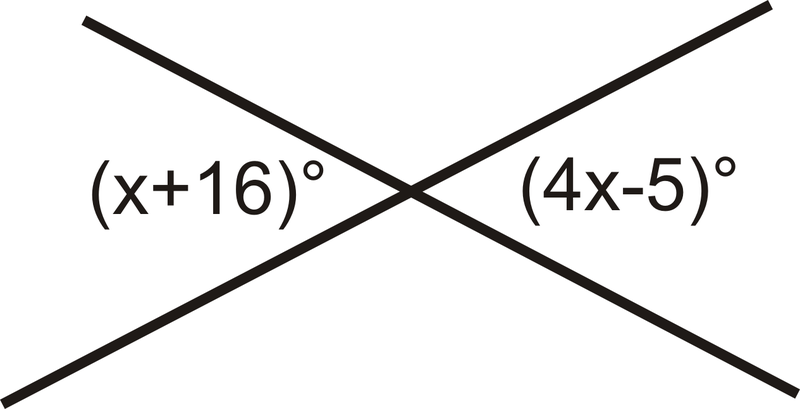f-d_cb1114c5a2bf758f3c020ca1dde1ff2034d4fe359c434e1847025242+image_tiny+image_tiny.png — Figura\(\PageIndex{2}\)

Solución

Los ángulos verticales son congruentes, así que establece los ángulos iguales entre sí y resuelve para\(x\).

\(x+16=4x−5\)

\(3x=21\)

\(x=7^{\circ}\)

Ejemplo\(\PageIndex{2}\)

Encuentra el valor de\(y\).

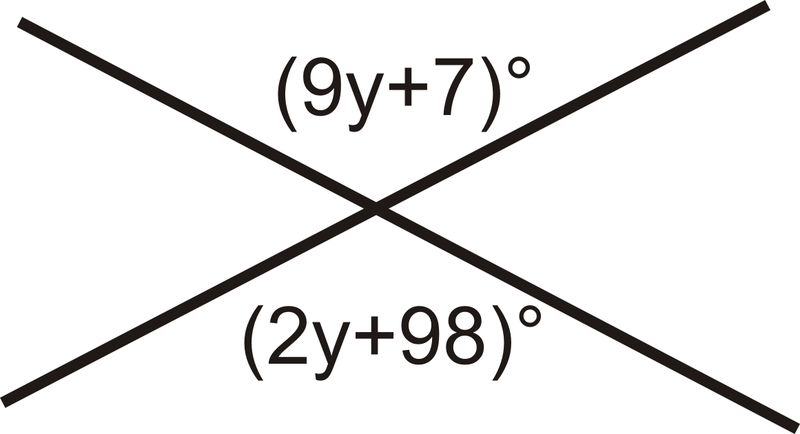f-d_c192cc4f78143e455258c341d97e20686e7cf12a5ec2c5465506cf7+image_tiny+image_tiny.png — Figura\(\PageIndex{3}\)

Solución

Los ángulos verticales son congruentes, así que establece los ángulos iguales entre sí y resuelve para\(y\).

\(9y+7=2y+98\)

\(7y=91\)

\(y=13^{\circ}\)

Ejemplo\(\PageIndex{3}\)

Encuentra\(m\angle 1\).

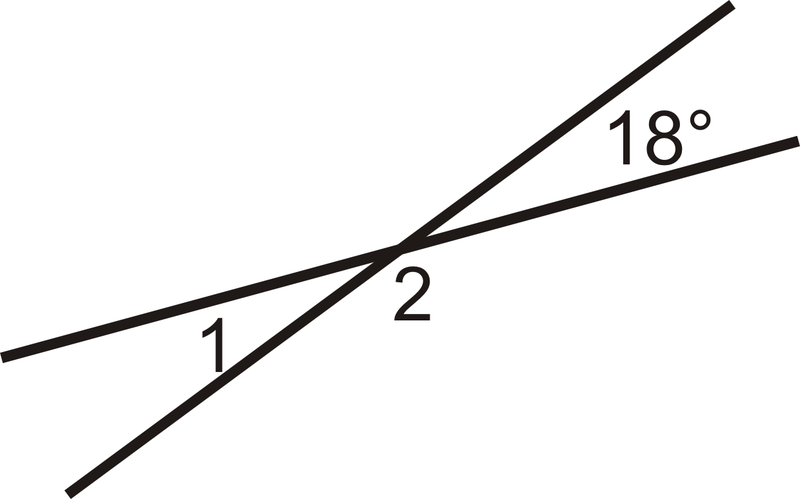F-D_450f99639ceaa9618ed92b9d2c1d0b0f7d5fc39640a56acdf0957a3d+image_tiny+image_tiny.png — Figura\(\PageIndex{4}\)

Solución

\(\angle 1\)es ángulos verticales con\(18^{\circ}\), entonces\ (m\ ángulo 1=18^ {\ circ}.

Ejemplo\(\PageIndex{4}\)

Si\ ángulo ABC y\ ángulo DEF son ángulos verticales y\ (m\ ángulo ABC= (4x+10) ^ {\ circ} y\ (m\ ángulo DEF= (5x+2) ^ {\ circ}, ¿cuál es la medida de cada ángulo?

Solución

Los ángulos verticales son congruentes, así que establece los ángulos iguales entre sí y resuelve para x Luego regresa para encontrar la medida de cada ángulo.

\(4x+10=5x+2\)

\(x=8\)

Entonces,\(m\angle ABC=m\angle DEF=(4(8)+10)^{\circ}=42^{\circ}\)

Ejemplo\(\PageIndex{5}\)

Verdadero o falso: los ángulos verticales son siempre menores de 90^ {\ circ}.

Solución

Esto es falso, puedes tener ángulos verticales que son más de 90^ {\ circ}. Los ángulos verticales son menores de 180^ {\ circ}.

Revisar

Usa el diagrama a continuación para los ejercicios 1-2. Tenga en cuenta que\(\overline{NK} \perp \overleftrightarrow{IL}\).

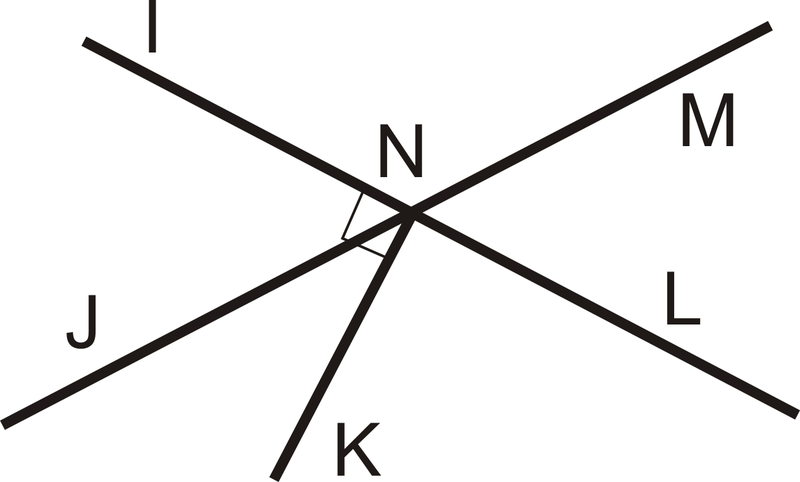f-d_ddbfde705abde0cdc132cdfcd782bec102f8b34c4b23a3ab4f4862ca+image_tiny+image_tiny.png — Figura\(\PageIndex{5}\)

Nombra un par de ángulos verticales.

Si\(m\angle INJ=63^{\circ}\), encuentra\(m\angle MNL\).

Para el ejercicio 3, determinar si la declaración es verdadera o falsa.

Los ángulos verticales tienen el mismo vértice.

Si\(\angle ABC\) y\(\angle DEF\) son ángulos verticales y\(m\angle ABC=(9x+1)^{\circ}\) y\(m\angle DEF=(5x+29)^{\circ}\), ¿cuál es la medida de cada ángulo?
Si\(\angle ABC\) y\(\angle DEF\) son ángulos verticales y\(m\angle ABC=(8x+2)^{\circ}\) y\(m\angle DEF=(2x+32)^{\circ}\), ¿cuál es la medida de cada ángulo?
Si\(\angle ABC\) y\(\angle DEF\) son ángulos verticales y\(m\angle ABC=(x+22)^{\circ}\) y\(m\angle DEF=(5x+2)^{\circ}\), ¿cuál es la medida de cada ángulo?
Si\(\angle ABC\) y\(\angle DEF\) son ángulos verticales y\(m\angle ABC=(3x+12)^{\circ}\) y\(m\angle DEF=(7x)^{\circ}\), ¿cuál es la medida de cada ángulo?
Si\(\angle ABC\) y\(\angle DEF\) son ángulos verticales y\(m\angle ABC=(5x+2)^{\circ}\) y\(m\angle DEF=(x+26)^{\circ}\), ¿cuál es la medida de cada ángulo?
Si\(\angle ABC\) y\(\angle DEF\) son ángulos verticales y\(m\angle ABC=(3x+1)^{\circ}\) y\(m\angle DEF=(2x+2)^{\circ}\), ¿cuál es la medida de cada ángulo?
Si\(\angle ABC\) y\(\angle DEF\) son ángulos verticales y\(m\angle ABC=(6x−3)^{\circ}\) y\(m\angle DEF=(5x+1)^{\circ}\), ¿cuál es la medida de cada ángulo?

Reseña (Respuestas)

Para ver las respuestas de Revisar, abra este archivo PDF y busque la sección 1.10.

Recursos

El vocabulario

Término	Definición
Ángulos Verticales	Los ángulos verticales son un par de ángulos opuestos creados por líneas que se cruzan.
Teorema de ángulos verticales	El Teorema de Ángulos Verticales establece que si dos ángulos son verticales, entonces son congruentes.

Recursos adicionales

Elemento Interactivo

Video: Ángulos complementarios, suplementarios y verticales

Actividades: Preguntas de discusión sobre ángulos verticales

Ayudas de estudio: Guía de estudio de ángulos

Práctica: Ángulos verticales

Mundo Real: Ángulos Verticales