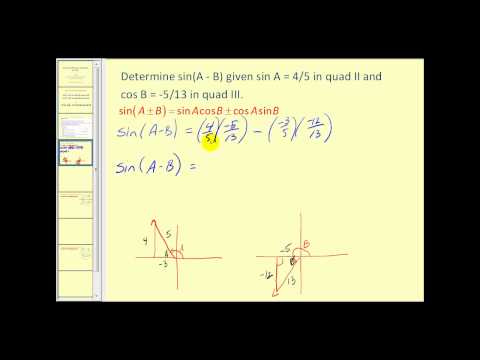
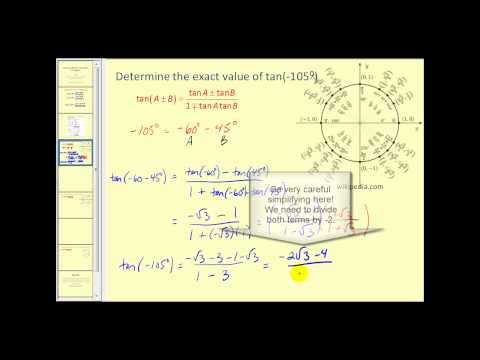
6.3: Suma y Diferencia de Identidades

Con tu conocimiento de ángulos especiales como el seno y el coseno de$30^{\circ}$ y$45^{\circ}$, puedes encontrar el seno y el coseno de$15^{\circ}$, la diferencia de$45^{\circ}$ y$30^{\circ}$, y$75^{\circ}$, la suma de$45^{\circ}$ y$30^{\circ}$. Usando lo
que sabes sobre el círculo unitario y las identidades de suma y diferencia, ¿cómo determinas$\sin 15^{\circ}$ y$\sin 75^{\circ}$?

Identidades de suma y diferencia

Primer vistazo a la derivación de la identidad de diferencia de coseno:

$\cos (\alpha-\beta)=\cos \alpha \cos \beta+\sin \alpha \sin \beta$

Comience dibujando dos ángulos arbitrarios$\alpha$ y$\beta$. En la imagen de arriba$\alpha$ está el ángulo en rojo y$\beta$ es el ángulo en azul. La diferencia$\alpha-\beta$ se anota en negro como$\theta$. La razón por la que hay dos imágenes es porque la imagen de la derecha tiene el mismo ángulo$\theta$ en una posición girada. Esto será útil para trabajar porque la longitud de$\overline{A B}$ será la misma que la longitud de$\overline{C D}$.

$\begin{aligned} \overline{A B} &=\overline{C D} \\ \sqrt{(\cos \alpha-\cos \beta)^{2}+(\sin \alpha-\sin \beta)^{2}} &=\sqrt{(\cos \theta-1)^{2}+(\sin \theta-0)^{2}} \\(\cos \alpha-\cos \beta)^{2}+(\sin \alpha-\sin \beta)^{2} &=(\cos \theta-1)^{2}+(\sin \theta-0)^{2} \end{aligned}$

$(\cos \alpha)^{2}-2 \cos \alpha \cos \beta+(\cos \beta)^{2}+(\sin \alpha)^{2}-2 \sin \alpha \sin \beta+(\sin \beta)^{2}=(\cos \theta-1)^{2}+(\sin \theta)^{2}$

$\begin{aligned} 2-2 \cos \alpha \cos \beta-2 \sin \alpha \sin \beta &=(\cos \theta)^{2}-2 \cos \theta+1+(\sin \theta)^{2} \\ 2-2 \cos \alpha \cos \beta-2 \sin \alpha \sin \beta &=1-2 \cos \theta+1 \\-2 \cos \alpha \cos \beta-2 \sin \alpha \sin \beta &=-2 \cos \theta \\ \cos \alpha \cos \beta+\sin \alpha \sin \beta &=\cos \theta \\ &=\cos (\alpha-\beta) \end{aligned}$

Se puede utilizar esta identidad para acreditar el coseno de una identidad suma. Primero, comenzar con el coseno de una diferencia y hacer una sustitución. Entonces usa la identidad par/impares.

$\cos \alpha \cos \beta+\sin \alpha \sin \beta=\cos (\alpha-\beta)$

Let$\gamma=-\beta$

$\cos \alpha \cos (-\gamma)+\sin \alpha \sin (-\gamma)=\cos (\alpha+\gamma)$
$\cos \alpha \cos \gamma-\sin \alpha \sin \gamma=\cos (\alpha+\gamma)$

Las pruebas para seno y tangente se dejan a los videos y ejemplos. Se enumeran aquí para su referencia. Se excluyen cotangente, secante y cosecante porque puedes usar identidades recíprocas para obtenerlas una vez que tienes seno, coseno y tangente.

Resumen

• $\cos (\alpha \pm \beta)=\cos \alpha \cos \beta \mp \sin \alpha \sin \beta$
• $\sin (\alpha \pm \beta)=\sin \alpha \cos \beta \pm \cos \alpha \sin \beta$
• $\tan (\alpha \pm \beta)=\frac{\sin (\alpha \pm \beta)}{\cos (\alpha \pm \beta)}=\frac{\tan \alpha \pm \tan \beta}{1 \mp \tan \alpha \tan \beta}$

El orden de los signos más o menos es importante porque para el coseno de una suma, el signo negativo se usa en el otro lado de la identidad. Esto es lo opuesto al seno de una suma, donde se usa un signo positivo al otro lado de la identidad.

Ejemplos

Ejemplo 1

Anteriormente, se le pidió que evaluara$\sin 15^{\circ}$ y$\sin 75^{\circ}$ exactamente sin calculadora. Para ello es necesario utilizar el seno de una diferencia y el seno de una suma.
\ (
\ begin {array} {l}
\ sin\ left (45^ {\ circ} -30^ {\ circ}\ derecha) =\ sin 45^ {\ circ}\ cos 30^ {\ circ} -\ cos 45^ {\ circ}\ sin 30^ {\ circ} =\ frac {\ sqrt {2}} {2}\ cdot\ frac {\ sqrt {2}} {2}\ cdot\ frac {\ sqrt {2}} {3}} {2} -\ frac {\ sqrt {2}} {2}\ cdot\ frac {1} {2} =\ frac {\ sqrt {6} -\ sqrt {2}} {4}\
\\ sin\ izquierda (45^ {\ circ} +30^ {\ circ}\ derecha) =\ sin 45^ {\ circ}\ cos 30^ {\ circ} +\ cos 45^ {\ circ}\ sin 30^ {\ circ} =\ frac {\ sqrt {2}} {2}\ cdot\ frac {\ sqrt {3}} {2} +\ frac {\ sqrt {2}}} {2}\ cdot\ frac {1} {2} =\ frac {\ sqrt {6} +\ sqrt {2}} {4}
\ end {array}
\)

Ejemplo 2

Encuentra el valor exacto de$\tan 15^{\circ}$ sin usar una calculadora.

$\tan 15^{\circ}=\tan \left(45^{\circ}-30^{\circ}\right)=\frac{\tan 45^{\circ}-\tan 30^{\circ}}{1+\tan 45^{\circ} \tan 30^{\circ}}=\frac{1-\frac{\sqrt{3}}{3}}{1+1 \cdot \frac{\sqrt{3}}{3}}=\frac{3-\sqrt{3}}{3+\sqrt{3}}$

Una solución final no tendrá un radical en el denominador. En este caso multiplicar por el conjugado del denominador eliminará al radical.

$=\frac{(3-\sqrt{3}) \cdot(3-\sqrt{3})}{(3+\sqrt{3}) \cdot(3-\sqrt{3})}$
$=\frac{(3-\sqrt{3})^{2}}{9-3}$
$=\frac{(3-\sqrt{3})^{2}}{6}$

Ejemplo 3

Evalúe la expresión exactamente sin usar una calculadora.

$\cos 50^{\circ} \cos 5^{\circ}+\sin 50^{\circ} \sin 5^{\circ}$

Una vez que conozcas la forma general de las identidades de suma y diferencia, entonces reconocerás esto como coseno de una diferencia.

$\cos 50^{\circ} \cos 5^{\circ}+\sin 50^{\circ} \sin 5^{\circ}=\cos \left(50^{\circ}-5^{\circ}\right)=\cos 45^{\circ}=\frac{\sqrt{2}}{2}$

Ejemplo 4

Utilice una identidad de suma o diferencia para encontrar un valor exacto de$\cot \left(\frac{5 \pi}{12}\right)$.

Comienza con la definición de cotangente como la inversa de tangente.

$\begin{aligned} \cot \left(\frac{5 \pi}{12}\right) &=\frac{1}{\tan \left(\frac{5 \pi}{12}\right)} \\ &=\frac{1}{\tan \left(\frac{9 \pi}{12}-\frac{4 \pi}{12}\right)} \\ &=\frac{1}{\tan \left(135^{\circ}-60^{\circ}\right)} \\ &=\frac{1+\tan 135^{\circ} \tan 60^{\circ}}{\tan 135^{\circ}-\tan 60^{\circ}} \\ &=\frac{1+(-1) \cdot \sqrt{3}}{(-1)-\sqrt{3}} \\ &=\frac{(1-\sqrt{3})}{(-1-\sqrt{3})} \\ &=\frac{(1-\sqrt{3})^{2}}{(-1+\sqrt{3}) \cdot(1-\sqrt{3})} \\ &=\frac{(1-\sqrt{3})^{2}}{-(1-3)} \\ &=\frac{(1-\sqrt{3})^{2}}{2} \end{aligned}$

Ejemplo 5

Demostrar la siguiente identidad:

$\frac{\sin (x-y)}{\sin (x+y)}=\frac{\tan x-\tan y}{\tan x+\tan y}$

Estos son los pasos:

$\begin{aligned} \frac{\sin (x-y)}{\sin (x+y)} &=\frac{\tan x-\tan y}{\tan x+\tan y} \\ \frac{\sin x \cos y-\cos x \sin y}{\sin x \cos y+\cos x \sin y} &=\\ \frac{\sin x \cos y-\cos x \sin y}{\sin x \cos y+\cos x \sin y} \cdot \frac{\left(\frac{1}{\cos x \cdot \cos y}\right)}{\left(\frac{1}{\cos x \cdot \cos y}\right)} &=\\ \frac{\left(\frac{\sin x}{\cos x \cdot \cos y}\right)-\left(\frac{\cos x \sin y}{\cos x \cdot \cos y}\right)}{\left(\frac{\sin x \cos x}{\cos x \cdot \cos y}\right)+\left(\frac{\cos x}{\cos x \cdot \cos y}\right)} &=\\ \frac{\tan x-\tan y}{\tan x+\tan y} &=\end{aligned}$

Revisar

Encuentra el valor exacto para cada expresión usando una identidad de suma o diferencia.

1. $\cos 75^{\circ}$

2. $\cos 105^{\circ}$

3. $\cos 165^{\circ}$

4. $\sin 105^{\circ}$

5. $\sec 105^{\circ}$

6. $\tan 75^{\circ}$

7. Demostrar el seno de una identidad suma.

8. Demostrar la tangente de una identidad de suma.

9. Demostrar la tangente de una identidad de diferencia.

10. Evaluar sin calculadora:$\cos 50^{\circ} \cos 10^{\circ}-\sin 50^{\circ} \sin 10^{\circ}$.

11. Evaluar sin calculadora:$\sin 35^{\circ} \cos 5^{\circ}-\cos 35^{\circ} \sin 5^{\circ}$.

12. Evaluar sin calculadora:$\sin 55^{\circ} \cos 5^{\circ}+\cos 55^{\circ} \sin 5^{\circ}$.

13. Si$\cos \alpha \cos \beta=\sin \alpha \sin \beta,$ entonces, ¿qué es lo que$\cos (\alpha+\beta)$ equivale?

14. Demostrar que$\tan \left(x+\frac{\pi}{4}\right)=\frac{1+\tan x}{1-\tan x}$

15. $\sin (x+\pi)=-\sin x$Demuéstralo.