8.E: Secciones Cónicas (Ejercicios)
( \newcommand{\kernel}{\mathrm{null}\,}\)
Ejercicio8.E.1
Calcular la distancia y el punto medio entre los dos puntos dados.
- (0,2)y(−4,−1)
- (6,0)y(−2,−6)
- (−2,4)y(−6,−8)
- (12,−1)y(52,−12)
- (0,−3√2)y(√5,−4√2)
- (−5√3,√6)y(−3√3,√6)
- Contestar
-
1. Distancia:5 unidades; punto medio:(−2,12)
3. Distancia:4√10 unidades; punto medio:(−4,−2)
5. Distancia:√7 unidades; punto medio:(√52,−7√22)
Ejercicio8.E.2
Determinar el área de un círculo cuyo diámetro está definido por los dos puntos dados.
- (−3,3)y(3,−3)
- (−2,−9)y(−10,−15)
- (23,−12)y(−13,32)
- (2√5,−2√2)y(0,−4√2)
- Contestar
-
1. 18πunidades cuadradas
3. 5π4unidades cuadradas
Ejercicio8.E.3
Reescribe en forma estándar y da el vértice.
- y=x2−10x+33
- y=2x2−4x−1
- y=x2−3x−1
- y=−x2−x−2
- x=y2+10y+10
- x=3y2+12y+7
- x=−y2+8y−3
- x=5y2−5y+2
- Contestar
-
1. y=(x−5)2+8;vértice:(5,8)
3. y=(x−32)2−134;vértice:(32,−134)
5. x=(y+5)2−15;vértice:(−15,−5)
7. x=−(y−4)2+13;vértice:(13,4)
Ejercicio8.E.4
Reescribir en forma estándar y gráfica. Asegúrate de encontrar el vértice y todas las intercepciones.
- y=x2−20x+75
- y=−x2−10x+75
- y=−2x2−12x−24
- y=4x2+4x+6
- x=y2−10y+16
- x=−y2+4y+12
- x=−4y2+12y
- x=9y2+18y+12
- x=−4y2+4y+2
- x=−y2−5y+2
- Contestar
-
1. y=(x−10)2−25;
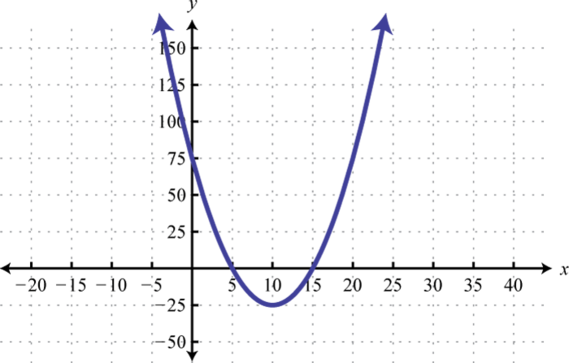
Figura 8.E.1 3. y=−2(x+3)2−6;
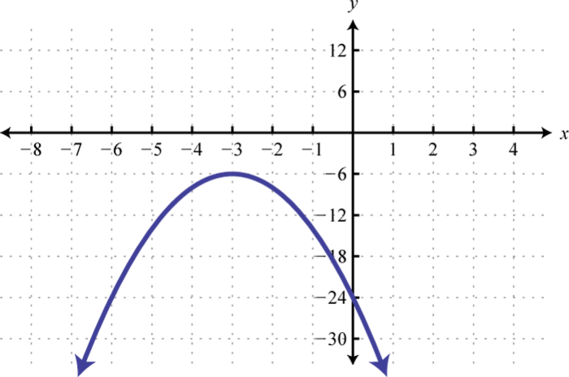
Figura 8.E.2 5. x=(y−5)2−9;

Figura 8.E.3 7. x=−4(y−32)2+9;
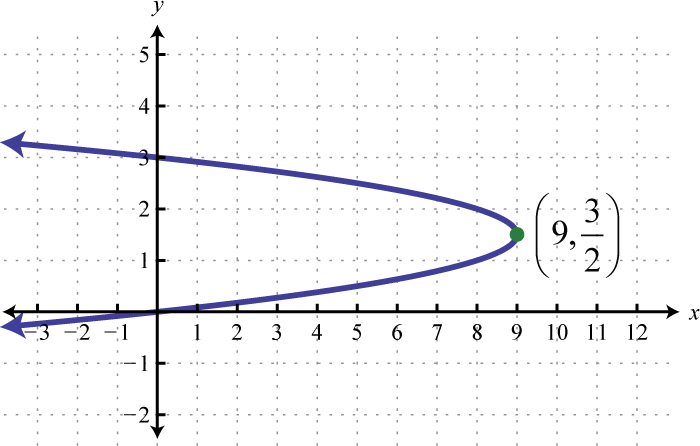
Figura 8.E.4 9. x=−4(y−12)2+3;
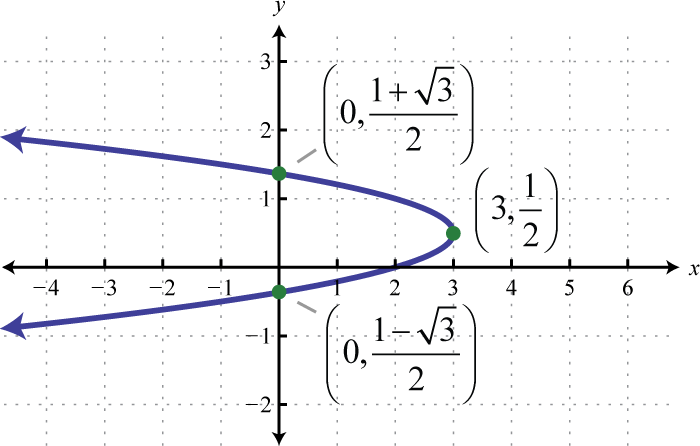
Figura 8.E.5
Ejercicio8.E.5
Determinar el centro y el radio dada la ecuación de un círculo en forma estándar.
- (x−6)2+y2=9
- (x+8)2+(y−10)2=1
- x2+y2=5
- (x−38)2+(y+52)2=12
- Contestar
-
1. Centro:(6,0); radio:r=3
3. Centro:(0,0); radio:r=√5
Ejercicio8.E.6
Determine la forma estándar para la ecuación del círculo:
- Centro(−7,2) con radior=10
- Centro(13,−1) con radior=23
- Centro(0,−5) con radior=2√7
- Centro(1,0) con radior=5√32
- Círculo cuyo diámetro está definido por(−4,10) y(−2,8)
- Círculo cuyo diámetro está definido por(3,−6) y(0,−4)
- Contestar
-
1. (x+7)2+(y−2)2=100
3. x2+(y+5)2=28
5. (x+3)2+(y−9)2=2
Ejercicio8.E.7
Encuentra losx - yy -interceptos.
- (x−3)2+(y+5)2=16
- (x+5)2+(y−1)2=4
- x2+(y−2)2=20
- (x−3)2+(y+3)2=8
- x2+y2−12y+27=0
- x2+y2−4x+2y+1=0
- Contestar
-
1. x-intercepta: ninguno;y -intercepta:(0,−5±√7)
3. x-intercepta:(±4,0);y -intercepta:(0,2±2√5)
5. x-intercepta: ninguno;y -intercepta:(0,3),(0,9)
Ejercicio8.E.8
Gráfica.
- (x+8)2+(y−6)2=4
- (x−20)2+(y+152)2=2254
- x2+y2=24
- (x−1)2+y2=14
- x2+(y−7)2=27
- (x+1)2+(y−1)2=2
- Contestar
-
1.

Figura 8.E.6 3.
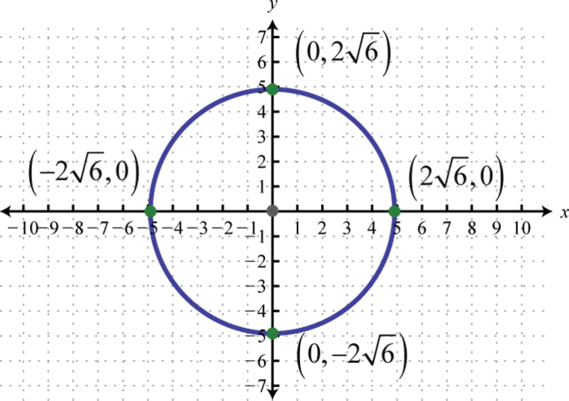
Figura 8.E.7 5.

Figura 8.E.8
Ejercicio8.E.9
Reescribir en forma estándar y gráfica.
- x2+y2−6x+4y−3=0
- x2+y2+8x−10y+16=0
- 2x2+2y2−2x−6y−3=0
- 4x2+4y2+8y+1=0
- x2+y2−5x+y−12=0
- x2+y2+12x−8y=0
- Contestar
-
1. (x−3)2+(y+2)2=16;
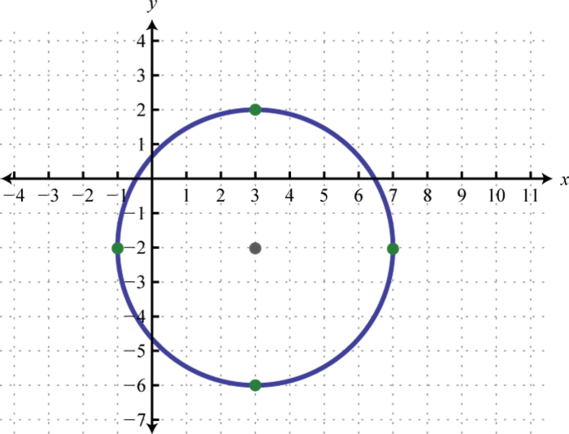
Figura 8.E.9 3. (x−12)2+(y−32)2=4;
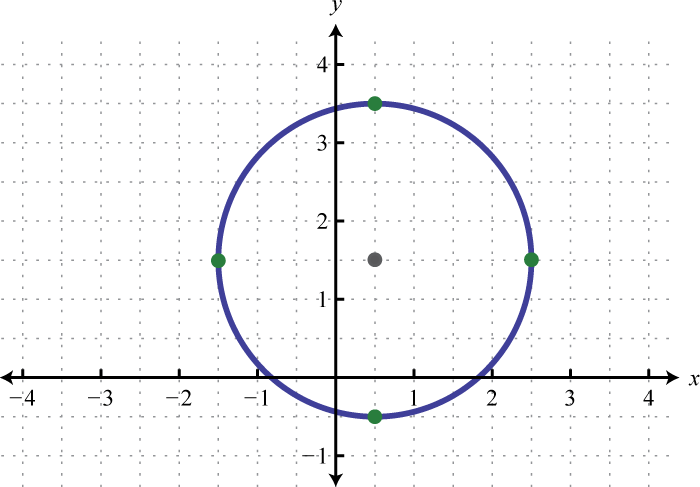
Figura 8.E.10 5. (x−52)2+(y+12)2=7;
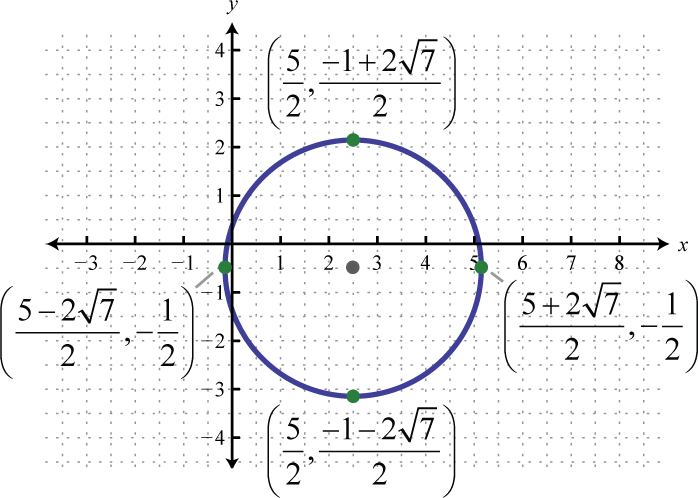
Figura 8.E.11
Ejercicio8.E.10
Dada la ecuación de una elipse en forma estándar, determine su centro, orientación, radio mayor y radio menor.
- (x+12)216+(y−10)24=1
- (x+3)23+y225=1
- x2+(y−5)212=1
- (x−8)25+(y+8)18=1
- Contestar
-
1. Centro:(−12,10); orientación: horizontal; radio mayor:4 unidades; radio menor:2 unidades
3. Centro:(0,5); orientación: vertical; radio mayor:2√3 unidades; radio menor:1 unidad
Ejercicio8.E.11
Determinar la forma estándar para la ecuación de la elipse dada la siguiente información.
- Centro(0,−4) cona=3 yb=4
- Centro(3,8) cona=1 yb=√7
- Centro(0,0) cona=5 yb=√2
- Centro(−10,−30) cona=10 yb=1
- Contestar
-
1. x29+(y+4)216=1
3. x225+y22=1
Ejercicio8.E.12
Encuentra losx - yy -interceptos.
- (x+2)24+y29=1
- (x−1)22+(y+1)23=1
- 5x2+2y2=20
- 5(x−3)2+6y2=120
- Contestar
-
1. x-intercepta:(−4,0),(0,0);y -intercepta:(0,0)
3. x-intercepta:(±2,0);y -intercepta:(0,±√10)
Ejercicio8.E.13
Gráfica.
- (x−10)225+(y+5)24=1
- (x+6)29+(y−8)236=1
- (x−32)24+(y−72)2=1
- (x−23)2+y24=1
- x22+y25=1
- (x+2)28+(y−3)212=1
- Contestar
-
1.
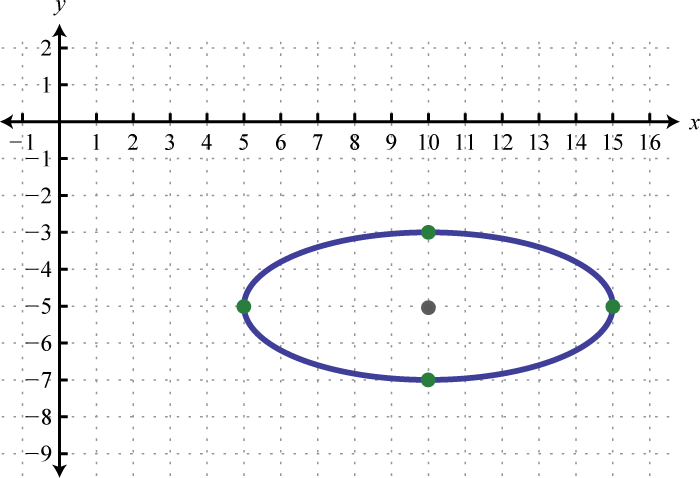
Figura 8.E.12 3.

Figura 8.E.13 5.
Figura 8.E.14
Ejercicio8.E.14
Reescribir en forma estándar y gráfica.
- 4x2+9y2−8x+90y+193=0
- 9x2+4y2+108x−80y+580=0
- x2+9y2+6x+108y+324=0
- 25x2+y2−350x−8y+1,216=0
- 8x2+12y2−16x−36y−13=0
- 10x2+2y2−50x+14y+7=0
- Contestar
-
1. (x−1)29+(y+5)24=1;

Figura 8.E.15 3. (x+3)29+(y+6)2=1;
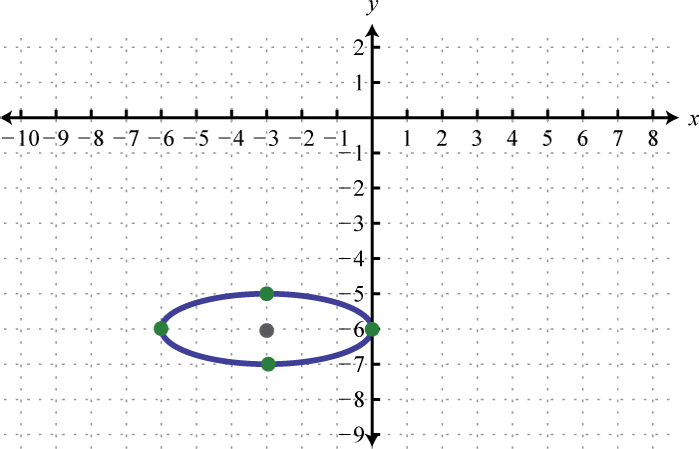
Figura 8.E.16 5. (x−1)26+(y−32)24=1;

Figura 8.E.17
Ejercicio8.E.15
Dada la ecuación de una hipérbola en forma estándar, determinar su centro, en qué dirección se abre la gráfica, y los vértices.
- (x−10)24−(y+5)216=1
- (x+7)22−(y−8)28=1
- (y−20)23−(x−15)2=1
- 3y2−12(x−1)2=36
- Contestar
-
1. Centro:(10,−5); abre izquierda y derecha; vértices:(8,−5),(12,−5)
3. Centro:(15,20); se abre hacia arriba y hacia abajo; vértices:(15,20−√3),(15,20+√3)
Ejercicio8.E.16
Determinar la forma estándar para la ecuación de la hipérbola.
- El centro(−25,10),a=3,b=√5, se abre hacia arriba y hacia abajo.
- El centro(9,−12),a=5√3,b=7, se abre a izquierda y derecha.
- El centro(−4,0),a=1,b=6, se abre a izquierda y derecha.
- El centro(−2,−3),a=10√2,b=2√3, se abre hacia arriba y hacia abajo.
- Contestar
-
1. (y−10)25−(x+25)29=1
3. (x+4)2−y236=1
Ejercicio8.E.17
Encuentra losx - yy -interceptos.
- (x−1)24−(y+3)29=1
- (x+4)28−(y−2)212=1
- 4(y−2)2−x2=16
- 6(y+1)2−3(x−1)2=18
- Contestar
-
1. x-intercepta:(1±2√2,0);y -intercepta: ninguna
3. x-intercepta:(0,0);y -intercepta:(0,0),(0,4)
Ejercicio8.E.18
Gráfica.
- (x−10)225−(y+5)2100=1
- (x−4)24−(y−8)216=1
- (y−3)29−(x−6)281=1
- (y+1)24−(x+1)225=1
- y227−(x−3)29=1
- x22−y23=1
- Contestar
-
1.
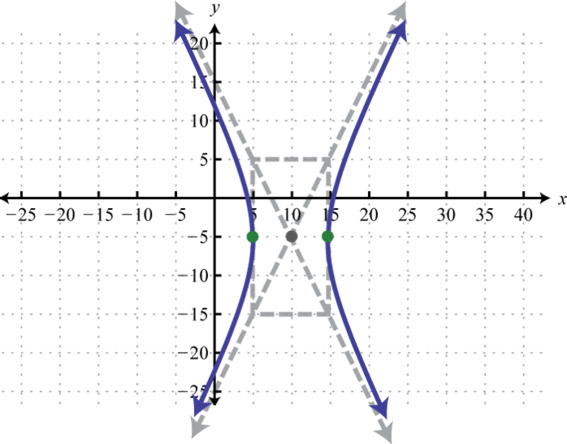
Figura 8.E.18 3.
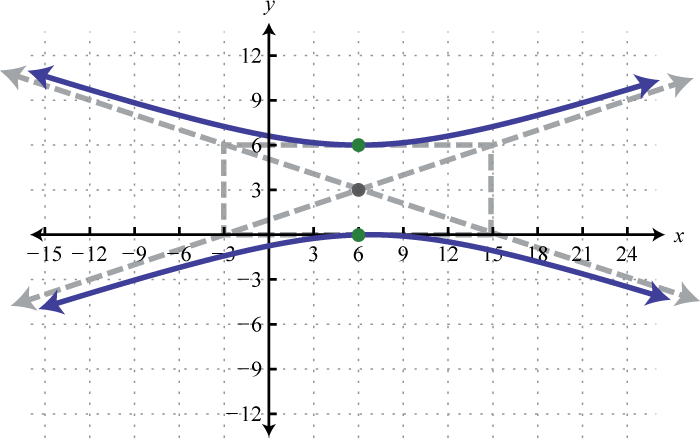
Figura 8.E.19 5.

Figura 8.E.20
Ejercicio8.E.19
Reescribir en forma estándar y gráfica.
- 4x2−9y2−8x−90y−257=0
- 9x2−y2−108x+16y+224=0
- 25y2−2x2−100y+50=0
- 3y2−x2−2x−10=0
- 8y2−12x2+24y−12x−33=0
- 4y2−4x2−16y−28x−37=0
- Contestar
-
1. (x−1)29−(y+5)24=1;
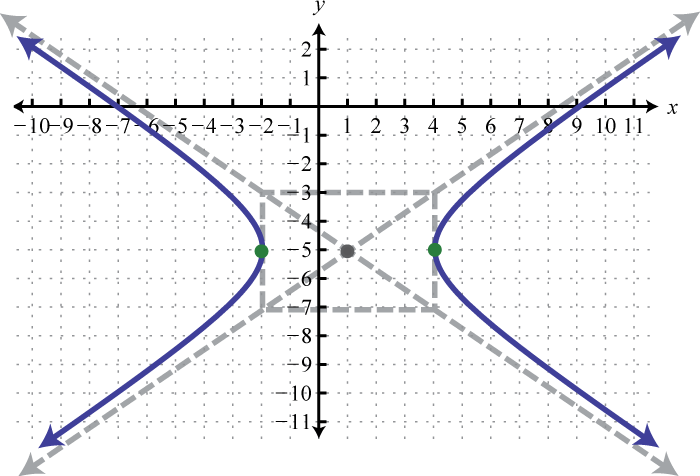
Figura 8.E.21 3. (y−2)22−x225=1;
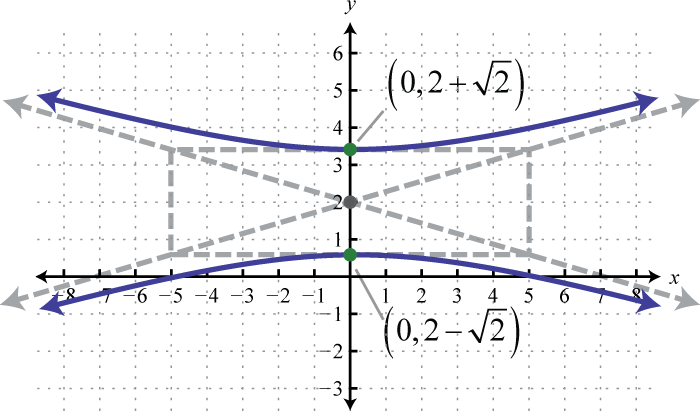
Figura 8.E.22 5. (y+32)26−(x+12)24=1
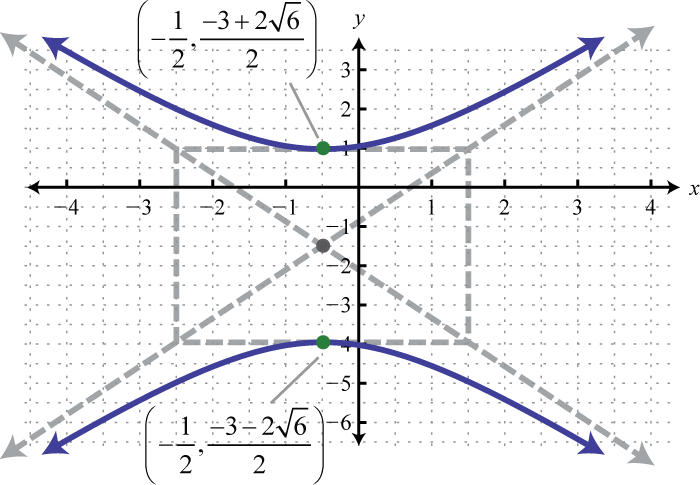
Figura 8.E.23
Ejercicio8.E.20
Identificar las secciones cónicas y reescribir en forma estándar.
- x2+y2−2x−8y+16=0
- x2+2y2+4x−24y+74=0
- x2−y2−6x−4y+3=0
- x2+y−10x+22=0
- x2+12y2−12x+24=0
- x2+y2+10y+22=0
- 4y2−20x2+16y+20x−9=0
- 16x−16y2+24y−25=0
- 9x2−9y2−6x−18y−17=0
- 4x2+4y2+4x−8y+1=0
- Contestar
-
1. Círculo;(x−1)2+(y−4)2=1
3. hipérbola;(x−3)22−(y+2)22=1
5. Elipse;(x−6)212+y2=1
7. hipérbola;(y+2)25−(x−12)2=1
9. hipérbola;(x−13)2−(y+1)2=1
Ejercicio8.E.21
Dada la gráfica, escribir la ecuación en forma general.
1.
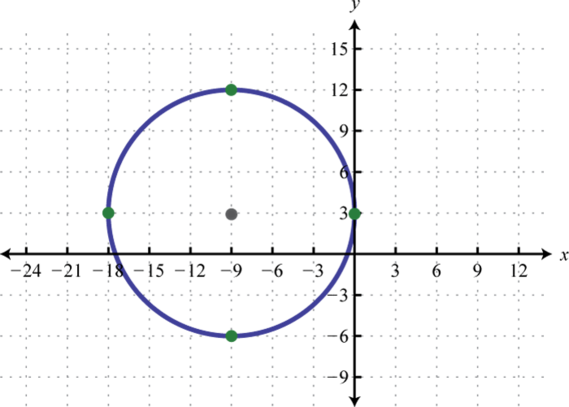
2.

3.
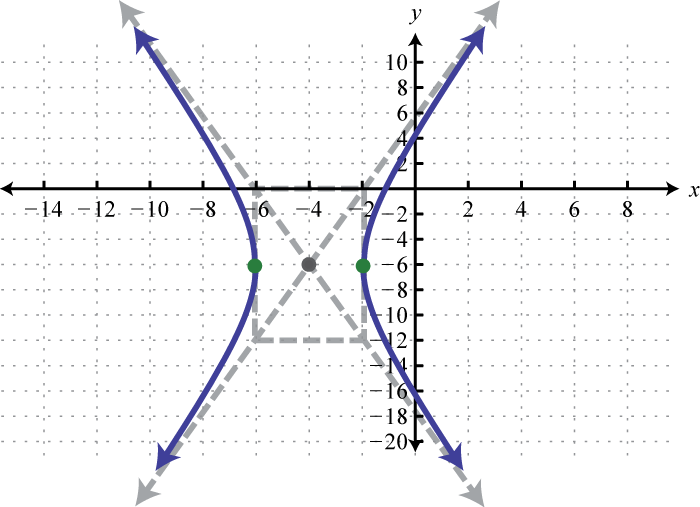
4.
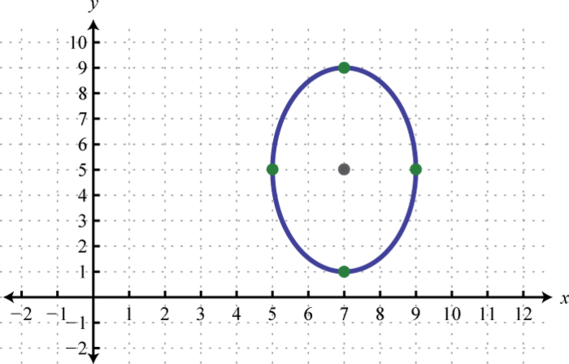
5.
6.

- Contestar
-
1. x2+y2+18x−6y+9=0
3. 9x2−y2+72x−12y+72=0
5. 9x2+64y2+54x−495=0
Ejercicio8.E.22
Resolver.
- {x2+y2=8x−y=4
- {x2+y2=1x+2y=1
- {x2+3y2=42x−y=1
- {2x2+y2=5x+y=3
- {3x2−2y2=1x−y=2
- {x2−3y2=10x−2y=1
- {2x2+y2=114x+y2=5
- {x2+4y2=12x2+4y=5
- {5x2−y2=10x2+y=2
- {2x2+y2=12x−4y2=−3
- {x2+4y2=10xy=2
- {y+x2=0xy−8=0
- {1x+1y=101x−1y=6
- {1x+1y=1y−x=2
- {x−2y2=3y=√x−4
- {(x−1)2+y2=1y−√x=0
- Contestar
-
1. (2,−2)
3. (−113,−1513),(1,1)
5. (−9,−11),(1,−1)
7. (−1,−3),(−1,3)
9. (−√2,0),(√2,0),(−√7,−5),(√7,−5)
11. (√2,√2)⋅(−√2,−√2)⋅(2√2,√22)⋅(−2√2,−√22)
13. (18,12)
15. (5,1)
Examen de muestra
Ejercicio8.E.23
- Teniendo en cuenta dos puntos(−4,−6) y(2,−8):
- Calcula la distancia entre ellos.
- Encuentra el punto medio entre ellos.
- Determinar el área de un círculo cuyo diámetro está definido por los puntos(4,−3) y(−1,2).
- Contestar
-
1. (1)2√10 unidades; (2)(−1,−7)
Ejercicio8.E.24
Reescribir en forma estándar y gráfica. Encuentra el vértice y todas las intercepciones si las hay.
- y=−x2+6x−5
- x=2y2+4y−6
- x=−3y2+3y+1
- Encuentra la ecuación de un círculo en forma estándar con2√5 unidades de centro(−6,3) y radio.
- Contestar
-
1. y=−(x−3)2+4;
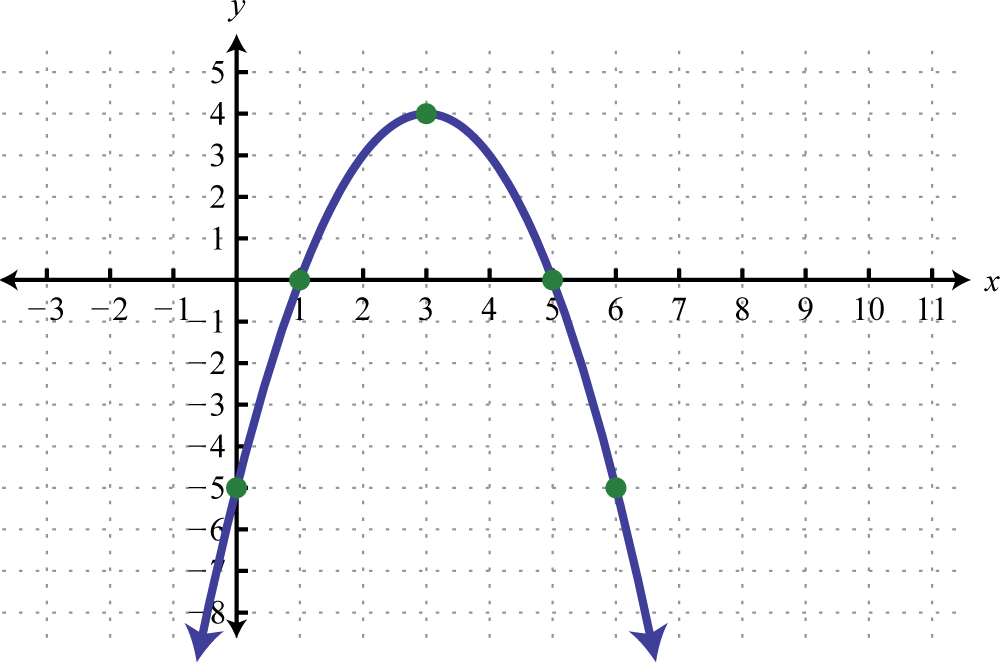
Figura 8.E.30 3. x=−3(y−12)2+74;
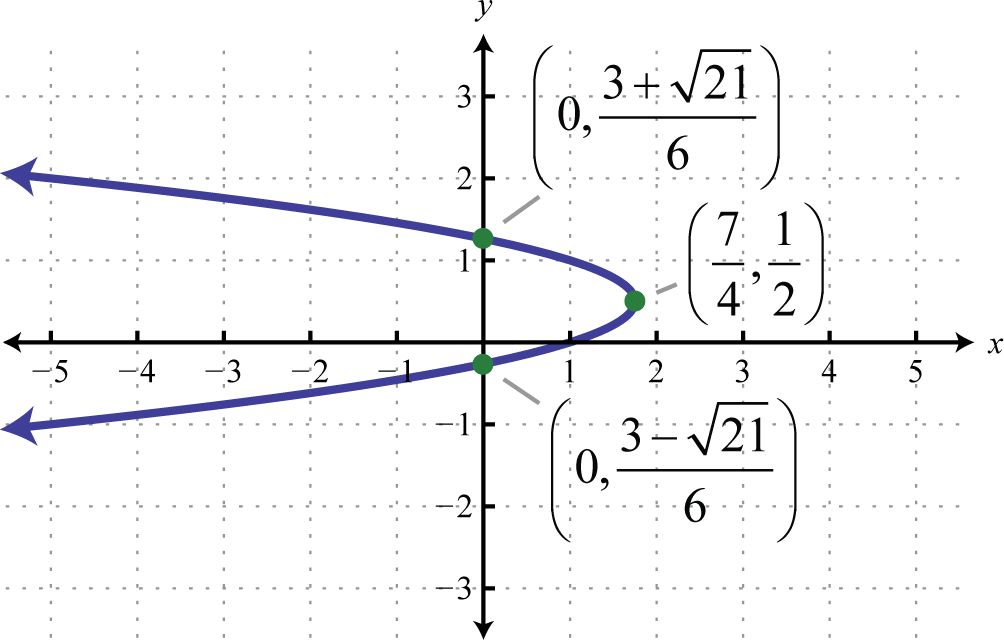
Figura 8.E.31
Ejercicio8.E.25
Esbozar la gráfica de la sección cónica dada su ecuación en forma estándar.
- (x−4)2+(y+1)2=45
- (x+3)24+y29=1
- y23−x29=1
- x216−(y−2)2=1
- Contestar
-
1.
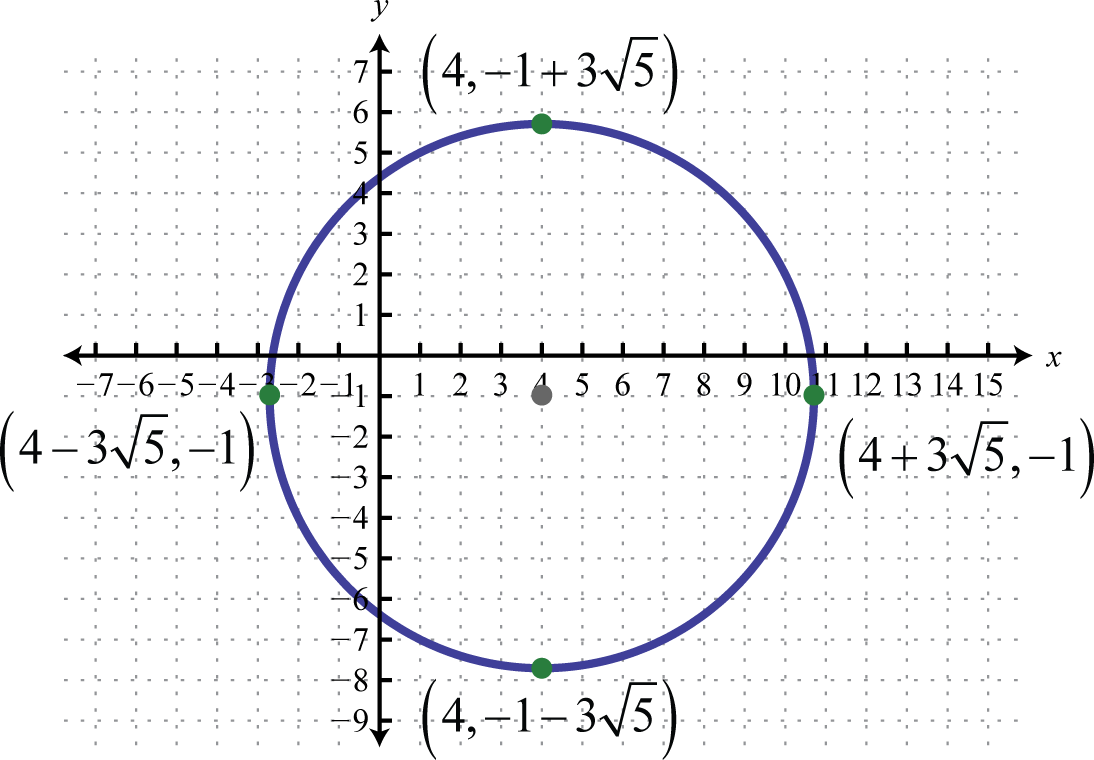
Figura 8.E.32 3.
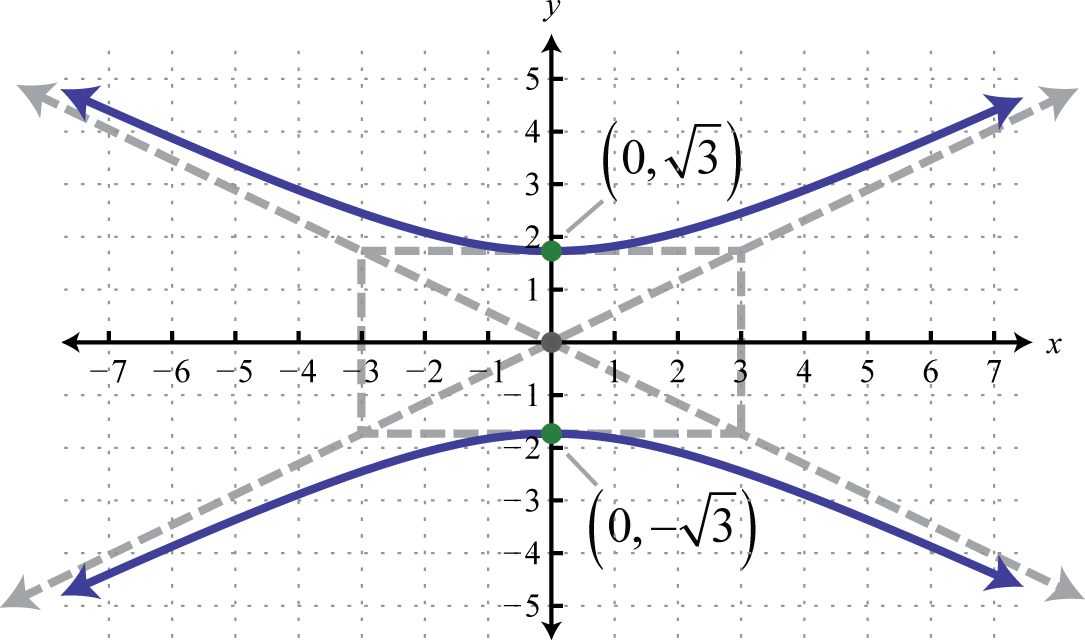
Figura 8.E.33
Ejercicio8.E.26
Reescribir en forma estándar y gráfica.
- 9x2+4y2−144x+16y+556=0
- x−y2+6y+7=0
- x2+y2+20x−20y+100=0
- 4y2−x2+40y−30x−225=0
- Contestar
-
1. (x−8)24+(y+2)29=1;
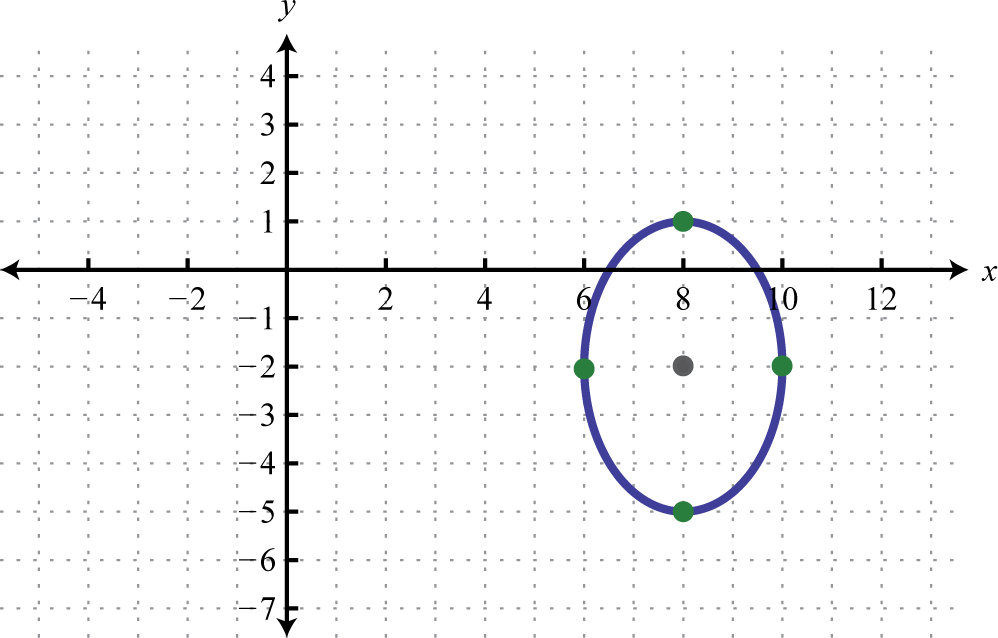
Figura 8.E.34 3. (x+10)2+(y−10)2=100;
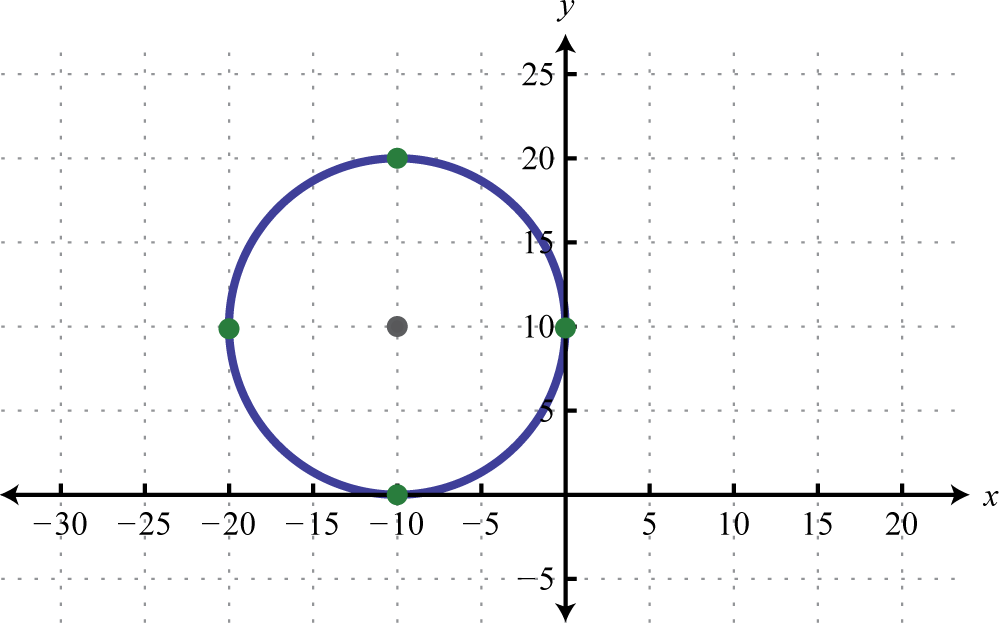
Figura 8.E.35
Ejercicio8.E.27
Encuentra losx - yy -interceptos.
- x=−2(y−4)2+9
- (y−1)212−(x+1)2=1
- Contestar
-
1. x-interceptar:(−23,0);y -intercepta:(0,8±3√22)
Ejercicio8.E.28
Resolver.
- {x+y=2y=−x2+4
- {y−x2=−3x2+y2=9
- {2x−y=1(x+1)2+2y2=1
- {x2+y2=6xy=3
- Contestar
-
1. (−1,3),(2,0)
3. ∅
Ejercicio8.E.29
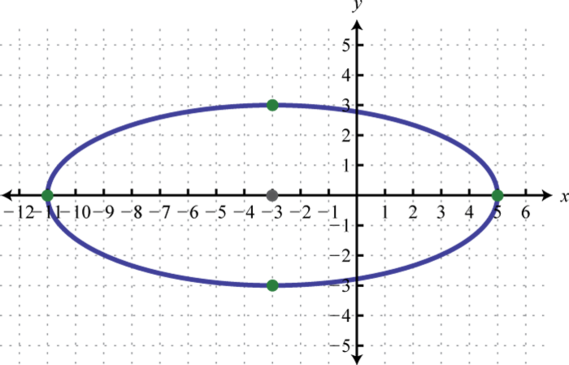
- Encuentra la ecuación de una elipse en forma estándar con vértices(−3,−5)(5,−5) y un radio menor2 unidades de longitud.
- Encuentra la ecuación de una hipérbola en forma estándar abriendo izquierda y derecha con vértices(±√5,0) y un eje conjugado que mide10 unidades.
- Dada la gráfica de la elipse, determinar su ecuación en forma general.
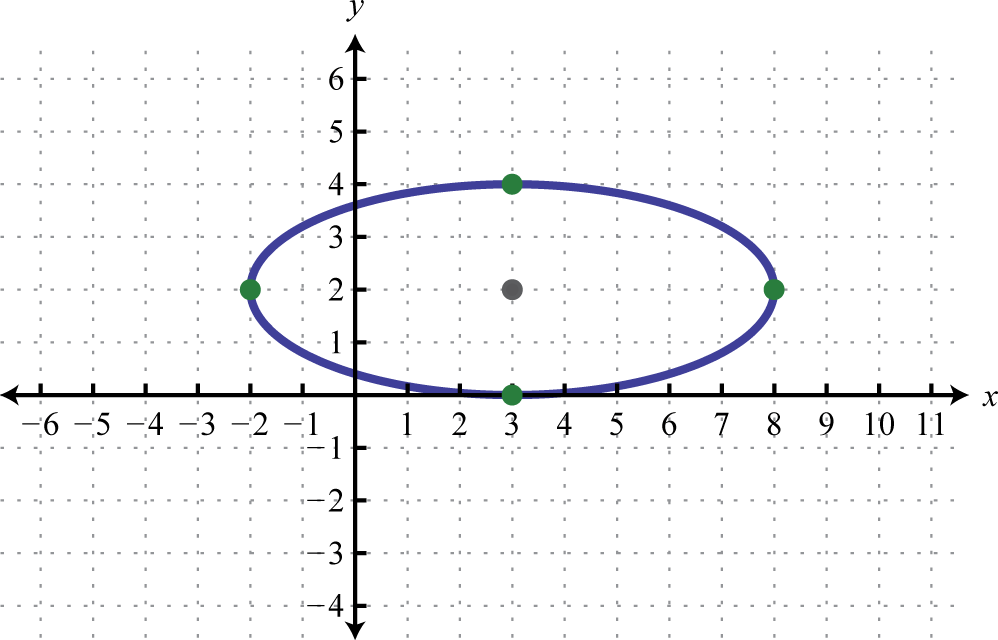
4. Una cubierta rectangular tiene un área de pies80 cuadrados y un perímetro que mide36 pies. Encuentra las dimensiones de la cubierta.
5. La diagonal de un rectángulo mide2√13 centímetros y el perímetro mide20 centímetros. Encuentra las dimensiones del rectángulo.
- Contestar
-
1. (x−1)216+(y+5)24=1
3. 4x2+25y2−24x−100y+36=0
5. 6centímetros por4 centímetros


