7.5: Estrategia General para Factorizar Polinomios

Objetivos de aprendizaje

Al final de esta sección, podrás:

• Reconocer y utilizar el método apropiado para factorizar un polinomio completamente

Nota

Antes de comenzar, toma este cuestionario de preparación.

1. Factor\(y^{2}-2 y-24\).
   Si te perdiste este problema, revisa el Ejercicio 7.2.19.
2. Factor\(3 t^{2}+17 t+10\).
   Si te perdiste este problema, revisa el Ejercicio 7.3.28.
3. Factor\(36 p^{2}-60 p+25\).
   Si te perdiste este problema, revisa el Ejercicio 7.4.1.
4. Factor\(5 x^{2}-80\).
   Si te perdiste este problema, revisa el Ejercicio 7.4.31.

Reconocer y Utilizar el Método Apropiado para Factorizar un Polinomio Completamente

Ahora te has familiarizado con todos los métodos de factorización que necesitarás en este curso. (En tu próximo curso de álgebra, se agregarán más métodos a tu repertorio). La siguiente figura resume todos los métodos de factorización que hemos cubierto. La figura\(\PageIndex{1}\) describe una estrategia que debes usar al factorizar polinomios.

Figura\(\PageIndex{1}\)

POLINOMIALES FACTORALES.

1. ¿Hay un factor común más grande?
   • Factorial hacia fuera.
2. ¿Es el polinomio un binomio, trinomio, o hay más de tres términos?
   • Si es un binomio:
     ¿Es una suma?
     • ¿De plazas? Las sumas de cuadrados no factorializan.
     • ¿De cubos? Usa el patrón de suma de cubos.
     ¿Es una diferencia?
     • ¿De plazas? Factor como producto de conjugados.
     • ¿De cubos? Usa la diferencia de patrón de cubos.
   • Si es trinomio:
     ¿Es de la forma\(x^{2}+b x+c ?\)? Deshacer FOIL.
     ¿Es de la forma\(a x^{2}+b x+c\)?
     • Si aa y cc son cuadrados, comprueba si se ajusta al patrón cuadrado trinomial.
     • Utilice el método de prueba y error o “ac”.
   • Si tiene más de tres términos:
     Usa el método de agrupación.
3. Cheque.
   • ¿Se factoriza completamente?
   • ¿Los factores se multiplican de nuevo al polinomio original?

Recuerda, un polinomio está completamente factorizado si, aparte de los monomios, ¡sus factores son primos!

Ejercicio\(\PageIndex{1}\)

Factor completamente:\(4 x^{5}+12 x^{4}\)

Responder

\ (\ begin {array} {lll}\ text {¿Hay un GCF? } &\ text {Sí,} 4 x^ {4} & 4 x^ {5} +12 x^ {4}\\\ text {Factorizar el GCF.} & &4 x^ {4} (x+3)\\\ text {Entre paréntesis, ¿es un binomio, un} & &\\\ text {trinomio, o hay más de tres términos? } &\ text {Binomial.} &\\\ quad\ text {¿Es una suma? } & &\ text {Sí.}\\\ quad\ text {¿De cuadrados? ¿De cubos? } & &\ text {No.}\\\ text {Check.}
\\\\\ quad\ text {¿La expresión está factorizada completamente? } & &\ text {Sí.}\\\ quad\ texto {Multiplicar.}\\\ begin {array} {l} {4 x^ {4} (x+3)}\\ {4 x^ {4}\ cdot x+4 x^ {4}\ cdot 3}\\ {4 x^ {5} +12 x^ {4}}\ marca de verificación\ end {array}\ end array {}\)

Ejercicio\(\PageIndex{2}\)

Factor completamente:\(3 a^{4}+18 a^{3}\)

Responder

3\(a^{3}(a+6)\)

Ejercicio\(\PageIndex{3}\)

Factor completamente:\(45 b^{6}+27 b^{5}\)

Responder

9\(b^{5}(5 b+3)\)

Ejercicio\(\PageIndex{4}\)

Factor completamente:\(12 x^{2}-11 x+2\)

Responder

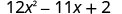
¿Existe un GCF?	No.
¿Es un binomio, trinomio, o hay más de tres términos?	Trinomio.
¿Son a y c cuadrados perfectos?	No, a = 12, no un cuadrado perfecto.
Utilice el método de prueba y error o el método “ac”. Vamos a utilizar prueba y error aquí.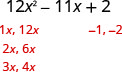

Cheque. \(\begin{array}{l}{(3 x-2)(4 x-1)} \\ {12 x^{2}-3 x-8 x+2} \\ {12 x^{2}-11 x+2 }\checkmark \end{array}\)

Ejercicio\(\PageIndex{5}\)

Factor completamente:\(10 a^{2}-17 a+6\)

Responder

\((5 a-6)(2 a-1)\)

Ejercicio\(\PageIndex{6}\)

Factor completamente:\(8 x^{2}-18 x+9\)

Responder

\((2 x-3)(4 x-3)\)

Ejercicio\(\PageIndex{7}\)

Factor completamente:\(g^{3}+25 g\)

Responder

\(\begin{array}{lll} \text { Is there a GCF? } & \text{Yes, g.} &g^{3}+25 g \\\text { Factor out the GCF. } & &g\left(g^{2}+25\right) \\ \text { In the parentheses, is it a binomial, trinomial, } & & \\ \text { or are there more than three terms? } &\text { Binomial. } & \\ \quad \text { Is it a sum? Of squares? } & \text { Yes. } & \text { Sums of squares are prime. } \\\text { Check. } \\ \\ \quad \text { Is the expression factored completely? } &\text { Yes. } \\ \quad \text { Multiply. } \\ \qquad \begin{array}{l}{g\left(g^{2}+25\right)} \\ {g^{3}+25 g }\checkmark \end{array} \end{array}\)

Ejercicio\(\PageIndex{8}\)

Factor completamente:\(x^{3}+36 x\)

Responder

\(x\left(x^{2}+36\right)\)

Ejercicio\(\PageIndex{9}\)

Factor completamente:\(27 y^{2}+48\)

Responder

3\(\left(9 y^{2}+16\right)\)

Ejercicio\(\PageIndex{10}\)

Factor completamente:\(12 y^{2}-75\)

Responder

\(\begin{array}{lll} \text { Is there a GCF? } & \text{Yes, 3.} &12 y^{2}-75 \\\text { Factor out the GCF. } & &3\left(4 y^{2}-25\right) \\ \text { In the parentheses, is it a binomial, trinomial, } & & \\ \text { or are there more than three terms? } &\text { Binomial. } & \\ \text { Is it a sum?} & \text { No. } & \\ \text { Is it a difference? Of squares or cubes? } &\text { Yes, squares. } & 3\left((2 y)^{2}-(5)^{2}\right) \\ \text { Write as a product of conjugates. } & &3(2 y-5)(2 y+5)\\\text { Check. } \\ \\ \text { Is the expression factored completely? } & \text{ Yes.}& \\ \text { Neither binomial is a difference of } \\ \text { squares. } \\ \text{ Multiply.} \\ \quad \begin{array}{l}{3(2 y-5)(2 y+5)} \\ {3\left(4 y^{2}-25\right)} \\ {12 y^{2}-75}\checkmark \end{array} \end{array}\)

Ejercicio\(\PageIndex{11}\)

Factor completamente:\(16 x^{3}-36 x\)

Responder

4\(x(2 x-3)(2 x+3)\)

Ejercicio\(\PageIndex{12}\)

Factor completamente:\(27 y^{2}-48\)

Responder

3\((3 y-4)(3 y+4)\)

Ejercicio\(\PageIndex{13}\)

Factor completamente:\(4 a^{2}-12 a b+9 b^{2}\)

Responder

¿Existe un GCF?	No.
¿Es un binomio, trinomio, o hay más términos?
Trinomio con\(a\neq 1\). Pero el primer término es un cuadrado perfecto.
¿El último término es una plaza perfecta?	Sí.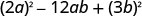
¿Se ajusta al patrón,\(a^{2}-2 a b+b^{2}\)?	Sí.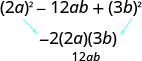
Escríbelo como un cuadrado.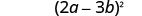
Comprueba tu respuesta.
¿Se factoriza completamente la expresión?
Sí.
El binomio no es una diferencia de cuadrados.
Multiplicar.
\((2 a-3 b)^{2}\)
\((2 a)^{2}-2 \cdot 2 a \cdot 3 b+(3 b)^{2}\)
\(4 a^{2}-12 a b+9 b^{2} \checkmark\)

Ejercicio\(\PageIndex{14}\)

Factor completamente:\(4 x^{2}+20 x y+25 y^{2}\)

Responder

\((2 x+5 y)^{2}\)

Ejercicio\(\PageIndex{15}\)

Factor completamente:\(9 m^{2}+42 m n+49 n^{2}\)

Responder

\((3 m+7 n)^{2}\)

Ejercicio\(\PageIndex{16}\)

Factor completamente:\(6 y^{2}-18 y-60\)

Responder

\(\begin{array}{lll} \text { Is there a GCF? } & \text{Yes, 6.} &6 y^{2}-18 y-60 \\\text { Factor out the GCF. } & \text { Trinomial with leading coefficient } 1&6\left(y^{2}-3 y-10\right) \\ \text { In the parentheses, is it a binomial, trinomial, } & & \\ \text { or are there more terms? } & & \\ \text { "Undo' FOIL. } & 6(y\qquad )(y\qquad ) &6(y+2)(y-5) \\ \text { Check your answer. } \\ \text { Is the expression factored completely? } & & \text{ Yes.} \\ \text { Neither binomial is a difference of squares. } \\ \text { Multiply. } \\ \\\qquad \begin{array}{l}{6(y+2)(y-5)} \\ {6\left(y^{2}-5 y+2 y-10\right)} \\ {6\left(y^{2}-3 y-10\right)} \\ {6 y^{2}-18 y-60} \checkmark \end{array} \end{array}\)

Ejercicio\(\PageIndex{17}\)

Factor completamente:\(8 y^{2}+16 y-24\)

Responder

8\((y-1)(y+3)\)

Ejercicio\(\PageIndex{18}\)

Factor completamente:\(5 u^{2}-15 u-270\)

Responder

5\((u-9)(u+6)\)

Ejercicio\(\PageIndex{19}\)

Factor completamente:\(24 x^{3}+81\)

Responder

¿Existe un GCF?	Sí, 3.	\(24 x^{3}+81\)
Factorial hacia fuera.		3\(\left(8 x^{3}+27\right)\)
Entre paréntesis, ¿es un binomio, trinomio, o hay más de tres términos?	Binomial.
¿Es una suma o diferencia?	Suma.
¿De cuadrados o cubos?	Suma de cubos.
Escríbelo usando el patrón de suma de cubos.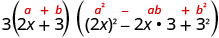
¿Se factoriza completamente la expresión?	Sí.	3\((2 x+3)\left(4 x^{2}-6 x+9\right)\)
Verificar multiplicando.		Te dejamos el cheque a ti.

Ejercicio\(\PageIndex{20}\)

Factor completamente:\(250 m^{3}+432\)

Responder

2\((5 m+6)\left(25 m^{2}-30 m+36\right)\)

Ejercicio\(\PageIndex{21}\)

Factor completamente:\(81 q^{3}+192\)

Responder

\(3(3q+4)\left(9q^{2}-12 q+16\right)\)

Ejercicio\(\PageIndex{22}\)

Factor completamente:\(2 x^{4}-32\)

Responder

\(\begin{array}{llc} \text { Is there a GCF? } & \text{Yes, 2.} &2 x^{4}-32 \\\text { Factor out the GCF. } & &2\left(x^{4}-16\right) \\ \text { In the parentheses, is it a binomial, trinomial, } & & \\ \text { or are there more than three terms? } & \text { Binomial. }& \\ \text { Is it a sum or difference? } &\text { Yes. }& \\\text { Of squares or cubes? } & \text { Difference of squares. } & 2\left(\left(x^{2}\right)^{2}-(4)^{2}\right) \\ \text { Write it as a product of conjugates. } & & 2\left(x^{2}-4\right)\left(x^{2}+4\right) \\ \text { The first binomial is again a difference of squares. } & & 2\left((x)^{2}-(2)^{2}\right)\left(x^{2}+4\right) \\ \text { Write it as a product of conjugates. } & & 2(x-2)(x+2)\left(x^{2}+4\right) \\ \text { Is the expression factored completely? } &\text { Yes. } & \\ \\ \text { None of these binomials is a difference of squares. } \\ \text { Check your answer. } \\ \text{ Multiply. }\\ \\ \qquad \qquad \begin{array}{l}{2(x-2)(x+2)\left(x^{2}+4\right)} \\ {2(x-2)(x+2)\left(x^{2}+4\right)} \\ {2(x-10)} \\ {2 x^{4}-32} \checkmark \end{array} \end{array}\)

Ejercicio\(\PageIndex{23}\)

Factor completamente:\(4 a^{4}-64\)

Responder

4\(\left(a^{2}+4\right)(a-2)(a+2)\)

Ejercicio\(\PageIndex{24}\)

Factor completamente:\(7 y^{4}-7\)

Responder

7\(\left(y^{2}+1\right)(y-1)(y+1)\)

Ejercicio\(\PageIndex{25}\)

Factor completamente:\(3 x^{2}+6 b x-3 a x-6 a b\)

Responder

\(\begin{array}{llc} \text { Is there a GCF? } & \text{Yes, 3.} &3 x^{2}+6 b x-3 a x-6 a b\\\text { Factor out the GCF. } & &3\left(x^{2}+2 b x-a x-2 a b\right)\\ \text { In the parentheses, is it a binomial, trinomial, } &\text { More than } 3 & \\ \text { or are there more terms? } &\text { terms. } & \\ \text { Use grouping. } & & \begin{array}{c}{3[x(x+2 b)-a(x+2 b)]} \\ {3(x+2 b)(x-a)}\end{array} \\ \text { Check your answer. } \\ \\ \text { Is the expression factored completely? Yes. } \\ \text { Multiply. } \\\qquad \qquad \begin{array}{l}{3(x+2 b)(x-a)} \\ {3\left(x^{2}-a x+2 b x-2 a b\right)} \\ {3 x^{2}-3 a x+6 b x-6 a b} \checkmark \end{array}\end{array}\)

Ejercicio\(\PageIndex{26}\)

Factor completamente:\(6 x^{2}-12 x c+6 b x-12 b c\)

Responder

6\((x+b)(x-2 c)\)

Ejercicio\(\PageIndex{27}\)

Factor completamente:\(16 x^{2}+24 x y-4 x-6 y\)

Responder

2\((4 x-1)(x+3 y)\)

Ejercicio\(\PageIndex{28}\)

Factor completamente:\(10 x^{2}-34 x-24\)

Responder

\(\begin{array}{llc} \text { Is there a GCF? } & \text{Yes, 2.} &10 x^{2}-34 x-24\\\text { Factor out the GCF. } & &2\left(5 x^{2}-17 x-12\right)\\ \text { In the parentheses, is it a binomial, trinomial, } &\text { Trinomial with } & \\ \text { or are there more than three terms? } &\space a \neq 1 & \\ \text { Use trial and error or the "ac" method. } & & 2\left(5 x^{2}-17 x-12\right) \\ & & 2(5 x+3)(x-4) \\ \text { Check your answer. Is the expression factored } \\\text { completely? Yes. }\\ \\ \text { Multiply. } \\ \qquad \begin{array}{l}{2(5 x+3)(x-4)} \\ {2\left(5 x^{2}-20 x+3 x-12\right)} \\ {2\left(5 x^{2}-17 x-12\right)} \\ {10 x^{2}-34 x-24}\checkmark \end{array}\end{array}\)

Ejercicio\(\PageIndex{29}\)

Factor completamente:\(4 p^{2}-16 p+12\)

Responder

4\((p-1)(p-3)\)

Ejercicio\(\PageIndex{30}\)

Factor completamente:\(6 q^{2}-9 q-6\)

Responder

3\((q-2)(2 q+1)\)

Conceptos clave

• Estrategia General para Factorizar Polinomios Ver Figura\(\PageIndex{1}\).
• Cómo factorizar polinomios
  1. ¿Hay un factor común más grande? Factorial hacia fuera.
  2. ¿Es el polinomio un binomio, trinomio, o hay más de tres términos?
     • Si es un binomio:
       ¿Es una suma?
       • ¿De plazas? Las sumas de cuadrados no factorizan.
       • ¿De cubos? Usa el patrón de suma de cubos.
       ¿Es una diferencia?
       • ¿De plazas? Factor como producto de conjugados.
       • ¿De cubos? Usa la diferencia de patrón de cubos.
     • Si es trinomio:
       ¿Es de la forma\(x^{2}+b x+c\)? Deshacer FOIL.
       ¿Es de la forma\(a x^{2}+b x+c\)?
       • Si 'a' y 'c' son cuadrados, verifique si se ajusta al patrón cuadrado trinomial.
       • Utilice el método de prueba y error o 'ac'.
     • Si tiene más de tres términos:
       Usa el método de agrupación.
  3. Cheque. ¿Se factoriza completamente? ¿Los factores se multiplican de nuevo al polinomio original?