Inicio
Educación Básica
Geometría
3: Líneas
3.4: Ángulos correspondientes

3.4: Ángulos correspondientes

Última actualización
Guardar como PDF

Page ID: 107386

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \) \( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)\(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\) \(\newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\) \( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\) \( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\) \( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\) \( \newcommand{\Span}{\mathrm{span}}\)\(\newcommand{\AA}{\unicode[.8,0]{x212B}}\)

Identificar los ángulos correspondientes como ángulos coincidentes.

Los ángulos correspondientes son dos ángulos que están en el “mismo lugar” con respecto a la transversal pero en líneas diferentes. Imagínese deslizar los cuatro ángulos formados con línea de\(l\) abajo a línea\(m\). Los ángulos que coinciden son los correspondientes.

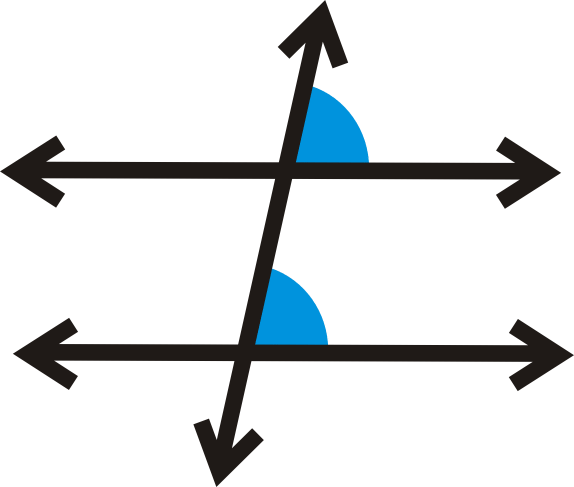
f-d_e4af34d1b0947ec9e0c6e5d881d69d25e8e33ffddc2b11087f8241d5+image_tiny+image_tiny.png — Figura\(\PageIndex{1}\)

Postulado de ángulos correspondientes: Si dos líneas paralelas son cortadas por una transversal, entonces los ángulos correspondientes son congruentes.

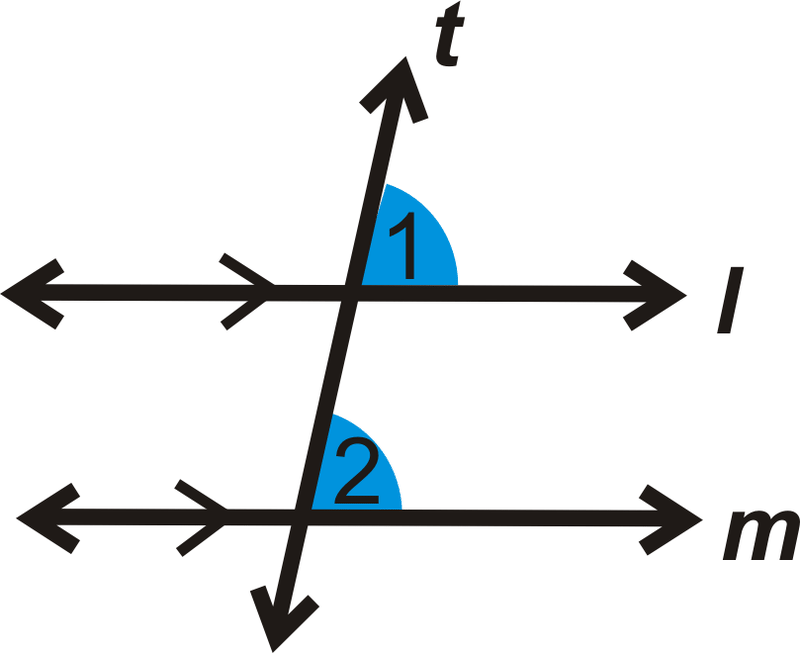
F-D_5cb183f69057304b506c6f83e8ea129defa8f490d1406072df9504df+image_tiny+image_tiny.png — Figura\(\PageIndex{2}\)

Si\(l \parallel m\), entonces\(\angle 1\cong \angle 2\).

Converse de Ángulos Correspondientes Postulado: Si los ángulos correspondientes son congruentes cuando dos líneas son cortadas por una transversal, entonces las líneas son paralelas.

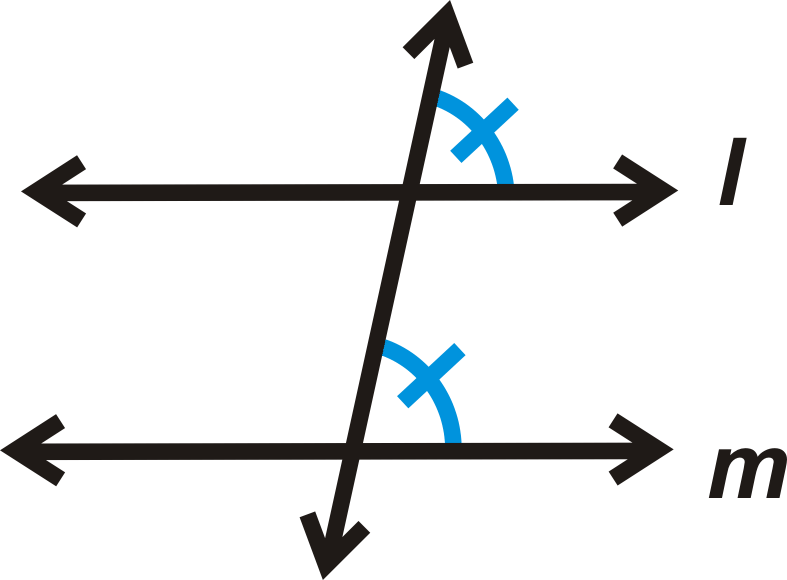
f-d_e07b6e10cf346793a9a35ddbbae1c31761ccab2ba7990215a74cb801+image_tiny+image_tiny.png — Figura\(\PageIndex{3}\)

entonces\(l \parallel m\).

¿Y si te presentaran dos ángulos que están en el mismo lugar con respecto a lo transversal pero en líneas diferentes? ¿Cómo describirías estos ángulos y qué podrías concluir sobre sus medidas?

Ejemplo\(\PageIndex{1}\)

Si\(m\angle 2=76^{\circ}\), ¿qué es\(m\angle 6\)?

f-d_7d690a17ce6b2e3bc2ec2070038ceed705eb9a6272064c8c84195102+image_tiny+image_tiny.png — Figura\(\PageIndex{4}\)

Solución

\(\angle 2\)y\(\angle 6\) son ángulos correspondientes y l||m de las flechas en la figura. \(\angle 2\cong \angle 6\)por el Postulado de Ángulos Correspondientes, lo que significa que\(m\angle 6=76^{\circ}\).

Ejemplo\(\PageIndex{2}\)

Usando las medidas de\ ángulo 2 y\ ángulo 6 del Ejemplo 1, encuentra todas las demás medidas de ángulo.

Solución

Si\(m\angle 2=76^{\circ}\), entonces\(m\angle 1=180^{\circ}−76^{\circ}=104^{\circ}\) (par lineal). \ ángulo 3\ cong\ ángulo 2\) (ángulos verticales), así\(m\angle 3=76^{\circ}\). \(m\angle 4=104^{\circ}\)(ángulo vertical con\ ángulo 1\)).

Por el Postulado de Ángulos Correspondientes\(\angle 1\cong \angle 5\), sabemos\(\angle 2\cong \angle 6\),\(\angle 3\cong \angle 7\),\(\angle 4\cong \angle 8\), y, así\(m\angle 5=104^{\circ}\),\(m\angle 6=76^{\circ}\),\(m\angle 7=76^{\circ}\), y\(m\angle 8=104^{\circ}\).

Ejemplo\(\PageIndex{3}\)

Encuentra el valor de y:

f-d_764c6382932c5ce076e8e9d394401386d1b5546376807aa3ce8ff988+image_tiny+image_tiny.png — Figura\(\PageIndex{5}\)

Solución

Las líneas horizontales se marcan paralelas y el ángulo marcado\(2y\) corresponde al ángulo marcado 80 por lo que estos dos ángulos son congruentes. Esto significa eso\(2y=80\) y por lo tanto\(y=40\).

Ejemplo\(\PageIndex{4}\)

Si a||b, ¿qué pares de ángulos son congruentes por el Postulado de Ángulos Correspondientes?

f-d_db29b4839d72e881911e626f7e7accc836a573e5d9a558caa2885019+image_tiny+image_tiny.png — Figura\(\PageIndex{6}\)

Solución

Hay 4 pares de ángulos correspondientes congruentes:

\(\angle 1\cong \angle 5\),\(\angle 2\cong \angle 6\),\(\angle 3\cong \angle 7\), y\(\angle 4\cong \angle 8\).

Ejemplo\(\PageIndex{5}\)

Si\(m\angle 8=110^{\circ}\) y\(m\angle 4=110^{\circ}\), entonces ¿qué sabemos de líneas\(l\) y\(m\)?

Solución

\(\angle 8\)y\(\angle 4\) son ángulos correspondientes. Ya que\(m\angle 8=m\angle 4\), podemos concluir que l||m.

Revisar

Determinar si el par angular\(\angle 4\) y\(\angle 2\) es congruente, suplementario o ninguno:

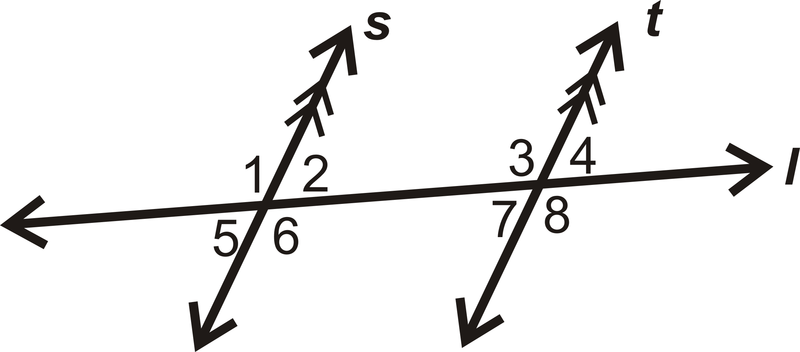
Figura\(\PageIndex{8}\)
Dé dos ejemplos de ángulos correspondientes en el diagrama:

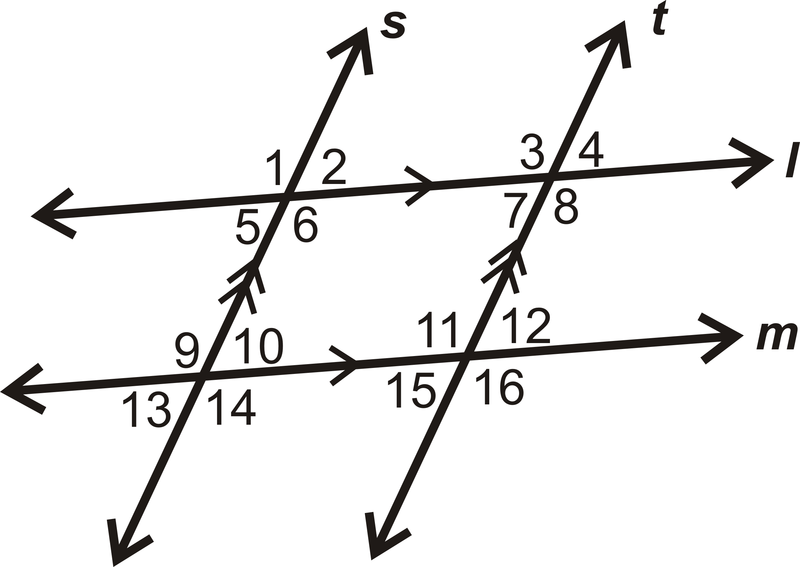
Figura\(\PageIndex{9}\)
Encuentra el valor de\(x\):

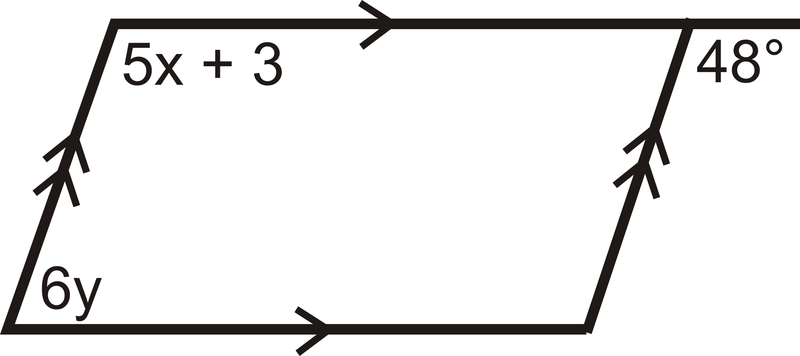
Figura\(\PageIndex{10}\)
¿Las líneas son paralelas? ¿Por qué o por qué no?

Figura\(\PageIndex{11}\)
¿Las líneas son paralelas? Justifica tu respuesta.

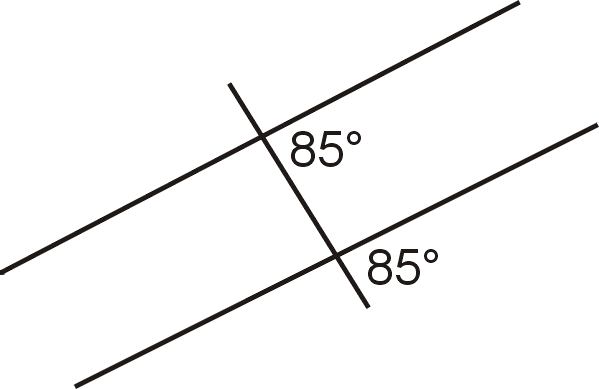
Figura\(\PageIndex{12}\)

Para 6-10, ¿cuál tiene\(x\) que ser el valor de para hacer paralelas las líneas?

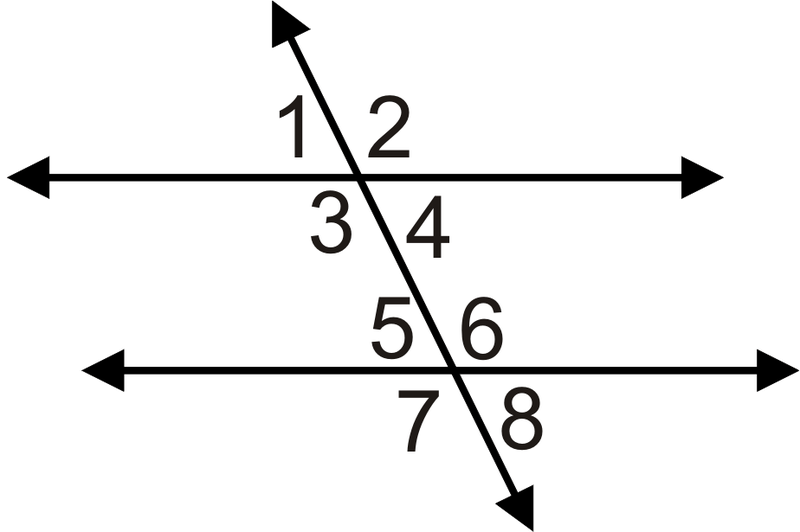
f-d_963dbdfc7b826d8117ff8f1171f6c6bf93e5c8d514fd8352bb797b67+imagen_tiny+imagen_tiny.png — Figura\(\PageIndex{13}\)

Si\(m\angle 1=(6x−5)^{\circ}\) y\(m\angle 5=(5x+7)^{\circ}\).
Si\(m\angle 2=(3x−4)^{\circ}\) y\(m\angle 6=(4x−10)^{\circ}\).
Si\(m\angle 3=(7x−5)^{\circ}\) y\(m\angle 7=(5x+11)^{\circ}\).
Si\(m\angle 4=(5x−5)^{\circ}\) y\(m\angle 8=(3x+15)^{\circ}\).
Si\(m\angle 2=(2x+4)^{\circ}\) y\(m\angle 6=(5x−2)^{\circ}\).

Reseña (Respuestas)

Para ver las respuestas de Revisar, abra este archivo PDF y busque la sección 3.3.

Recursos

vocabulario

Término	Definición
Ángulos correspondientes	Los ángulos correspondientes son dos ángulos que están en la misma posición con respecto a la transversal, pero en líneas diferentes.

Recurso Adicional

Elemento Interactivo

Video: Principios de Ángulos Correspondientes - Básico

Actividades: Preguntas de discusión de ángulos correspondientes

Ayudas de estudio: Guía de estudio de ángulos y transversales

Práctica: Ángulos correspondientes

Mundo Real: Ángulos Correspondientes