4.40: Aplicaciones de la Fórmula de Distancia
- Page ID
- 107549
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)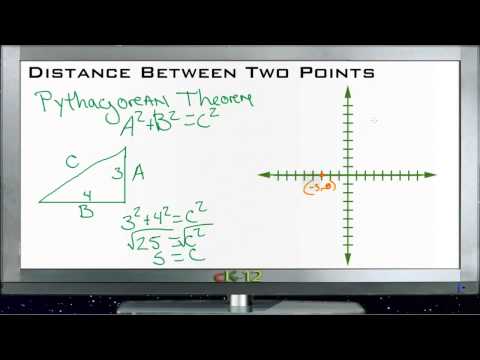Largo entre dos puntos basado en un triángulo rectángulo.
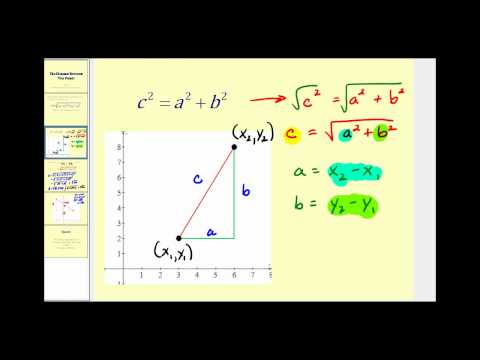
Fórmula de distancia en el plano de coordenadas
La distancia entre dos puntos\((x_1, y_1)\) y se\((x_2,y_2)\) puede definir como\(d=\sqrt{(x_2−x_1)^2+(y_2−y_1)^2}\). Esto se llama fórmula de distancia. ¡Recuerda que las distancias son siempre positivas!
¿Y si te dieran las coordenadas de dos puntos? ¿Cómo podrías encontrar lo lejos que están estos dos puntos?
Ejemplo\(\PageIndex{1}\)
Encuentra la distancia entre\((-2, -3)\) y\((3, 9)\).
Solución
Utilice la fórmula de distancia, conecte los puntos y simplifique.
\(\begin{align*}d&=\sqrt{(3−(−2))^2+(9−(−3))^2} \\ &=\sqrt{(5)^2+(12)^2} \\ &= \sqrt{25+144} \\ &= \sqrt{169}=13\text{ units }\end{align*}\)
Ejemplo\(\PageIndex{2}\)
Encuentra la distancia entre\((12, 26)\) y\((8, 7)\).
Solución
Utilice la fórmula de distancia, conecte los puntos y simplifique.
\(\begin{align*}d&=\sqrt{(8−12)^2+(7−26)^2} \\ &= \sqrt{(−4)^2+(−19)^2} \\ &= \sqrt{16+361} \\ &= \sqrt{377}\approx 19.42\text{ units }\end{align*}\)
Ejemplo\(\PageIndex{3}\)
Encuentra la distancia entre\((4, -2)\) y\((-10, 3)\).
Solución
Enchufe\((4, -2)\) para\((x_1, y_1)\) y\((-10, 3)\) para\((x_2,y_2)\) y simplificar.
\(\begin{align*}d&=\sqrt{(−10−4)^2+(3+2)^2} \\ &= \sqrt{(−14)^2+(5)^2} \\ &= \sqrt{196+25} \\ &= \sqrt{221}\approx 14.87\text{ units }\end{align*}\)
Ejemplo\(\PageIndex{4}\)
Encuentra la distancia entre\((3, 4)\) y\((-1, 3)\).
Solución
Enchufe (3, 4)\) para\((x_1, y_1)\) y\((-1, 3)\) para\((x_2,y_2)\) y simplificar.
\(\begin{align*}d &=\sqrt{(−1−3)^2+(3−4)^2} \\ &= \sqrt{(−4)^2+(−1)^2} \\ &= \sqrt{16+1} \\ &= \sqrt{17} \approx 4.12\text{ units }\end{align*}\)
Ejemplo\(\PageIndex{5}\)
Encuentra la distancia entre\((4, 23)\) y\((8, 14)\).
Solución
Enchufe\((4, 23)\) para\((x_1, y_1)\) y\((8, 14)\) para\((x_2,y_2)\) y simplificar.
\(\begin{align*} d&=\sqrt{(8−4)^2+(14−23)^2} \\ &=\sqrt{(4)^2+(−9)^2} \\ &=\sqrt{16+81} \\ & =\sqrt{97} \approx 9.85\text{ units }\end{align*} \)
Revisar
Encuentra la distancia entre cada par de puntos. Redondee su respuesta a la centésima más cercana.
- \((4, 15)\)y\((-2, -1)\)
- \((-6, 1)\)y\((9, -11)\)
- \((0, 12)\)y\((-3, 8)\)
- \((-8, 19)\)y\((3, 5)\)
- \((3, -25)\)y\((-10, -7)\)
- \((-1, 2)\)y\((8, -9)\)
- \((5, -2)\)y\((1, 3)\)
- \((-30, 6)\)y\((-23, 0)\)
- \((2, -2)\)y\((2, 5)\)
- \((-9, -4)\)y\((1, -1) \)
Reseña (Respuestas)
Para ver las respuestas de Revisar, abra este archivo PDF y busque la sección 3.10.
Recurso
El vocabulario
| Término | Definición |
|---|---|
| Fórmula de distancia | La distancia entre dos puntos\((x_1, y_1)\) y se\((x_2,y_2)\) puede definir como\(d=\sqrt{(x_2−x_1)^2+(y_2−y_1)^2}\). |
| Teorema de Pitágoras | El Teorema de Pitágoras es una relación matemática entre los lados de un triángulo rectángulo\(a^2+b^2=c^2\), dada por, donde\(a\) y\(b\) son patas del triángulo y c es la hipotenusa del triángulo. |
Recursos adicionales
Elemento interactivo
Video: La fórmula de la distancia
Actividades: Fórmula de distancia en el plano de coordenadas Preguntas de discusión
Ayudas de estudio: Guía de estudio de segmentos
Práctica: Aplicaciones de la Fórmula de Distancia
Mundo real: Fórmula de distancia en el plano de coordenadas