6.15: Cuadriláteros Inscritos en Círculos
( \newcommand{\kernel}{\mathrm{null}\,}\)
Cuadriláteros con cada vértice en círculo y ángulos opuestos que son complementarios.
Un polígono inscrito es un polígono donde cada vértice está en el círculo, como se muestra a continuación.

Para cuadriláteros inscritos en particular, los ángulos opuestos siempre serán complementarios.
Teorema Cuadrilátero Inscrito: Un cuadrilátero se puede inscribir en círculo si y sólo si los ángulos opuestos son suplementarios.
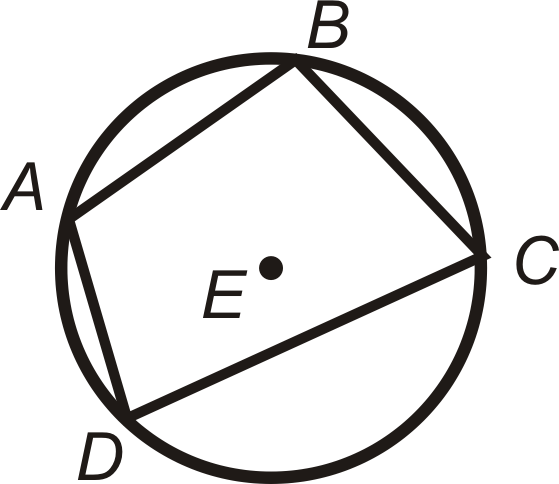
SiABCD está inscrito en⨀E, entoncesm∠A+m∠C=180∘ ym∠B+m∠D=180∘. Por el contrario, Sim∠A+m∠C=180∘ ym∠B+m∠D=180∘, entoncesABCD se inscribe en⨀E.
¿Y si te dieran un círculo con un cuadrilátero inscrito en él? ¿Cómo podría usar la información sobre los arcos formados por las medidas de ángulo cuadrilátero y/o cuadrilátero para encontrar la medida de los ángulos cuadriláteros desconocidos?
Ejemplo6.15.1
-
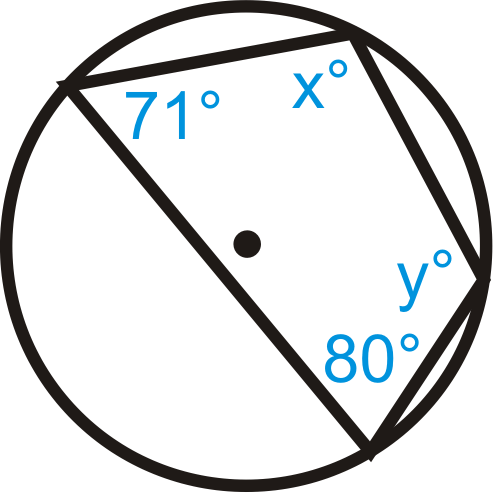
Figura6.15.3 -
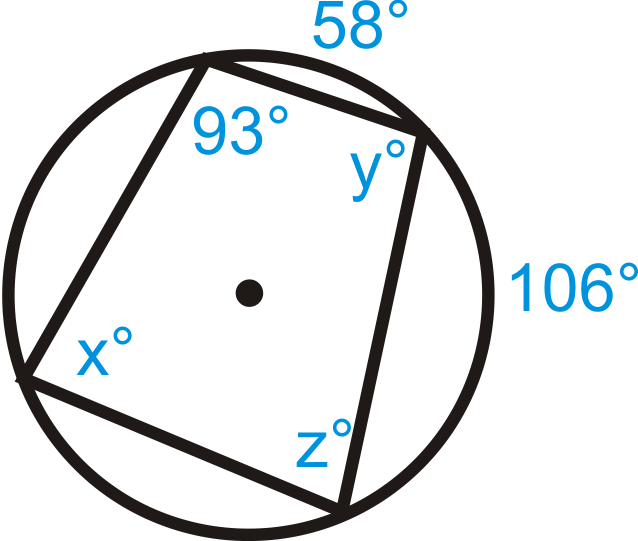
Figura6.15.4
Solución
- \ (\ begin {alineado}
x+80^ {\ circ} &=180^ {\ circ}\ qquad& y+71^ {\ circ} &=180^ {\ circ}\\
x&=100^ {\ circ} & y&=109^ {\ circ}
\ end {alineado}\)
- \ (\ begin {alineado}
z+93^ {\ circ} &=180^ {\ circ} & x&=\ frac {1} {2}\ left (58^ {\ circ} +106^ {\ circ}\ derecha) & y+82^ {\ circ} &=180^ {\ circ}\\
z &=87^ {\ circ} & x &=82^ {\ circ} & y&=98^ {\ circ}
\ final {alineado}\)
Ejemplo6.15.2
Encuentrax yy en la imagen de abajo.
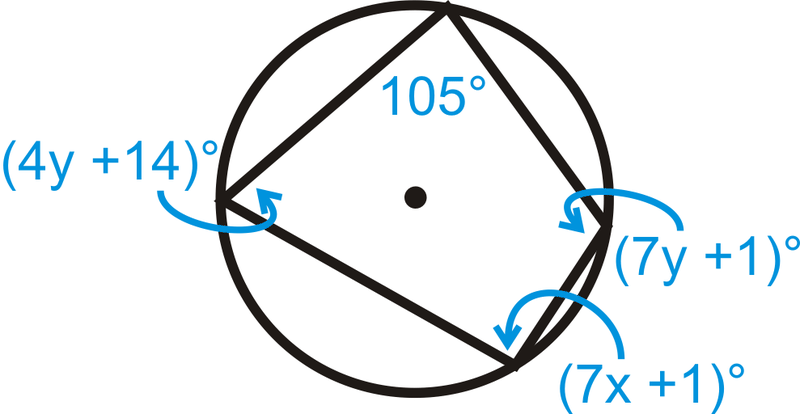
Solución
\ (\ begin {array} {rlrl}
(7 x+1) ^ {\ circ} +105^ {\ circ} & =180^ {\ circ} & (4 y+14) ^ {\ circ} + (7 y+1) ^ {\ circ} & =180^ {\ circ}\\
7 x+106^ {\ circ} & =180^ {\ circ} & 11 y+15^ {\ circ} & =180^ {\ circ}\\
7 x & =74 & 11 y & =165\\
x & ; =10.57 & y&=15
\ end {array}\)
Ejemplo6.15.3
Encuentra los valores de x e y en⨀A.
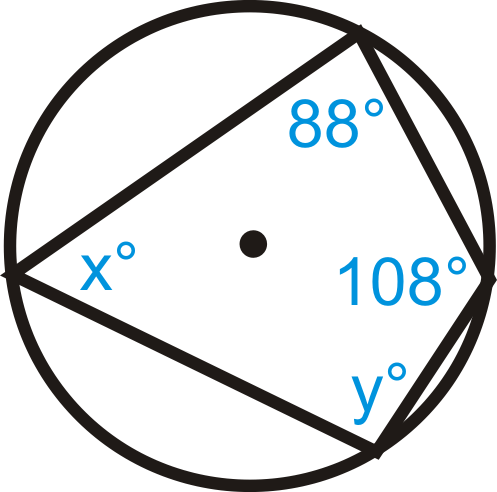
Solución
Utilice el Teorema Cuadrilátero Inscrito. x∘+108∘=180∘asíx=72∘. Del mismo modo,y∘+88∘=180∘ asíy=92∘.
Ejemplo6.15.4
CuadriláteroABCD está inscrito en⨀E. Encontrarm∠A,m∠B,m∠C, ym∠D.
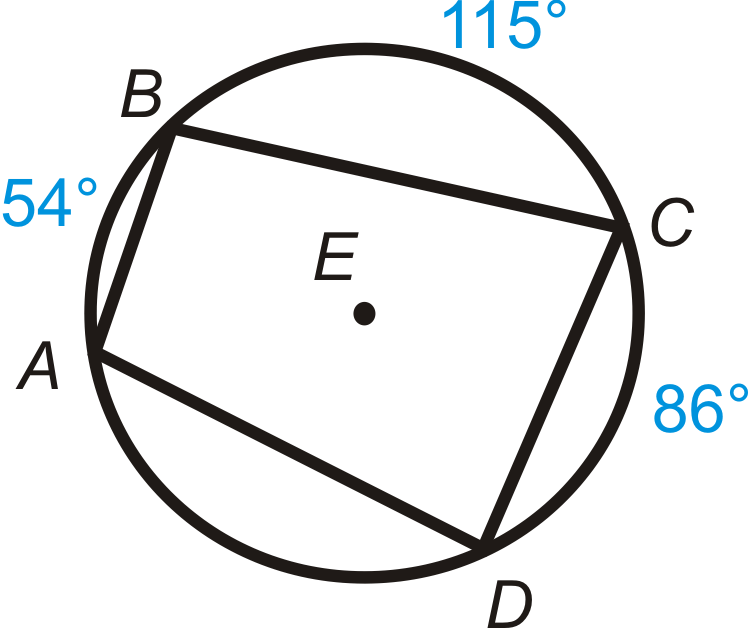
Solución
Primero, tenga en cuenta quem^AD=105∘ debido a que el círculo completo debe sumar hasta360∘.
m∠A=12m^BD=12(115+86)=100.5∘m∠B=12m^AC=12(86+105)=95.5∘m∠C=180∘−m∠A=180∘−100.5∘=79.5∘m∠D=180∘−m∠B=180∘−95.5∘=84.5∘
Revisar
Rellene los espacios en blanco.
- Un polígono (n) _______________ tiene todos sus vértices en un círculo.
- Los ángulos _____________ de un cuadrilátero inscrito son ________________.
CuadriláteroABCD está inscrito en⨀E. Encuentra:
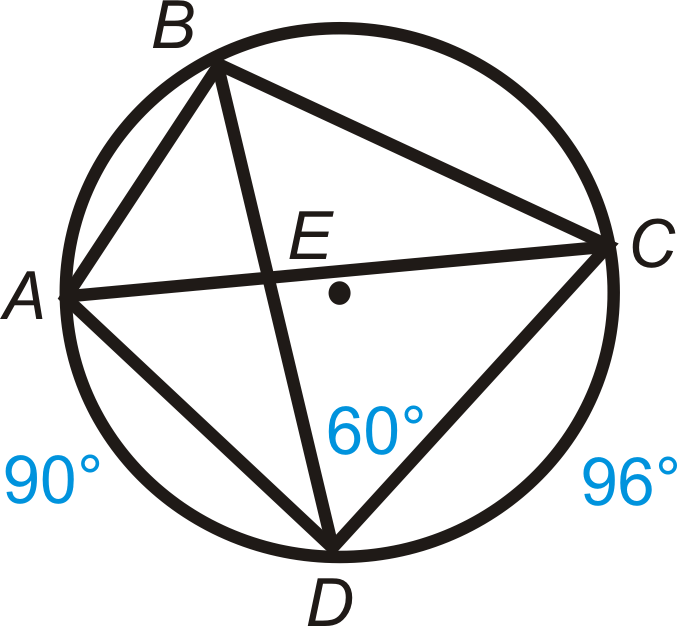
- m∠DBC
- m^BC
- m^AB
- m∠ACD
- m∠ADC
- m∠ACB
Encuentra el valor dex y/oy en⨀A.
-
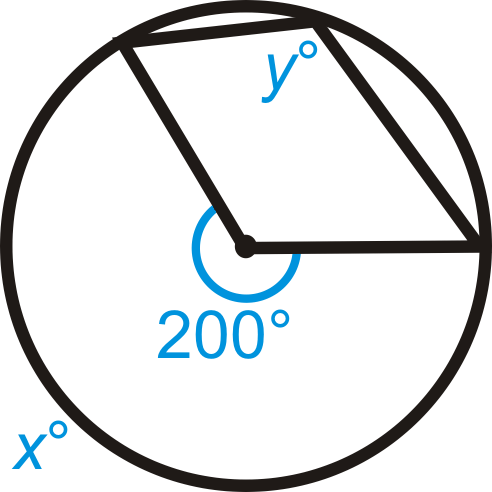
Figura6.15.9 -
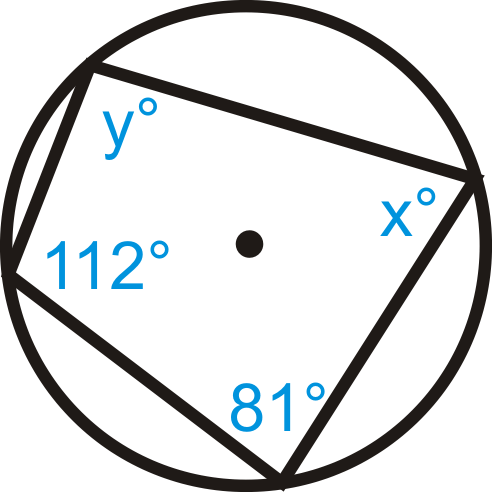
Figura6.15.10 -
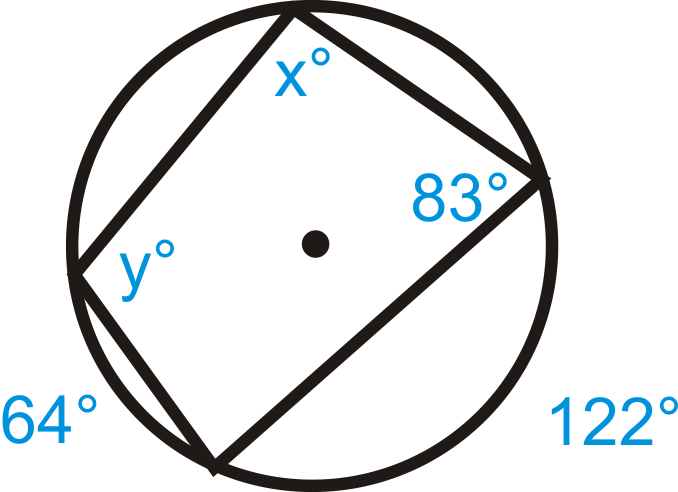
Figura6.15.11
Resolver parax.
-
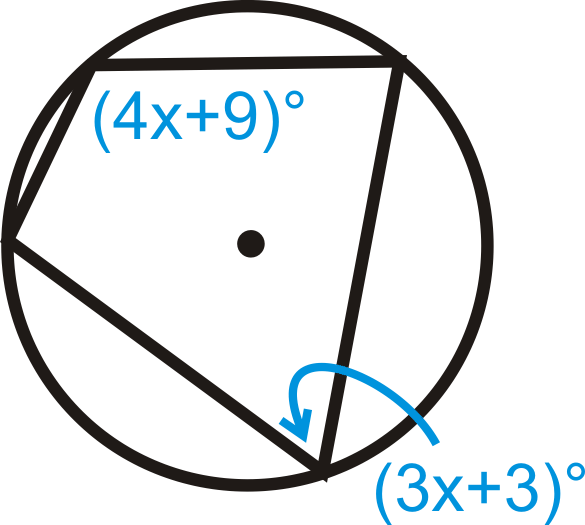
Figura6.15.12 -
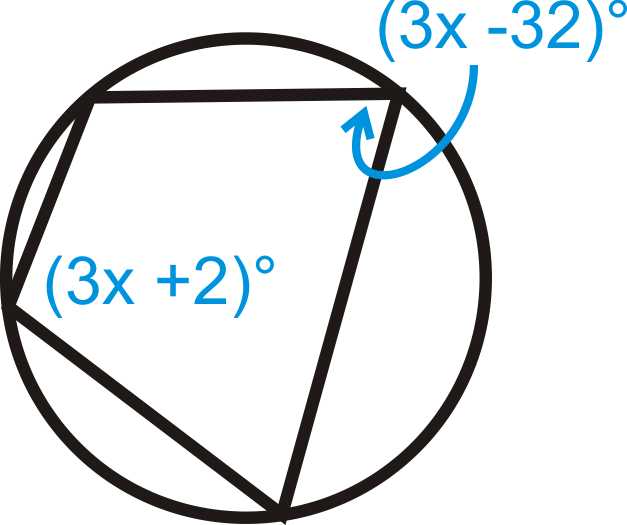
Figura6.15.13
vocabulario
| Término | Definición |
|---|---|
| ángulo central | Un ángulo formado por dos radios y cuyo vértice se encuentra en el centro del círculo. |
| acorde | Un segmento de línea cuyos extremos están en un círculo. |
| círculo | El conjunto de todos los puntos que están a la misma distancia de un punto específico, llamado el centro. |
| diámetro | Un acorde que pasa por el centro del círculo. La longitud de un diámetro es dos veces la longitud de un radio. |
| ángulo inscrito | Un ángulo con su vértice en el círculo y cuyos lados son acordes. |
| arco interceptado | El arco que se encuentra dentro de un ángulo inscrito y cuyos extremos están en el ángulo. |
| radio | La distancia desde el centro hasta el borde exterior de un círculo. |
| Polígono con Inscritos | Un polígono inscrito es un polígono con cada vértice en un círculo dado. |
| Teorema Cuadrilátero con Inscritos | El Teorema Cuadrilátero Inscrito establece que un cuadrilátero puede ser inscrito en un círculo si y sólo si los ángulos opuestos del cuadrilátero son suplementarios. |
| Cuadriláteros Cíclicos | Un cuadrilátero cíclico es un cuadrilátero que se puede inscribir en un círculo. |
Recursos adicionales
Elemento Interactivo
Video: Cuadriláteros Inscritos en Círculos Principios - Básico
Actividades: Cuadriláteros Inscritos en Círculos Preguntas de Discusión
Ayudas de estudio: Guía de estudio Inscritos en Círculos
Práctica: Cuadriláteros Inscritos en Círculos
Mundo real: Amanecer en Stonehenge


