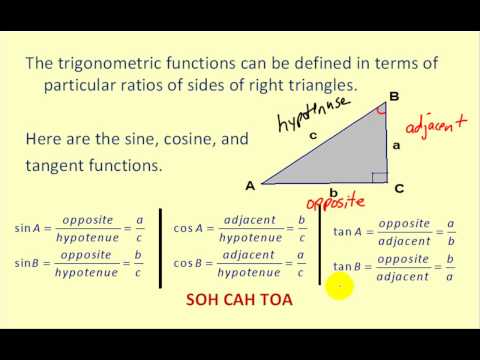
2.1.1: Trigonometría de Triángulo Recto
( \newcommand{\kernel}{\mathrm{null}\,}\)
Seno, coseno, tangente y otras relaciones de lados de un triángulo rectángulo.
Seno, coseno y tangente
La trigonometría es el estudio de las relaciones entre los lados y los ángulos de los triángulos rectos. Las patas se denominan adyacentes u opuestas dependiendo del ángulo agudo que se esté utilizando.
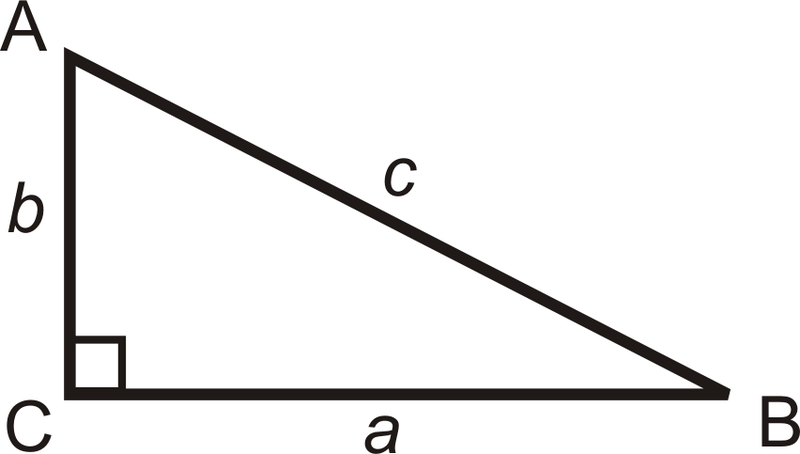
a is adjacent to ∠Ba is opposite ∠Ab is adjacent to ∠Ab is opposite ∠Bc is the hypotenuse
Las tres relaciones trigonométricas básicas se denominan seno, coseno y tangente. Para triángulo rectángulo ∆ABC, tenemos:
\ (\ begin {alineado}
\ text {seno Ratio:}\ dfrac {\ text {pierna opuesta}} {\ texto {hipotenusa}}\ qquad\ sin A=\ dfrac {a} {c}\ texto {o}\ sin B=\ dfrac {b} {c}\
\ text {coseno Ratio:}\ dfrac {\ texto {pierna adyacente}} {texto {hipotenusa}}\ qquad\ cos A=\ dfrac {b} {c}\ texto {o}\ cos B=\ dfrac {a} {c}\ \
\ texto {Relación tangente:}\ dfrac {\ texto {pierna opuesta}} {\ texto {pierna adyacente}}\ qquad\ tan A=\ dfrac {a} {b}\ texto {o}\ tan B=\ dfrac {b} {a}
\ end {alineado}\)
Una manera fácil de recordar las proporciones es usar SOH-CAH-TOA.

Algunos puntos importantes:
- Siempre reduce las proporciones (fracciones) cuando puedas.
- Usa el Teorema de Pitágoras para encontrar el lado faltante (si lo hay).
- Si hay un radical en el denominador, racionalizar el denominador.
¿Y si te dieran un triángulo rectángulo y te dijeran que sus lados miden 3, 4 y 5 pulgadas? ¿Cómo podrías encontrar el seno, el coseno y la tangente de uno de los ángulos no rectos del triángulo?
Encuentra el seno, coseno y proporciones tangentes de∠A.
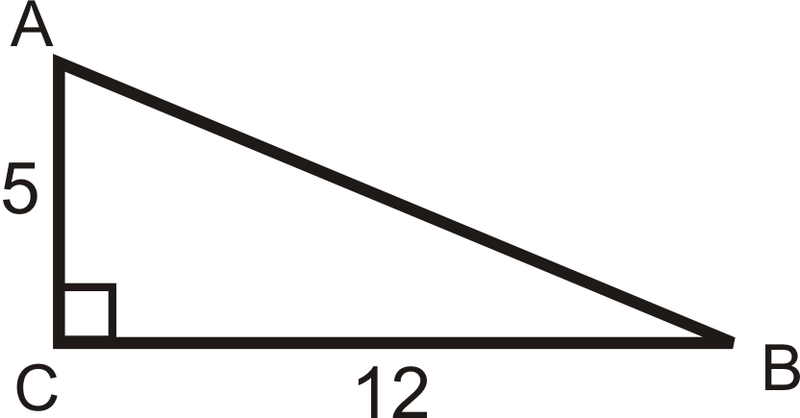
Solución
Primero, necesitamos usar el Teorema de Pitágoras para encontrar la longitud de la hipotenusa.
\ (\ begin {alineado}
5^ {2} +12^ {2} &=c^ {2}\\
13 &=c\ end {alineado}\)
\ (\ begin {alineado}
\ sin A &=\ dfrac {l e g\ texto {opuesto}\ ángulo A} {\ texto {hipotenusa}} =\ dfrac {12} {13} &\ cos A=\ dfrac {\ texto {pierna adyacente a}\ ángulo A} {\ texto {hipotenusa}} =\ dfrac {5} {13},\
\ tan\ A &=\ dfrac {\ text {pierna opuesta}\ ángulo A} {\ texto {pierna adyacente a}\ ángulo A} =\ dfrac {12} {5}
\ final {alineado}\)
Encuentra el seno, coseno, y tangente de∠B.
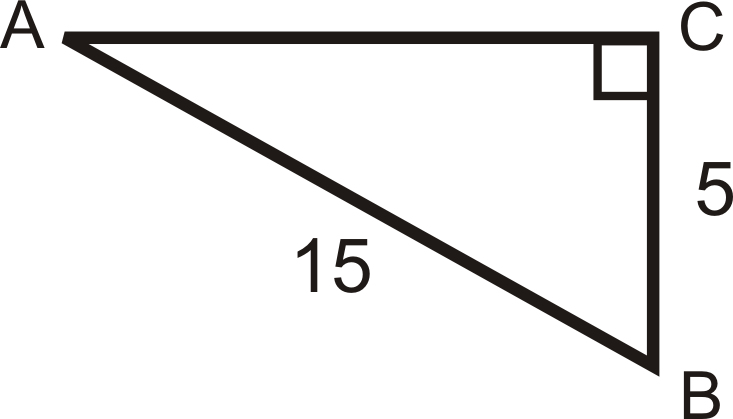
Encuentra la longitud del lado faltante.
Solución
\ (\ begin {alineado}
A C^ {2} +5^ {2} &=15^ {2}\
A C^ {2} &=200\\
A C &=10\ sqrt {2}\ end {alineado}\)
\ (\ begin {alineado}\ sin B &=\ dfrac {10\ sqrt {2}} {15} =\ dfrac {2\ sqrt {2}} {3}\ quad\ cos B=\ dfrac {5} {15} =\ dfrac {1} {3}\ quad\ tan B=\ dfrac {10\ sqrt {2}} {5} =2\ sqrt {2}
\ final {alineado}\)
Encuentra el seno, coseno y tangente de30∘.
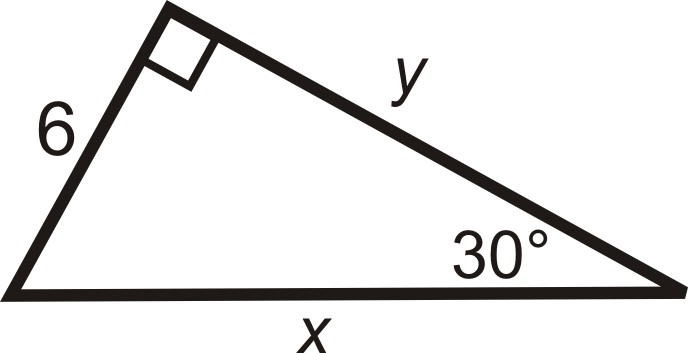
Solución
Se trata de un triángulo 30-60-90. La pierna corta es de 6,y=6√3 yx=12.
sin30∘=612=12cos30∘=6√312=√32tan30∘=66√3=1√3⋅√3√3=√33
Contesta las preguntas sobre la siguiente imagen. Reducir todas las fracciones.
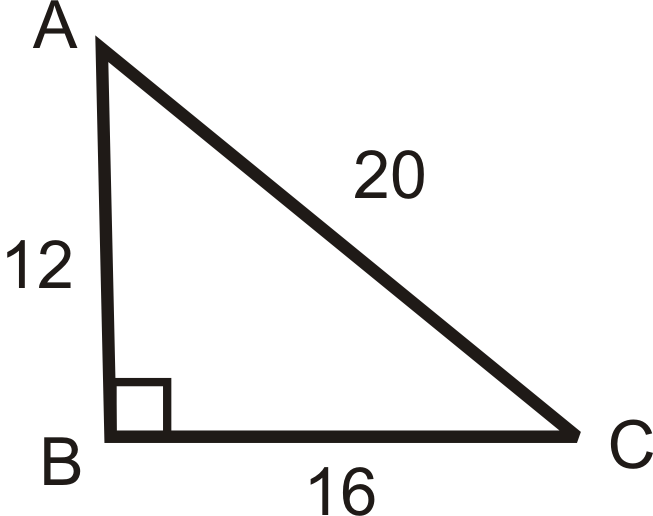
¿Qué essinAcosA, ytanA?
Solución
\ (\ begin {array} {l}
\ sin A=\ dfrac {16} {20} =\ dfrac {4} {5}\
\ cos A=\ dfrac {12} {20} =\ dfrac {3} {5}\
\ tan A=\ dfrac {16} {12} =\ dfrac {4} {3}
\ end {array}\)
Revisar
Usa el diagrama para rellenar los espacios en blanco a continuación.
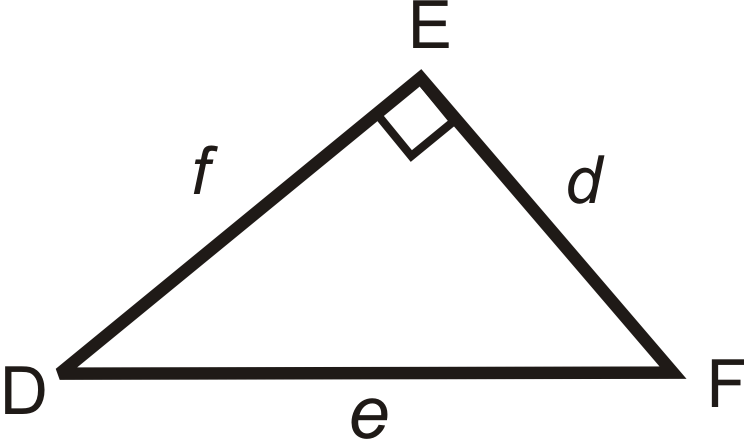
- tanD=??
- sinF=??
- tanF=??
- cosF=??
- sinD=??
- cosD=??
De las preguntas 1-6, podemos concluir lo siguiente. Rellene los espacios en blanco.
- cos_=sinFysin_=cosF.
- tanDytanF son _________ el uno del otro.
Encuentra el seno, coseno y tangente de∠A. Reducir todas las fracciones y radicales.
-

Figura2.1.1.8 -
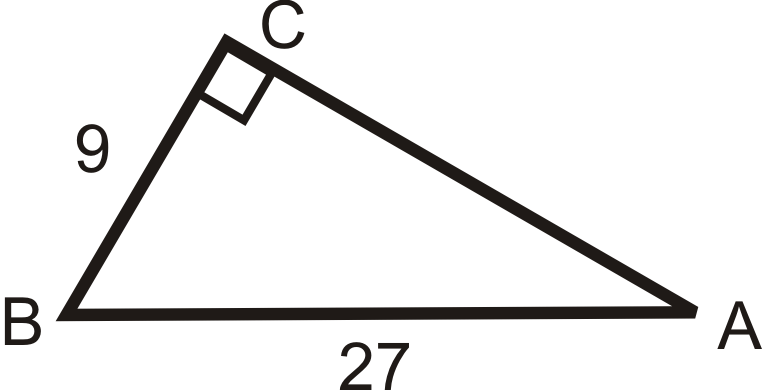
Figura2.1.1.9 -
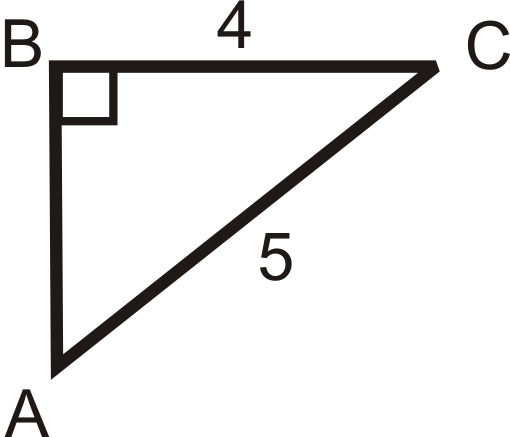
Figura2.1.1.10 -
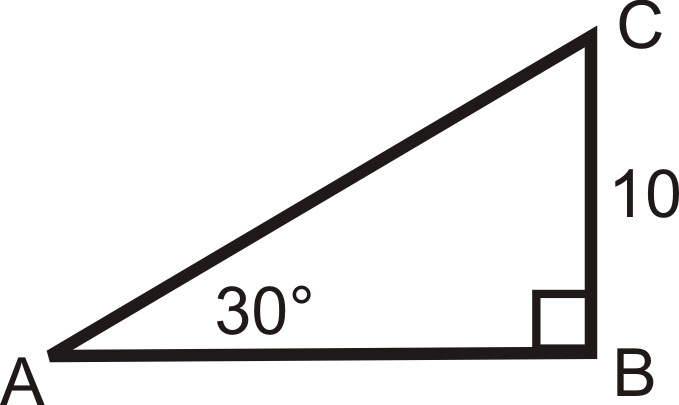
Figura2.1.1.11 -
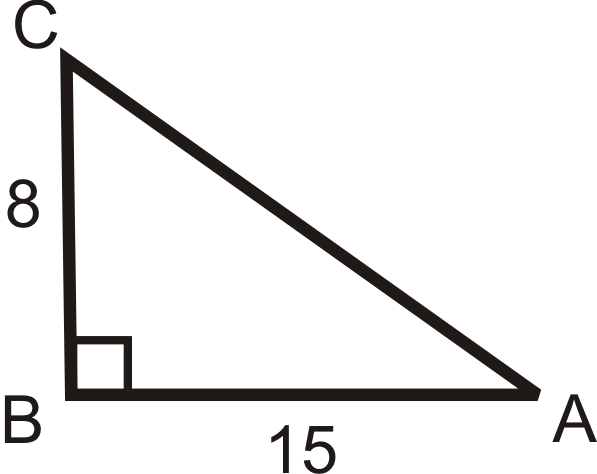 Figura\(\PageIndex{12}\)
Figura\(\PageIndex{12}\)
Reseña (Respuestas)
Para ver las respuestas de Revisar, abra este archivo PDF y busque la sección 8.7.
Recursos
El vocabulario
| Término | Definición |
|---|---|
| Ángulo Agudo | Un ángulo agudo es un ángulo con una medida de menos de 90 grados. |
| Ángulos adyacentes | Dos ángulos son adyacentes si comparten un lado y un vértice. La palabra 'adyacente' significa 'al lado' o 'al lado de'. |
| Hipotenusa | La hipotenusa de un triángulo rectángulo es el lado más largo del triángulo rectángulo. Está frente al ángulo recto. |
| Patas de un Triángulo Recto | Las patas de un triángulo rectángulo son los dos lados más cortos del triángulo rectángulo. Las patas están adyacentes al ángulo recto. |
| opuesto | Lo contrario de un númerox es−x. Un número y su opuesto siempre suman a cero. |
| Teorema de Pitágoras | El Teorema de Pitágoras es una relación matemática entre los lados de un triángulo rectánguloa2+b2=c2, dada por, donde a y b son patas del triángulo y c es la hipotenusa del triángulo. |
| Radical | El signo\boldsymbol{\sqrt}, o raíz cuadrada,. |
| seno | El seno de un ángulo en un triángulo rectángulo es un valor que se encuentra dividiendo la longitud del lado opuesto al ángulo dado por la longitud de la hipotenusa. |
| Ratios trigonométricos | Ratios que nos ayudan a subestimar las relaciones entre lados y ángulos de triángulos rectos. |
Recursos adicionales
Video: Introducción a las Funciones Trigonométricas U\ sin g Triángulos
Actividades: Seno, coseno, tangente Discusión Preguntas
Ayudas de estudio: Guía de estudio de relaciones trigonométricas
Práctica: Trigonometría de Triángulo Recto
Mundo real: Tangente de coseno