1.6: Propiedades de los números reales
- Page ID
- 112449
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Al final de esta sección, podrás:
- Utilizar las propiedades conmutativas y asociativas
- Usar las propiedades de identidad, inversa y cero
- Simplificar expresiones usando la propiedad distributiva
Utilizar las propiedades conmutativas y asociativas
El orden que añadimos dos números no afecta el resultado. Si sumamos\(8+9\) o\(9+8\), los resultados son los mismos, ambos equivalen a 17. Entonces,\(8+9=9+8\). ¡El orden en que añadimos no importa!
De igual manera, al multiplicar dos números, el orden no afecta el resultado. Si multiplicamos\(9·8\) o\(8·9\) los resultados son los mismos, ambos equivalen a 72. Entonces,\(9·8=8·9\). ¡El orden en que nos multiplicamos no importa! Estos ejemplos ilustran la Propiedad Conmutativa.
\[\begin{array}{lll} \textbf{of Addition} & \text{If }a \text{ and }b \text{are real numbers, then} & a+b=b+a. \\ \textbf{of Multiplication} & \text{If }a \text{ and }b \text{are real numbers, then} & a·b=b·a. \end{array} \]
Al sumar o multiplicar, cambiar el orden da el mismo resultado.
La Propiedad Conmutativa tiene que ver con el orden. Nosotros restamos\(9−8\) y\(8−9\), y vemos eso\(9−8\neq 8−9\). Dado que cambiar el orden de la resta no da el mismo resultado, sabemos que la resta no es conmutativa.
La división tampoco es conmutativa. Ya que\(12÷3\neq 3÷12\), cambiar el orden de la división no dio el mismo resultado. ¡Las propiedades conmutativas se aplican solo a la suma y multiplicación!
- La suma y la multiplicación son conmutativas.
- La resta y la división no son conmutativas.
Al sumar tres números, cambiar la agrupación de los números da el mismo resultado. Por ejemplo,\((7+8)+2=7+(8+2)\), ya que cada lado de la ecuación equivale a 17.
Esto también es cierto para la multiplicación. Por ejemplo,\(\left(5·\frac{1}{3}\right)·3=5·\left(\frac{1}{3}·3\right)\), ya que cada lado de la ecuación equivale a 5.
Estos ejemplos ilustran la Propiedad Asociativa.
\[\begin{array}{lll} \textbf{of Addition} & \text{If }a,b, \text{ and }c \text{ are real numbers, then} & (a+b)+c=a+(b+c). \\ \textbf{of Multiplication} & \text{If }a,b,\text{ and }c \text{ are real numbers, then} & (a·b)·c=a·(b·c). \end{array} \]
Al sumar o multiplicar, cambiar la agrupación da el mismo resultado.
La Propiedad Asociativa tiene que ver con la agrupación. Si cambiamos cómo se agrupan los números, el resultado será el mismo. Observe que son los mismos tres números en el mismo orden, la única diferencia es la agrupación.
Vimos que la resta y la división no eran conmutativas. Tampoco son asociativas.
\[\begin{array}{cc} (10−3)−2\neq 10−(3−2) & (24÷4)÷2\neq 24÷(4÷2) \\ 7−2\neq 10−1 & 6÷2\neq 24÷2 \\ 5\neq 9 & 3\neq 12 \end{array}\]
A la hora de simplificar una expresión, siempre es una buena idea planificar cuáles serán los pasos. Para combinar términos similares en el siguiente ejemplo, usaremos la Propiedad Conmutativa de adición para escribir los términos similares juntos.
Simplificar:\(18p+6q+15p+5q\).
- Contestar
-
\[\begin{array}{lc} \text{} & 18p+6q+15p+5q \\ \text{Use the Commutative Property of addition to} & 18p+15p+6q+5q \\ \text{reorder so that like terms are together.} & {} \\ \text{Add like terms.} & 33p+11q \end{array}\]
Simplificar:\(23r+14s+9r+15s\).
- Contestar
-
\(32r+29s\)
Simplificar:\(37m+21n+4m−15n\).
- Contestar
-
\(41m+6n\)
Cuando tenemos que simplificar las expresiones algebraicas, a menudo podemos facilitar el trabajo aplicando primero la Propiedad Conmutativa o la Propiedad Asociativa.
Simplificar:\((\frac{5}{13}+\frac{3}{4})+\frac{1}{4}\).
- Contestar
-
\( \begin{array}{lc} \text{} & (\frac{5}{13}+\frac{3}{4})+\frac{1}{4} \\ {\text{Notice that the last 2 terms have a common} \\ \text{denominator, so change the grouping.} } & \frac{5}{13}+(\frac{3}{4}+\frac{1}{4}) \\ \text{Add in parentheses first.} & \frac{5}{13}+(\frac{4}{4}) \\ \text{Simplify the fraction.} & \frac{5}{13}+1 \\ \text{Add.} & 1\frac{5}{13} \\ \text{Convert to an improper fraction.} & \frac{18}{13} \end{array}\)
Simplificar:\((\frac{7}{15}+\frac{5}{8})+\frac{3}{8}.\)
- Contestar
-
\(1 \frac{7}{15}\)
Simplificar:\((\frac{2}{9}+\frac{7}{12})+\frac{5}{12}\).
- Contestar
-
\(1\frac{2}{9}\)
Usar las Propiedades de Identidad, Inversa y Cero
¿Qué sucede cuando sumamos 0 a algún número? Agregar 0 no cambia el valor. Por esta razón, llamamos a 0 la identidad aditiva. La Identidad Propiedad de Adición que establece que para cualquier número real\(a,a+0=a\) y\(0+a=a.\)
¿Qué pasa cuando multiplicamos cualquier número por uno? Multiplicar por 1 no cambia el valor. Entonces llamamos a 1 la identidad multiplicativa. La Identidad Propiedad de Multiplicación que establece que para cualquier número real\(a,a·1=a\) y\(1⋅a=a.\)
Resumimos las Propiedades de Identidad aquí.
\[\begin{array}{ll} \textbf{of Addition} \text{ For any real number }a:a+0=a & 0+a=a \\ \\ \\ \textbf{0} \text{ is the } \textbf{additive identity} \\ \textbf{of Multiplication} \text{ For any real number } a:a·1=a & 1·a=a \\ \\ \\ \textbf{1} \text{ is the } \textbf{multiplicative identity} \end{array}\]
¿Qué número sumado a 5 da la identidad aditiva, 0? Sabemos
.jpg)
¡El número faltante era lo opuesto al número!
Llamamos a\(−a\) la inversa aditiva de\(a\). Lo contrario de un número es su inverso aditivo. Un número y su opuesto se suman a cero, que es la identidad aditiva. Esto lleva a la Propiedad Inversa de Adición que establece para cualquier número real\(a,a+(−a)=0.\)
¿Qué número multiplicado por\(\frac{2}{3}\) da la identidad multiplicativa, 1? En otras palabras, ¿\(\frac{2}{3}\)veces qué resulta en 1? Sabemos
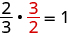
¡El número faltante era el recíproco del número!
Llamamos a\(\frac{1}{a}\) la inversa multiplicativa de a. El recíproco de un número es su inverso multiplicativo. Esto lleva a la Propiedad Inversa de Multiplicación que establece que para cualquier número real\(a,a\neq 0,a·\frac{1}{a}=1.\)
Aquí declararemos formalmente las propiedades inversas.
\[\begin{array}{lc} \textbf{of addition} \text{For any real number }a, & a+(−a)=0 \\ \;\;\;\; −a \text{ is the } \textbf{additive inverse }\text{ of }a & {} \\ \;\;\;\; \text{A number and its } \textit{opposite } \text{add to zero.} \\ \\ \\ \textbf{of multiplication } \text{For any real number }a,a\neq 0 & a·\dfrac{1}{a}=1 \\ \;\;\;\;\;\dfrac{1}{a} \text{ is the } \textbf{multiplicative inverse} \text{ of }a \\ \;\;\;\; \text{A number and its } \textit{reciprocal} \text{ multiply to one.} \end{array}\]
El Propiedad Identidad de suma dice que cuando sumamos 0 a cualquier número, el resultado es ese mismo número. ¿Qué sucede cuando multiplicamos un número por 0? Multiplicar por 0 hace que el producto sea igual a cero.
¿Qué pasa con la división que involucra cero? ¿Qué es\(0÷3\)? Piensa en un ejemplo real: Si no hay galletas en el tarro de galletas y 3 personas están para compartirlas, ¿cuántas galletas obtiene cada persona? No hay cookies para compartir, por lo que cada persona obtiene 0 cookies. Entonces,\(0÷3=0.\)
Podemos verificar la división con el hecho de multiplicación relacionado. Entonces sabemos\(0÷3=0\) porque\(0·3=0\).
Ahora piensa en dividir por cero. ¿Cuál es el resultado de dividir 4 por 0? Piense en el hecho de multiplicación relacionado:
¿Hay un número que multiplicado por 0 da 4? Dado que cualquier número real multiplicado por 0 da 0, no hay un número real que pueda multiplicarse por 0 para obtener 4. Concluimos que no hay respuesta a\(4÷0\) y así decimos que la división por 0 es indefinida.
Resumimos las propiedades de cero aquí.
Multiplicación por Cero: Para cualquier número real a,
\[a⋅0=0 \; \; \; 0⋅a=0 \; \; \; \; \text{The product of any number and 0 is 0.}\]
División por Cero: Para cualquier número real a,\(a\neq 0\)
\[\begin{array}{cl} \dfrac{0}{a}=0 & \text{Zero divided by any real number, except itself, is zero.} \\ \dfrac{a}{0} \text{ is undefined} & \text{Division by zero is undefined.} \end{array}\]
Ahora vamos a practicar el uso de las propiedades de identidades, inversos y cero para simplificar las expresiones.
Simplificar:\(−84n+(−73n)+84n.\)
- Contestar
-
\(\begin{array}{lc} \text{} & −84n+(−73n)+84n \\ \text{Notice that the first and third terms are} \\ \text{opposites; use the Commutative Property of} & −84n+84n+(−73n) \\ \text{addition to re-order the terms.} \\ \text{Add left to right.} & 0+(−73n) \\ \text{Add.} & −73n \end{array}\)
Simplificar:\(−27a+(−48a)+27a\).
- Contestar
-
\(−48a\)
Simplificar:\(39x+(−92x)+(−39x)\).
- Contestar
-
\(−92x\)
Ahora veremos cómo es útil reconocer los recíprocos. Antes de multiplicar de izquierda a derecha, busque reciprocales—su producto es 1.
Simplificar:\(\frac{7}{15}⋅\frac{8}{23}⋅\frac{15}{7}\).
- Contestar
-
\(\begin{array}{lc} \text{} & \frac{7}{15}⋅\frac{8}{23}⋅\frac{15}{7} \\ \text{Notice the first and third terms} \\ {\text{are reciprocals, so use the Commutative} \\ \text{Property of multiplication to re-order the} \\ \text{factors.}} & \frac{7}{15}·\frac{15}{7}·\frac{8}{23} \\ \text{Multiply left to right.} & 1·\frac{8}{23} \\ \text{Multiply.} & \frac{8}{23} \end{array}\)
Simplificar:\(\frac{9}{16}⋅\frac{5}{49}⋅\frac{16}{9}\).
- Contestar
-
\(\frac{5}{49}\)
Simplificar:\(\frac{6}{17}⋅\frac{11}{25}⋅\frac{17}{6}\).
- Contestar
-
\(\frac{11}{25}\)
El siguiente ejemplo nos hace conscientes de la distinción entre dividir 0 por algún número o algún número dividido por 0.
Simplificar: a.\(\frac{0}{n+5}\), donde\(n\neq −5\) b.\(\frac{10−3p}{0}\) donde\(10−3p\neq 0.\)
- Contestar
-
a.
\(\begin{array}{lc} {} & \dfrac{0}{n+5} \\ \text{Zero divided by any real number except itself is 0.} & 0 \end{array}\)
b.
\(\begin{array}{lc} {} & \dfrac{10−3p}{0} \\ \text{Division by 0 is undefined.} & \text{undefined} \end{array}\)
Simplificar: a.\(\frac{0}{m+7}\), donde\(m\neq −7\) b.\(\frac{18−6c}{0}\), donde\(18−6c\neq 0\).
- Contestar
-
a. 0
b. indefinido
Simplificar: a.\(\frac{0}{d−4}\), donde\(d\neq 4\) b.\(\frac{15−4q}{0}\), donde\(15−4q\neq 0\).
- Contestar
-
a. 0
b. indefinido
Simplificar expresiones mediante la propiedad distributiva
Supongamos que tres amigos van al cine. Cada uno necesita $9.25, es decir, 9 dólares y 1 trimestre, para pagar sus boletos. ¿Cuánto dinero necesitan todos juntos?
Se puede pensar en los dólares por separado de los trimestres. Necesitan 3 veces $9 entonces $27 y 3 veces 1 trimestre, entonces 75 centavos. En total, necesitan 27.75 dólares. Si piensas en hacer las matemáticas de esta manera, estás usando la Propiedad Distributiva.
\(\begin{array}{lc} \text{If }a,b \text{,and }c \text{are real numbers, then} \; \; \; \; \; & a(b+c)=ab+ac \\ {} & (b+c)a=ba+ca \\ {} & a(b−c)=ab−ac \\{} & (b−c)a=ba−ca \end{array}\)
En álgebra, utilizamos la Propiedad Distributiva para eliminar paréntesis a medida que simplificamos las expresiones.
Simplificar:\(3(x+4)\).
- Contestar
-
\(\begin{array} {} & 3(x+4) \\ \text{Distribute.} \; \; \; \; \; \; \; \; & 3·x+3·4 \\ \text{Multiply.} & 3x+12 \end{array}\)
Simplificar:\(4(x+2)\).
- Contestar
-
\(4x8\)
Simplificar:\(6(x+7)\).
- Contestar
-
\(6x42\)
A algunos estudiantes les resulta útil dibujar flechas para recordarles cómo usar la Propiedad Distributiva. Entonces el primer paso en Ejemplo se vería así:
Simplificar:\(8(\frac{3}{8}x+\frac{1}{4})\).
- Contestar
-
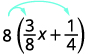
Distribuir.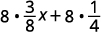 
Multiplicar.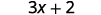 
Simplificar:\(6(\frac{5}{6}y+\frac{1}{2})\).
- Contestar
-
\(5y+3\)
Simplificar:\(12(\frac{1}{3}n+\frac{3}{4})\)
- Contestar
-
\(4n+9\)
Usar la Propiedad Distributiva como se muestra en el siguiente ejemplo será muy útil cuando resolvamos aplicaciones de dinero en capítulos posteriores.
Simplificar:\(100(0.3+0.25q)\).
- Contestar
-
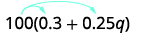
Distribuir.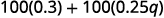 
Multiplicar. 
Simplificar:\(100(0.7+0.15p).\)
- Contestar
-
\(70+15p\)
Simplificar:\(100(0.04+0.35d)\).
- Contestar
-
\(4+35d\)
Cuando distribuimos un número negativo, ¡debemos tener mucho cuidado para que las señales sean correctas!
Simplificar:\(−11(4−3a).\)
- Contestar
-
\(\begin{array}{lc} {} & −11(4−3a) \\ \text{Distribute. } \; \; \; \; \; \; \; \; \; \;& −11·4−(−11)·3a \\ \text{Multiply.} & −44−(−33a) \\ \text{Simplify.} & −44+33a \end{array}\)
Observe que también podría escribir el resultado como\(33a−44.\) ¿Sabes por qué?
Simplificar:\(−5(2−3a)\).
- Contestar
-
\(−10+15a\)
Simplificar:\(−7(8−15y).\)
- Contestar
-
\(−56+105y\)
En el siguiente ejemplo, mostraremos cómo usar la Propiedad Distributiva para encontrar lo contrario de una expresión.
Simplificar:\(−(y+5)\).
- Contestar
-
\(\begin{array}{lc} {} & −(y+5) \\ \text{Multiplying by }−1 \text{ results in the opposite.}& −1(y+5) \\ \text{Distribute.} & −1·y+(−1)·5 \\ \text{Simplify.} & −y+(−5) \\ \text{Simplify.} & −y−5 \end{array} \)
Simplificar:\(−(z−11)\).
- Contestar
-
\(−z+11\)
Simplificar:\(−(x−4)\).
- Contestar
-
\(−x+4\)
Habrá momentos en los que necesitaremos usar la Propiedad Distributiva como parte del orden de operaciones. Empieza por mirar los paréntesis. Si no se puede simplificar la expresión dentro de los paréntesis, el siguiente paso sería multiplicar usando la Propiedad Distributiva, que elimina los paréntesis. Los siguientes dos ejemplos ilustrarán esto.
Simplificar:\(8−2(x+3)\)
- Contestar
-
Seguimos el orden de las operaciones. La multiplicación viene antes de la resta, por lo que distribuiremos primero los 2 y luego restaremos.
\(\begin{array}{lc} {} & \text{8−2(x+3)} \\ \text{Distribute.} & 8−2·x−2·3 \\ \text{Multiply.} & 8−2x−6 \\ \text{Combine like terms.} &−2x+2 \end{array}\)
Simplificar:\(9−3(x+2)\).
- Contestar
-
\(3−3x\)
Simplificar:\(7x−5(x+4)\).
- Contestar
-
\(2x−20\)
Simplificar:\(4(x−8)−(x+3)\).
- Contestar
-
\(\begin{array}{lc} {} & 4(x−8)−(x+3) \\ \text{Distribute.} & 4x−32−x−3 \\ \text{Combine like terms.} & 3x−35 \end{array}\)
Simplificar:\(6(x−9)−(x+12)\).
- Contestar
-
\(5x−66\)
Simplificar:\(8(x−1)−(x+5)\).
- Contestar
-
\(7x−13\)
Aquí se resumen todas las propiedades de los números reales que hemos utilizado en este capítulo.
| Propiedad conmutativa
Al sumar o multiplicar, cambiar el orden da el mismo resultado \[\begin{array}{lll} \textbf{of Addition} & \text{If }a \text{ and }b \text{are real numbers, then} & a+b=b+a. \\ \textbf{of Multiplication} & \text{If }a \text{ and }b \text{are real numbers, then} & a·b=b·a. \end{array} \] |
| Propiedad asociativa
Al sumar o multiplicar, cambiar la agrupación da el mismo resultado. \[\begin{array}{lll} \textbf{of Addition} & \text{If }a,b, \text{ and }c \text{ are real numbers, then} & (a+b)+c=a+(b+c). \\ \textbf{of Multiplication} & \text{If }a,b,\text{ and }c \text{ are real numbers, then} & (a·b)·c=a·(b·c). \end{array} \] |
| Propiedad distributiva
\[\begin{array}{lc} \text{If }a,b \text{,and }c \text{are real numbers, then} \; \; \; \; \; & a(b+c)=ab+ac \\ {} & (b+c)a=ba+ca \\ {} & a(b−c)=ab−ac \\{} & (b−c)a=ba−ca \end{array}\] |
| Propiedad Identidad \[\begin{array}{ll} \textbf{of Addition} \text{ For any real number }a:a+0=a & 0+a=a \\ \;\;\;\; \textbf{0} \text{ is the } \textbf{additive identity} \\ \textbf{of Multiplication} \text{ For any real number } a:a·1=a & 1·a=a \\ \;\;\;\; \textbf{1} \text{ is the } \textbf{multiplicative identity} \end{array}\] |
| Inverse (propiedad)
\[\begin{array}{lc} \textbf{of addition } \text{For any real number }a, & a+(−a)=0 \\ \;\;\;\; −a \text{ is the } \textbf{additive inverse }\text{ of }a & {} \\ \;\;\;\; \text{A number and its } \textit{opposite } \text{add to zero.} \\ \\ \\ \textbf{of multiplication } \text{For any real number }a,a\neq 0 & a·\dfrac{1}{a}=1 \\ \;\;\;\;\;\dfrac{1}{a} \text{ is the } \textbf{multiplicative inverse} \text{ of }a \\ \;\;\;\; \text{A number and its } \textit{reciprocal} \text{ multiply to one.} \end{array}\] |
| Inmuebles de Zero \[\begin{array}{lc} \text{For any real number }a, & a·0=0 \\ {} & 0·a=0 \\ \text{For any real number }a,a\neq 0, & \dfrac{0}{a}=0 \\ \text{For any real number }a, & \dfrac{a}{0} \text{ is undefined} \end{array}\] |
Conceptos clave
| Propiedad conmutativa Al agregar o multiplicar, cambiar el orden da el mismo resultado \[\begin{array}{lll} \textbf{of Addition} & \text{If }a \text{ and }b \text{are real numbers, then} & a+b=b+a. \\ \textbf{of Multiplication} & \text{If }a \text{ and }b \text{are real numbers, then} & a·b=b·a. \end{array} \] |
| Propiedad asociativa Al agregar o multiplicar, cambiar la agrupación da el mismo resultado. \[\begin{array}{lll} \textbf{of Addition} & \text{If }a,b, \text{ and }c \text{ are real numbers, then} & (a+b)+c=a+(b+c). \\ \textbf{of Multiplication} & \text{If }a,b,\text{ and }c \text{ are real numbers, then} & (a·b)·c=a·(b·c). \end{array} \] |
| Propiedad distributiva
\[\begin{array}{lc} \text{If }a,b \text{,and }c \text{are real numbers, then} \; \; \; \; \; & a(b+c)=ab+ac \\ {} & (b+c)a=ba+ca \\ {} & a(b−c)=ab−ac \\{} & (b−c)a=ba−ca \end{array}\] |
| Propiedad de Identidad
\[\begin{array}{ll} \textbf{of Addition} \text{ For any real number }a:a+0=a & 0+a=a \\ \;\;\;\; \textbf{0} \text{ is the } \textbf{additive identity} \\ \textbf{of Multiplication} \text{ For any real number } a:a·1=a & 1·a=a \\ \;\;\;\; \textbf{1} \text{ is the } \textbf{multiplicative identity} \end{array}\] |
| Inverse (propiedad)
\[\begin{array}{lc} \textbf{of addition} \text{For any real number }a, & a+(−a)=0 \\ \;\;\;\; −a \text{ is the } \textbf{additive inverse }\text{ of }a & {} \\ \;\;\;\; \text{A number and its } \textit{opposite } \text{add to zero.} \\ \\ \\ \textbf{of multiplication } \text{For any real number }a,a\neq 0 & a·\dfrac{1}{a}=1 \\ \;\;\;\;\;\dfrac{1}{a} \text{ is the } \textbf{multiplicative inverse} \text{ of }a \\ \;\;\;\; \text{A number and its } \textit{reciprocal} \text{ multiply to one.} \end{array}\] |
| Inmuebles de Zero
\[\begin{array}{lc} \text{For any real number }a, & a·0=0 \\ {} & 0·a=0 \\ \text{For any real number }a,a\neq 0, & \dfrac{0}{a}=0 \\ \text{For any real number }a, & \dfrac{a}{0} \text{ is undefined} \end{array}\] |
Glosario
- identidad aditiva
- El número 0 es la identidad aditiva porque sumar 0 a cualquier número no cambia su valor.
- inversa aditiva
- Lo contrario de un número es su inverso aditivo.
- identidad multiplicativa
- El número 1 es la identidad multiplicativa porque multiplicar 1 por cualquier número no cambia su valor.
- inversa multiplicativa
- El recíproco de un número es su inverso multiplicativo.