10.4: Método de inversión

Aquí hay una aplicación de inversión, que incluimos como ilustración; no la usaremos más en el libro.

Teorema$\PageIndex{1}$ Ptolemy's identity.

Dejado$ABCD$ ser un cuadriángulo inscrito. Supongamos que los puntos$A,B,C$, y$D$ aparecen en la circlina en el mismo orden. Entonces

$AB \cdot CD + BC \cdot DA = AC \cdot BD.$

Prueba

Supongamos que los puntos se$A,B,C,D$ encuentran en una línea en este orden.

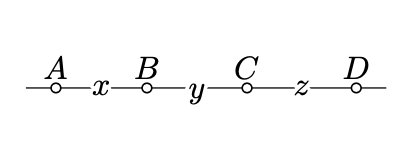
Establecer$x = AB$,$y = BC$,$z = CD$. Tenga en cuenta que

$x \cdot z + y \cdot (x + y + z) = (x + y) \cdot (y + z).$

Ya que$AC = x + y$$BD = y + z$,$DA = x + y + z$, y, prueba la identidad.

Queda por considerar el caso cuando el cuadriángulo$ABCD$ está inscrito en un círculo, digamos$\Gamma$.

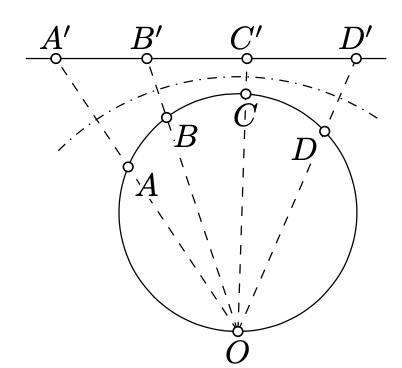
La identidad puede ser reescrita como

$\dfrac{AB \cdot DC}{BD \cdot CA} + \dfrac{BC \cdot AD}{CA \cdot DB} = 1.$

En el lado izquierdo tenemos dos ratios cruzados. Según el Teorema 10.3.1a, el lado izquierdo no cambia si aplicamos una inversión a cada punto.

Considera una inversión en un círculo centrado en un punto$O$ que se encuentra$\Gamma$ entre$A$ y$D$. Por Teorema Teorema 10.3.2, esta inversión se mapea$\Gamma$ a una línea. Esto reduce el problema al caso cuando$A, B, C$, y se$D$ encuentran en una línea, que ya se consideró.

En la prueba anterior, reescribimos la identidad de Tolomeo en una forma que es invariante con respecto a la inversión para luego aplicar una inversión que hace evidente la afirmación. La solución del siguiente ejercicio se basa en la misma idea; hay que hacer una elección correcta de inversión.

Ejercicio$\PageIndex{1}$

Supongamos que cuatro círculos son mutuamente tangentes entre sí. Demostrar que cuatro (entre seis) de sus puntos de tangencia se encuentran en una circlina.

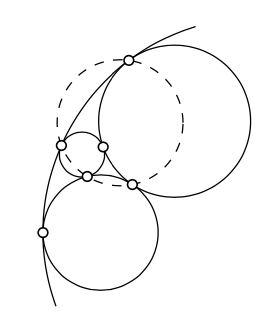
Insinuación

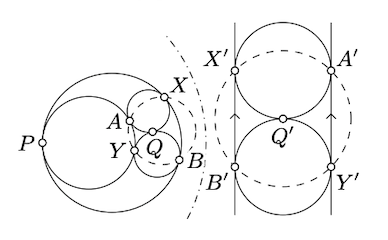
Etiquetar los puntos de tangencia por$X, Y , A, B, P$, y$Q$ como en el diagrama. Aplicar una inversión con el centro en$P$. Observe que los dos círculos que tangentes en$P$ se convierten en líneas paralelas y los dos círculos restantes son tangentes entre sí y estas dos líneas paralelas.

Tenga en cuenta que los puntos de tangencia$A'$$B'$$X'$,,, y$Y'$ con las líneas paralelas son vértices de un cuadrado; en particular se encuentran en un círculo. Estos puntos son imágenes de$A, B, X$, y$Y$ bajo la inversión. Por Teorema Teorema 10.3.1, los puntos$A, B, X$, y$Y$ también se encuentran en una circlina.

Ejercicio$\PageIndex{2}$

Supongamos que tres círculos tangentes entre sí y a dos líneas paralelas como se muestra en la imagen.

Mostrar que la línea que pasa a través$A$ y también$B$ es tangente a dos círculos en$A$.

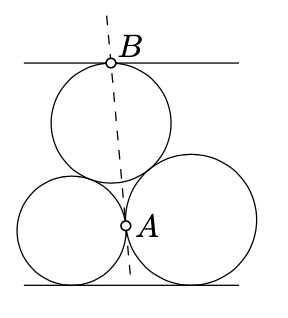
Insinuación

Aplicar la inversión en un círculo con centro$A$. El punto$A$ irá al infinito, los dos círculos tangentes en se$A$ convertirán en líneas paralelas y las dos líneas paralelas se convertirán en círculos tangentes en$A$; ver el diagrama.

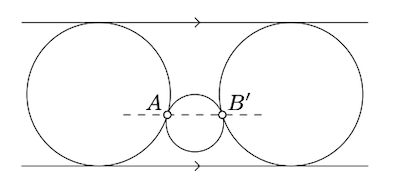
Queda por mostrar que la línea discontinua ($AB'$) es paralela a las otras dos líneas.