20.8: Método de área
( \newcommand{\kernel}{\mathrm{null}\,}\)
En esta sección daremos ejemplos de pruebas delgadas utilizando las propiedades del área. Tenga en cuenta que estas pruebas no son realmente elementales ya que el precio que se paga para introducir la función de área es alto.
Comenzamos con la prueba del teorema de Pitágoras. En los Elementos de Euclides, el teorema de Pitágoras se formuló como igualdad 20.8.1 a continuación y la prueba utilizó una técnica similar.

Comprobante. Tenemos que demostrar que sia yb son piernas yc es la hipotenusa de un triángulo rectángulo, entonces
a2+b2=c2.
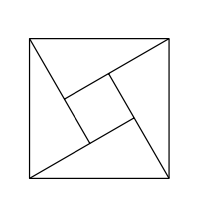
Supongamos queT denota el triángulo recto sólido con patasab y yKx el cuadrado sólido con ladox.
Construyamos dos subdivisiones deKa+b:
- Ka+bSubdividir en dos cuadrados sólidos congruentes conKa yKb y 4 triángulos sólidos congruentes conT, ver el primer diagrama.
- Ka+bSubdividir en un cuadrado sólido congruenteKc y 4 triángulos rectos sólidos congruentes conT, ver el segundo diagrama.
Aplicando la Proposición 20.4.2 pocas veces, lo conseguimos
area Ka+b=area Ka+area Kb+4⋅area T==area Kc+4⋅area T.
Por lo tanto,
area Ka+area Kb=area Kc.
Por Teorema 20.5.1,
area Kx=x2,
para cualquierx>0. De ahí sigue el enunciado.
Construir otra prueba del teorema de Pitágoras basada en el diagrama.
(En las anotaciones anteriores se muestra una subdivisión deKc enKa−b y cuatro copias deT ifa>b.)
- Pista
-
Suponiendoa>b,Qc subdividimos enQa−b y cuatro triángulos congruentes aT. Por lo tanto
area Qc=area Qa−b+4⋅area T.
Según el Teorema 20.7.1,area T=12⋅a⋅b. Por lo tanto, la identidad 20.8.2 puede escribirse como
c2=(a−b)2+2⋅a⋅b.
Simplificando, obtenemos el teorema de Pitágoras.
El casoa=b es aún más sencillo. El caso seb>a puede hacer de la misma manera.
Mostrar que la suma de distancias desde un punto a los lados de un triángulo equilátero es la misma para todos los puntos dentro del triángulo.
- Pista
-
SiX es un punto dentro de△ABC, entonces▴ABC se subdivide en▴ABX,▴BCX, y▴CAX. Por lo tanto
area (▴ABX)+area (▴BCX)+area (▴CAX)=area (▴ABC).
Seta=AB=BC=CA. Dejarh1,h2, yh3 denotar las distancias deX a los lados[AB],[BC], y[CA]. Entonces por el Teorema 20.7.1,
area (▴ABX)=12⋅h1⋅a,area (▴BCX)=12⋅h2⋅a,area (▴CAX)=12⋅h3⋅a.
Por lo tanto,
h1+h2+h3=2a⋅area (▴ABC).
Supongamos que dos triángulosABC yA′B′C′ en el plano euclidiano tienen altitudes iguales caídas desdeA yA′ respectivamente. Entonces
area (▴A′B′C′)area (▴ABC)=B′C′BC.
En particular, la misma identidad sostiene siA=A′ y las bases[BC] y[B′C′] se encuentran en una línea.
- Prueba
-
Deja queh sea la altitud. Por Teorema 20.7.1,
area (▴A′B′C′)area (▴ABC)=12⋅h⋅B′C′12⋅h⋅BC=B′C′BC.
Ahora vamos a mostrar cómo usar esta afirmación para probar Lemma 8.4.1. Primero, recordemos su declaración:
Si no△ABC es degenerado y su ángulo bisectriz en lasA intersectas[BC] y el puntoD. Entonces
ABAC=DBDC.
- Prueba
-
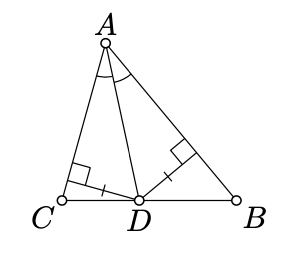
Aplicando Reclamación20.8.1, lo conseguimos
area (▴ABD)area (▴ACD)=BDCD.
Por Proposición 8.10 los triángulosABD yACD tienen altitudes iguales desdeD. Aplicando Reclamación de20.8.1 nuevo, lo conseguimos
area (▴ABD)area (▴ACD)=ABAC.
y de ahí el resultado.
Supongamos queABC es un triángulo no degenerado yA′ se encuentra entreB yC. En este caso el segmento de línea[AA′] se llama ceviano (lleva el nombre de Giovanni Ceva y se pronuncia como cheviano.) de△ABC atA. El segundo enunciado en el siguiente ejercicio se denomina teorema de Ceva.
DejarABC ser un triángulo no degenerado. Supongamos sus cevianos[AA′],[BB′] y se[CC′] cruzan en un puntoX. Demostrar que
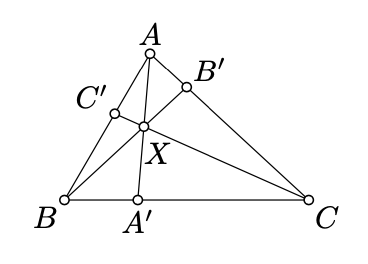
area (▴ABX)area (▴BCX)=AB′B′C,area (▴BCX)area (▴CAX)=BC′C′A,area (▴CAX)area (▴ABX)=CA′A′B.
Concluir que
AB′⋅CA′⋅BC′B′C⋅A′B⋅C′A=1.
- Pista
-
Aplicar Reclamación20.8.1 para demostrar que
area (▴ABB′)area (▴BCB′)=area (▴AXB′)area (▴XCB′)=AB′B′C.
Y observa que
area (▴ABB′)=area (▴ABX)+area (▴AXB′),
area (▴BCB′)=area (▴BCX)+area (▴XCB′).Implica la primera identidad; el resto es análogo.
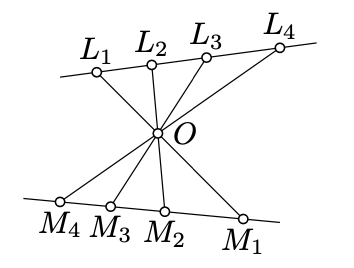
Supongamos que los puntosL1L2,L3,,L4 se encuentran en una líneaℓ y los puntosM1M2,,M3,M4 se encuentran en una línea m. Supongamos que las líneas(L1M1)(L2M2),(L3M3),, y(L4M4) pasan por un puntoO que no se encuentra sobreℓ nim.
- Aplicar Reclamación20.8.1 para demostrar esoarea ▴OLiLjarea ▴OMiMj=OLi⋅OLjOMi⋅OMj para cualquieri≠j.
- Utilizar (a) para demostrarL1L2⋅L3L4L2L3⋅L4L1=M1M2⋅M3M4M2M3⋅M4M1; que es decir, los cuatriples(L1,L2,L3,L4) y(M1,M2,M3,M4) tienen la misma relación cruzada.
- Pista
-
Para probar (a), aplicar Reclamar20.8.1 dos veces a los triángulosOLiLj,OLjMi, yOMiMj.
Para probar la parte (b), use20.8.1 Reclamar para reescribir el lado izquierdo usando las áreas de triángulosOL1L2,OL2L3,OL3L4, yOL4L1. Además, use la parte (a) para reescribirla usando áreas deOM1M2,OM2M3,OM3M4,OM4M1 y aplique Reclamar20.8.1 nuevamente para obtener el lado derecho.

