2.6: Tareas
- Page ID
- 113195
- Envíe la tarea por separado de este libro de trabajo y grapa todas las páginas juntas. (Un elemento básico para la presentación completa de toda la tarea de la unidad)
- Inicie un nuevo módulo en la parte frontal de una nueva página y escriba el número de módulo en la parte superior central de la página.
- Las respuestas sin apoyar el trabajo no recibirán crédito.
- Algunas soluciones se dan en el manual de soluciones.
- Puedes trabajar con compañeros de clase pero hacer tu propio trabajo.
Por cada dos conjuntos anotados, indique si los conjuntos coinciden o no. Si lo hacen, mostrar una coincidencia. Si no lo hacen, explique por qué no.
a. Pequeños bloques A azules y grandes bloques A rojos
b. bloques A amarillos y bloques A circulares
Describir una coincidencia entre el conjunto de números de conteo, {1, 2, 3,...} y el conjunto de múltiplos positivos de cinco, {5, 10, 15,...}.
Muestra cada correspondencia uno a uno posible entre los bloques A pequeños azules y los pequeños bloques A rojos. Usa abreviaturas o imágenes para denotar los bloques.
Convierte cada numeral a un numeral Hindu-Árabe Base Diez.
| a. apoplejía: | | | | | | | | | | | | | | | |
| b. Contador:\(\cancel{||||}\cancel{||||}\cancel{||||} |||\) |
| c. Romano: MCMLXII |
| d. romano: DCCXLIV |
| e. romano:\(\bar{\bar{\text{IV}}\text{CCX}}\) DLI |
|
f. Egipcio:  |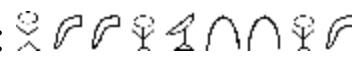
|
g. chino  |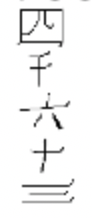
|
h. Maya  |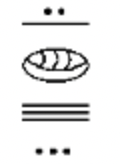
| i.\(4032_{\text{seven}}\) |
| j.\(T6W_{\text{thirteen}}\) |
| k.\(1 \ 111 \ 001 \ 011_{\text{two}}\) |
| l.\(507_{\text{nine}}\) |
Convierte 342 a un numeral en el sistema de numeración o base especificada.
| a. romano | b. Base Siete | c. Egipcio |
| d. Base Dos | e. Chino |
Convertir 838 a:
| a. Base Doce | b. Base Ocho |
| c. Base Cinco | d. Maya |
Convertir 13,595 a Base Doce
Convertir 120,258 a Maya
Cuenta de 620 a 630 en Base Cinco
Indique el número que viene justo antes:
| a.\(173 \ 425 \ 760_{\text{eleven}}\) | b.\(2 \ 010 \ 212 \ 000_{\text{four}}\) |
Indique el número que viene justo después de:
| a.\(539100TE_{\text{twelve}}\) | b.\(3 \ 102 \ 313 \ 444_{\text{five}}\) |
Responde verdadero o falso. Si es falso, explique por qué
| a.\(2_{\text{four}} = 2\) | b.\(3_{\text{four}} = 3_{\text{twelve}}\) | c.\(10_{\text{twelve}} = 10_{\text{five}}\) |
Usando Base Tres bloques, tenías 7 pisos, 10 largos y 5 unidades. ¿Qué número representa esto en
| a. ¿Base Tres? | b. ¿Base Diez? |
Escribe cada número mostrado en notación expandida como un numeral en la base especificada.
| a.\(3 \times 7^{8} + 6 \times 7^{5} + 4 \times 7^{4}\) a Base Siete |
| b.\(1 \times 3^{10} + 2 \times 3^{9} + 2 \times 3^{3}\) a la Base Tres |
Escribe cada número en notación expandida:
| a.\(200 \ 050 \ 030 \ 000_{\text{nine}}\) |
| b.\(1 \ 000 \ 100 \ 001 \ 000_{\text{two}}\) |
Escribe cada número en forma expandida. Después, convierte cada numeral a un número mixto Base Diez con la fracción simplificada.
| a.\(43.3_{\text{nine}}\) | b.\(35.12_{\text{six}}\) |
| c.\(121.21_{\text{three}}\) | d.\(333.333_{\text{five}}\) |
Reescribe de forma expandida a un numeral en la base apropiada.
| a.\(3 \times 4^{2} + 2 \times 4^{0} + 3 \times 4^{-1} + 1 \times 4^{-3}\) |
| b.\(5 \times 11^{3} + 10 \times 11^{1} + 8 \times 11^{-1} + 1 \times 11^{-2}\) |
| c.\(1 \times 2^{2} + 1 x\times 2^{0} + 1 \times 2^{-1} + 1 \times 2^{-4}\) |