4.18: Ángulos y teoremas exteriores
( \newcommand{\kernel}{\mathrm{null}\,}\)
Los ángulos exteriores son iguales a la suma de los interiores remotos.
Ángulos exteriores
Un Ángulo Exterior es el ángulo formado por un lado de un polígono y la extensión del lado adyacente.
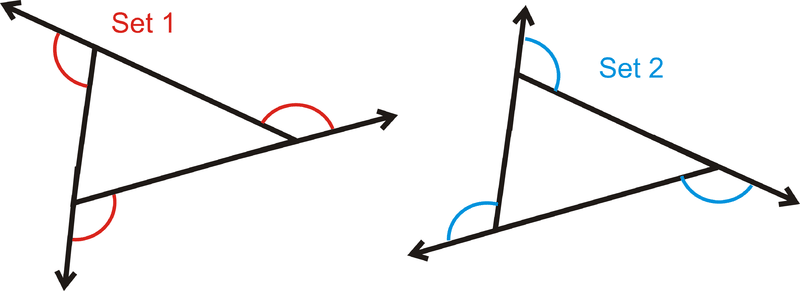
En todos los polígonos, hay dos conjuntos de ángulos exteriores, uno que gira alrededor de las agujas del reloj y el otro va en sentido antihorario.
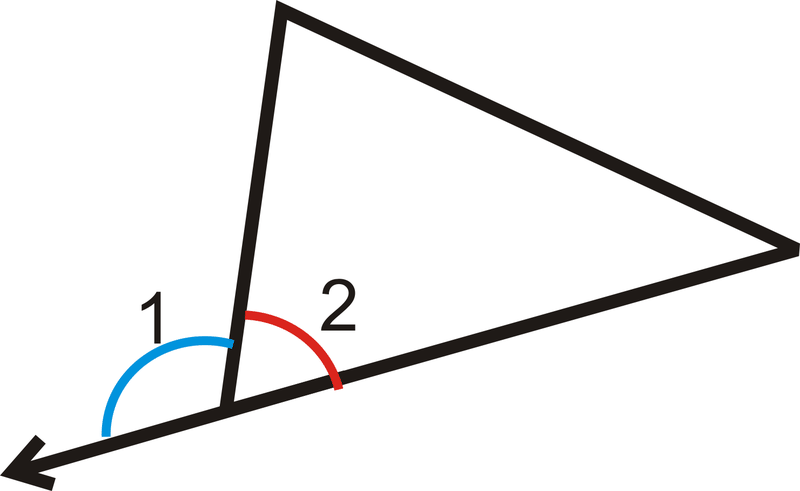
Observe que el ángulo interior y su ángulo exterior adyacente forman un par lineal y se suman a180∘.
m∠1+m∠2=180∘
Hay dos teoremas importantes a conocer que involucran ángulos exteriores: el Teorema de la Suma de Ángulo Exterior y el Teorema de Ángulo Exterior.
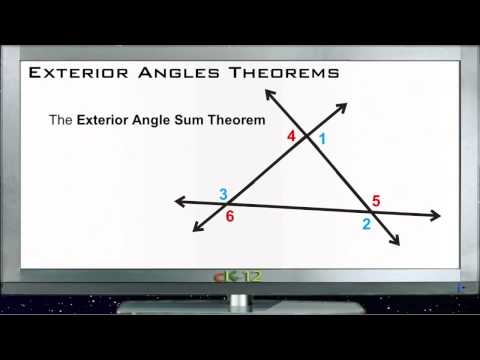
El teorema de la suma de ángulos exteriores establece que los ángulos exteriores de cualquier polígono siempre se sumarán360∘.
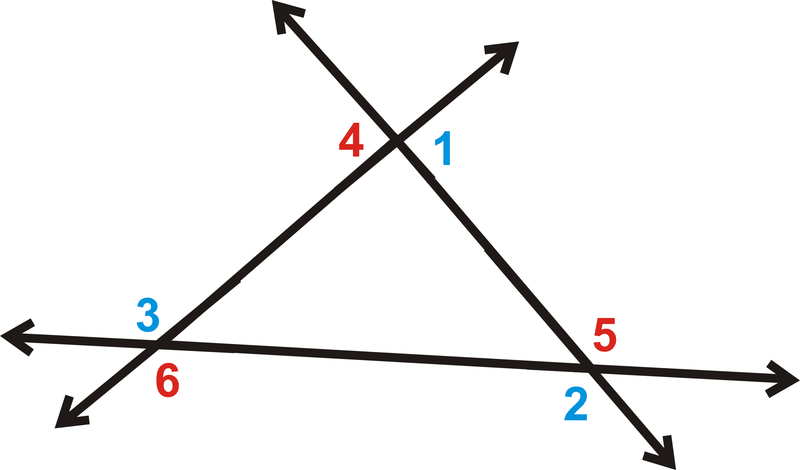
m∠1+m∠2+m∠3=360∘
m∠4+m∠5+m∠6=360∘.
El Teorema del Ángulo Exterior establece que un ángulo exterior de un triángulo es igual a la suma de sus ángulos interiores remotos. (Los ángulos interiores remotos son los dos ángulos interiores en un triángulo que no son adyacentes al ángulo exterior indicado).
 Figura4.18.4
Figura4.18.4m∠A+m∠B=m∠ACD
¿Y si supieras que dos de los ángulos exteriores de un triángulo medían130∘? ¿Cómo pudo encontrar la medida del tercer ángulo exterior?
Ejemplo4.18.1
Dos ángulos interiores de un triángulo son40∘ y73∘. ¿Cuáles son las medidas de los tres ángulos exteriores del triángulo?
Solución
Recuerde que cada ángulo interior forma un par lineal (suma180∘) con un ángulo exterior. Entonces, ya que uno de los ángulos interiores es40∘ eso significa que uno de los ángulos exteriores es140∘ (porque40+140=180). De igual manera, dado que otro de los ángulos interiores es73∘, uno de los ángulos exteriores debe serlo107∘. El tercer ángulo interior no se nos da, pero podríamos averiguarlo usando el Teorema de la Suma del Triángulo. También podemos utilizar el Teorema de la Suma de Ángulo Exterior. Si dos de los ángulos exteriores son140∘ y107∘, entonces el tercer Ángulo Exterior debe ser113∘ desde140+107+113=360.
Entonces, las medidas de los tres ángulos exteriores son 140, 107 y 113.
Ejemplo4.18.2
Encuentra el valorx y la medida de cada ángulo.

Solución
Configura una ecuación usando el Teorema del Ángulo Exterior.
(4x+2)∘+(2x−9)∘⏟remote interior angles=(5x+13)∘⏟exterior angle(6x−7)∘=(5x+13)∘x=20
Sustituye en 20x para encontrar cada ángulo.
[4(20)+2]∘=82∘[2(20)−9]∘=31∘Exteriorangle:[5(20)+13]∘=113∘
Ejemplo4.18.3
Encuentra la medida de∠RQS.
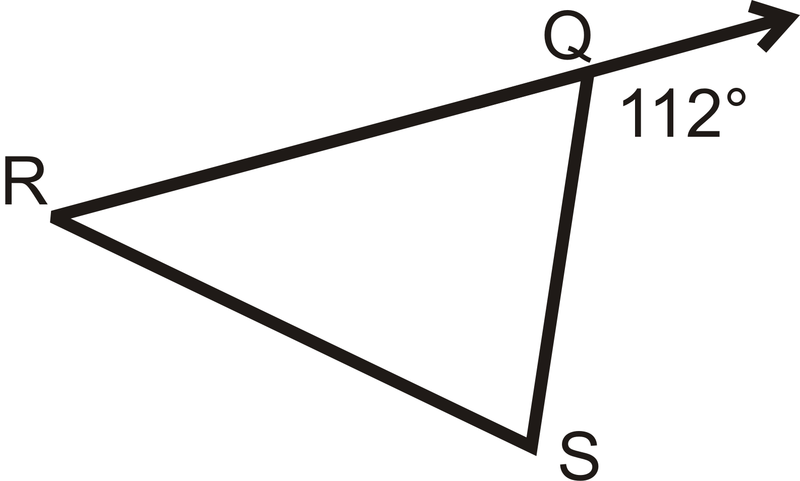 Figura4.18.5
Figura4.18.5Solución
Observe que112∘ es un ángulo exterior deΔRQS y es complementario a∠RQS.
Configure una ecuación para resolver el ángulo faltante.
112∘+m∠RQS=180∘m∠RQS=68∘
Ejemplo4.18.4
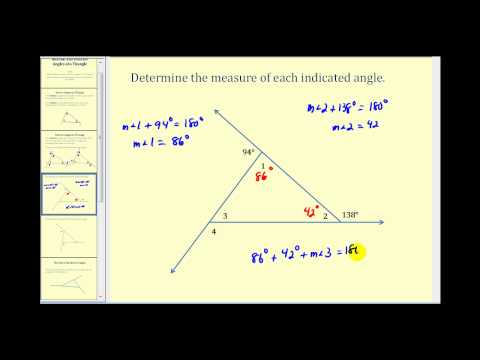
Encuentra las medidas de los ángulos numerados interior y exterior en el triángulo.
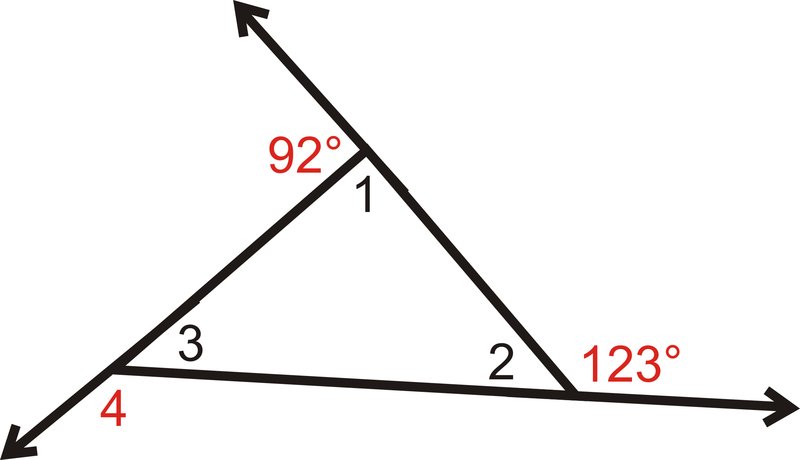 Figura4.18.6
Figura4.18.6Solución
Eso lo sabemosm∠1+92∘=180∘ porque forman un par lineal. Entonces, m\ ángulo 1=88^ {\ circ}\).
Del mismo modo,m∠2+123∘=180∘ porque forman un par lineal. Entonces, m\ ángulo 2=57^ {\ circ}\).
También sabemos que los tres ángulos interiores deben sumar 180^ {\ circ}\) por el Teorema de la Suma del Triángulo.
m∠1+m∠2+m∠3=180∘bytheTriangleSumTheorem.88∘+57∘+m∠3=180m∠3=35∘
Por último,m∠3+m∠4=180∘becausetheyformalinearpair.
35∘+m∠4=180∘m∠4=145∘
Ejemplo4.18.5
¿Cuál es el valor dep en el triángulo de abajo?
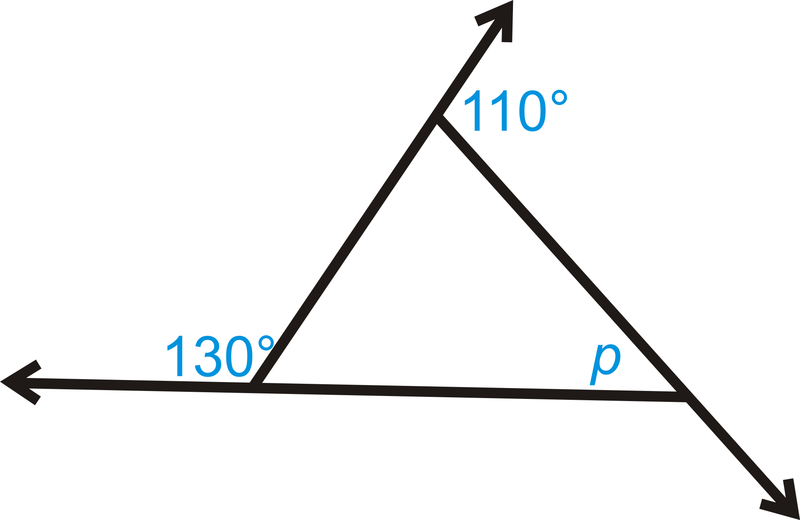
Solución
Primero, necesitamos encontrar el ángulo exterior faltante, al que llamaremosx. Configure una ecuación usando el Teorema de la Suma de Ángulo Exterior.
130∘+110∘+x=360∘x=360∘−130∘−110∘x=120∘
xyp sumar180∘ porque son un par lineal.
x+p=180∘120∘+p=180∘p=60∘
Revisar
Determinarm∠1.
-
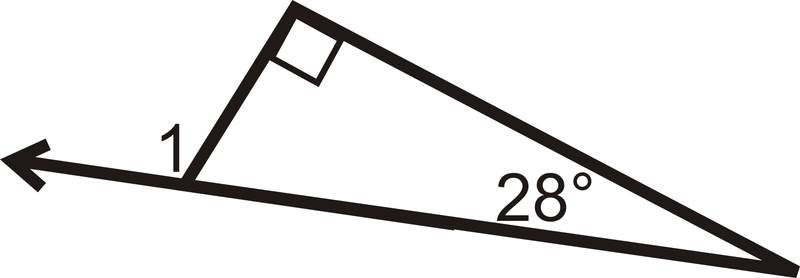
Figura4.18.8 -
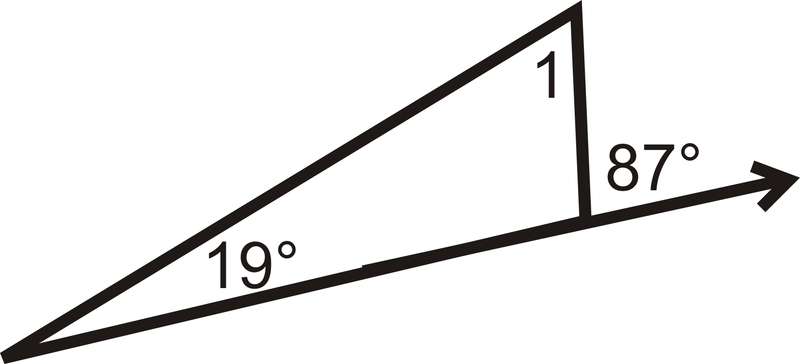
Figura4.18.9 -
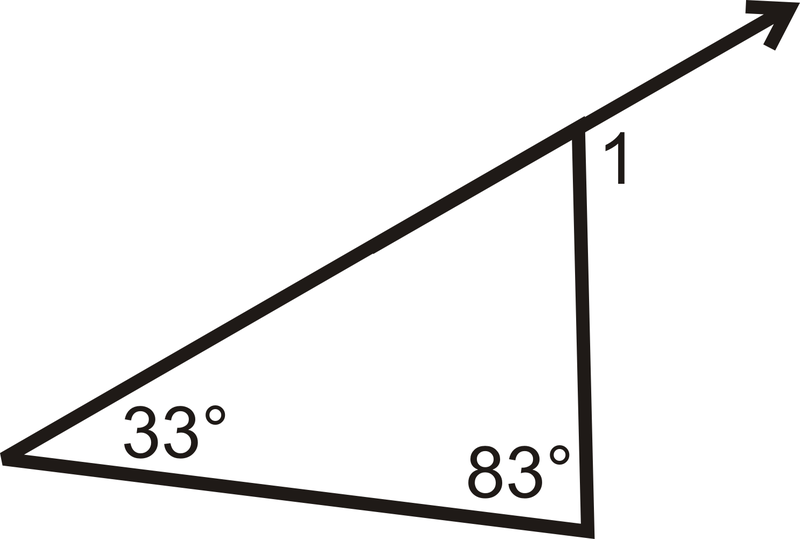
Figura4.18.10 -
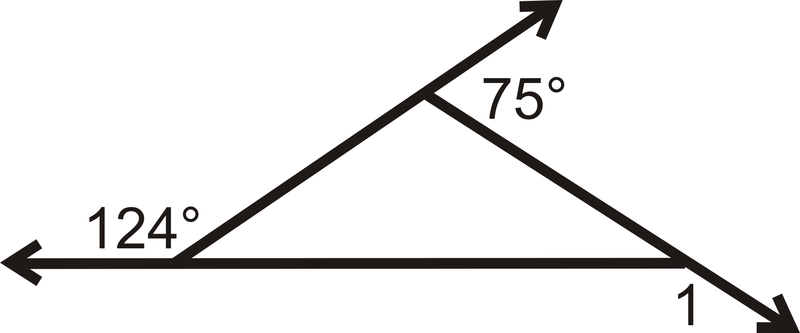
Figura4.18.11 -

Figura4.18.12 -

Figura4.18.13
Utilice la siguiente imagen para los siguientes tres problemas:
- ¿Qué esm∠1+m∠2+m∠3?
- ¿Qué esm∠4+m∠5+m∠6?
- ¿Qué esm∠7+m∠8+m∠9?
Resolver parax.
-
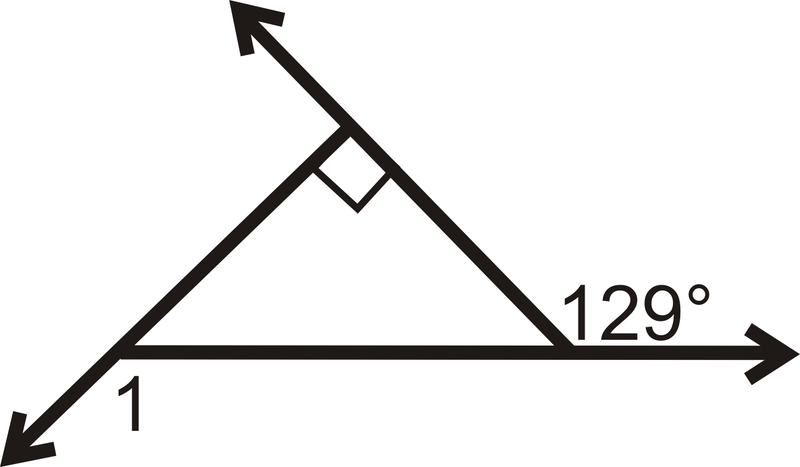
Figura4.18.14 -
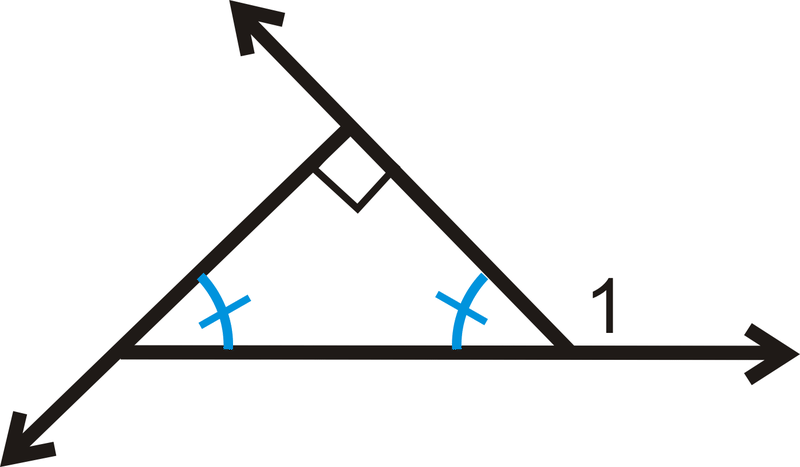
Figura4.18.15 -
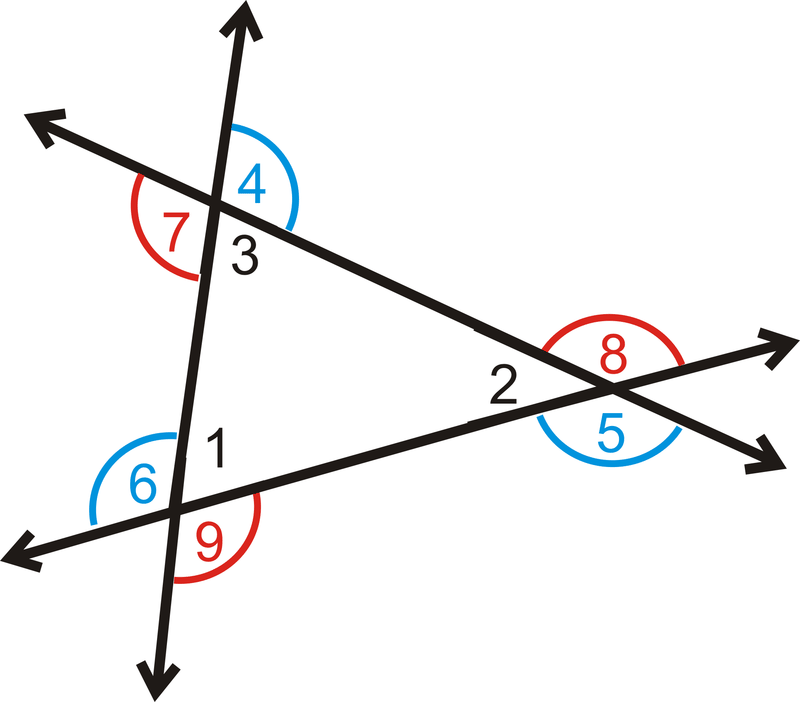
Figura4.18.16
Recursos
vocabulario
| Término | Definición |
|---|---|
| Ángulos exteriores | Un ángulo exterior es el ángulo formado por un lado de un polígono y la extensión del lado adyacente. |
| ángulos interiores | Los ángulos en el interior de un polígono. |
| ángulos interiores remotos | Los ángulos interiores remotos (de un triángulo) son los dos ángulos interiores que no son adyacentes al ángulo exterior indicado. |
| Teorema de suma de triángulo | El Teorema de la Suma del Triángulo establece que los tres ángulos interiores de cualquier triángulo siempre se sumarán180∘. |
| Teorema de la suma de ángulos exteriores | El teorema de la suma de ángulos exteriores establece que los ángulos exteriores de cualquier polígono siempre sumarán 360 grados. |
Recursos adicionales
Elemento interactivo
Video: Ejemplos de Teoremas de Ángulos Exteriores - Básico
Actividades: Teoremas de ángulos exteriores Preguntas de discusión
Ayudas de estudio: Guía de estudio de las relaciones
Práctica: Ángulos y teoremas exteriores
Mundo real: Teorema de ángulos exteriores