8.15: Software de Geometría para Reflexiones
- Page ID
- 107581
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)
( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\id}{\mathrm{id}}\)
\( \newcommand{\Span}{\mathrm{span}}\)
\( \newcommand{\kernel}{\mathrm{null}\,}\)
\( \newcommand{\range}{\mathrm{range}\,}\)
\( \newcommand{\RealPart}{\mathrm{Re}}\)
\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)
\( \newcommand{\Argument}{\mathrm{Arg}}\)
\( \newcommand{\norm}[1]{\| #1 \|}\)
\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)
\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)
\( \newcommand{\vectorA}[1]{\vec{#1}} % arrow\)
\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow\)
\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vectorC}[1]{\textbf{#1}} \)
\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)
\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)
\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)
\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)
\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)
\(\newcommand{\avec}{\mathbf a}\) \(\newcommand{\bvec}{\mathbf b}\) \(\newcommand{\cvec}{\mathbf c}\) \(\newcommand{\dvec}{\mathbf d}\) \(\newcommand{\dtil}{\widetilde{\mathbf d}}\) \(\newcommand{\evec}{\mathbf e}\) \(\newcommand{\fvec}{\mathbf f}\) \(\newcommand{\nvec}{\mathbf n}\) \(\newcommand{\pvec}{\mathbf p}\) \(\newcommand{\qvec}{\mathbf q}\) \(\newcommand{\svec}{\mathbf s}\) \(\newcommand{\tvec}{\mathbf t}\) \(\newcommand{\uvec}{\mathbf u}\) \(\newcommand{\vvec}{\mathbf v}\) \(\newcommand{\wvec}{\mathbf w}\) \(\newcommand{\xvec}{\mathbf x}\) \(\newcommand{\yvec}{\mathbf y}\) \(\newcommand{\zvec}{\mathbf z}\) \(\newcommand{\rvec}{\mathbf r}\) \(\newcommand{\mvec}{\mathbf m}\) \(\newcommand{\zerovec}{\mathbf 0}\) \(\newcommand{\onevec}{\mathbf 1}\) \(\newcommand{\real}{\mathbb R}\) \(\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}\) \(\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}\) \(\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}\) \(\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}\) \(\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}\) \(\newcommand{\laspan}[1]{\text{Span}\{#1\}}\) \(\newcommand{\bcal}{\cal B}\) \(\newcommand{\ccal}{\cal C}\) \(\newcommand{\scal}{\cal S}\) \(\newcommand{\wcal}{\cal W}\) \(\newcommand{\ecal}{\cal E}\) \(\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}\) \(\newcommand{\gray}[1]{\color{gray}{#1}}\) \(\newcommand{\lgray}[1]{\color{lightgray}{#1}}\) \(\newcommand{\rank}{\operatorname{rank}}\) \(\newcommand{\row}{\text{Row}}\) \(\newcommand{\col}{\text{Col}}\) \(\renewcommand{\row}{\text{Row}}\) \(\newcommand{\nul}{\text{Nul}}\) \(\newcommand{\var}{\text{Var}}\) \(\newcommand{\corr}{\text{corr}}\) \(\newcommand{\len}[1]{\left|#1\right|}\) \(\newcommand{\bbar}{\overline{\bvec}}\) \(\newcommand{\bhat}{\widehat{\bvec}}\) \(\newcommand{\bperp}{\bvec^\perp}\) \(\newcommand{\xhat}{\widehat{\xvec}}\) \(\newcommand{\vhat}{\widehat{\vvec}}\) \(\newcommand{\uhat}{\widehat{\uvec}}\) \(\newcommand{\what}{\widehat{\wvec}}\) \(\newcommand{\Sighat}{\widehat{\Sigma}}\) \(\newcommand{\lt}{<}\) \(\newcommand{\gt}{>}\) \(\newcommand{\amp}{&}\) \(\definecolor{fillinmathshade}{gray}{0.9}\)Graficar imágenes dadas preimagen y línea de reflexión. Realizar reflexiones usando Geogebra.
Gráficas de Reflexiones
El triángulo A tiene coordenadas\(E(−5,−5)\),\(F(2,−6)\) y\(G(−2,0)\). Dibuja el triángulo en el plano cartesiano. Refleja la imagen a través del\(y\) eje. Indicar las coordenadas de la imagen resultante.
En geometría, una transformación es una operación que mueve, voltea o cambia una forma para crear una nueva forma. Una reflexión es un ejemplo de una transformación que toma una forma (llamada preimagen) y la voltea a través de una línea (llamada línea de reflexión) para crear una nueva forma (llamada imagen).
Para graficar una reflexión, puedes visualizar lo que sucedería si voltearas la forma a través de la línea.
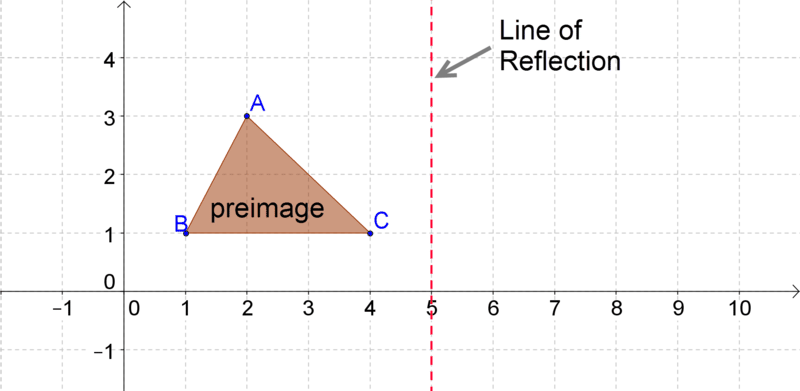
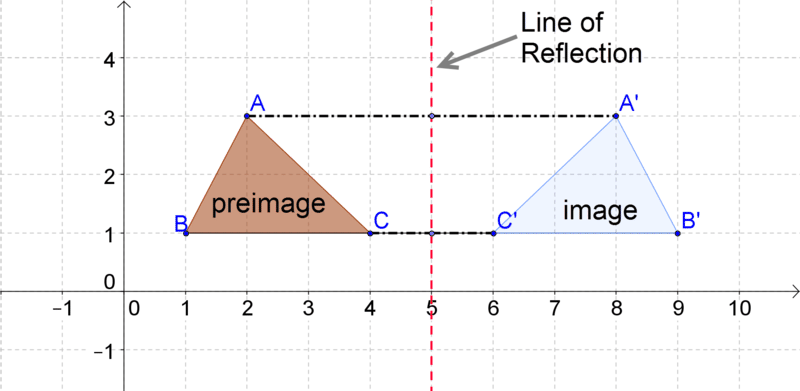
Cada punto de la preimagen estará a la misma distancia de la línea de reflexión que su punto correspondiente en la imagen. Por ejemplo, para el par de triángulos de abajo, ambos\(A\) y\(A′\) están a 3 unidades de distancia de la línea de reflexión.
Para reflexiones comunes, también puedes recordar lo que sucede con sus coordenadas:
- reflexiones a través del\(x\) eje -eje:\(y\) los valores se multiplican por -1.
- reflexiones a través del\(y\) eje -eje:\(x\) los valores se multiplican por -1.
- reflexiones a través de la línea\(y=x\):\(x\) y\(y\) los valores cambian de lugar.
- reflexiones a través de la línea\(y=-x\). \(x\)y\(y\) los valores cambian de lugar y se multiplican por -1.
Conocer las reglas anteriores te permitirá reconocer reflexiones incluso cuando una gráfica no esté disponible.
Dibujemos las reflexiones descritas en los siguientes problemas. Incluir la preimagen si no se da.
- La línea\(\overline{AB}\) dibujada de\((-4, 2)\) a se\((3, 2)\) ha reflejado a través del\(x\) eje.
- \(ABC\)El triángulo se refleja a través de la línea\(y=-x\) para formar la imagen\(A′B′C′\). Dibuja y etiqueta la imagen reflejada.
Ahora, encontremos las coordenadas de la siguiente imagen reflejada y dibujemos la imagen:
El diamante\(ABCD\) se refleja a través de la línea\(y=x\) para formar la imagen\(A′B′C′D′\).
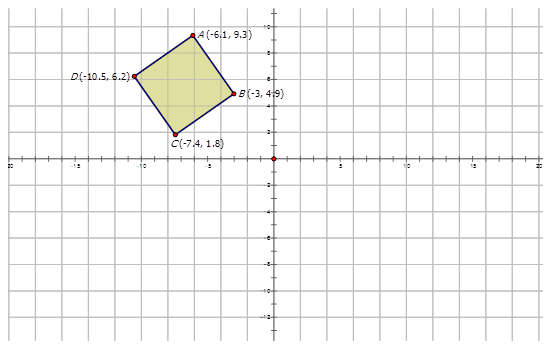
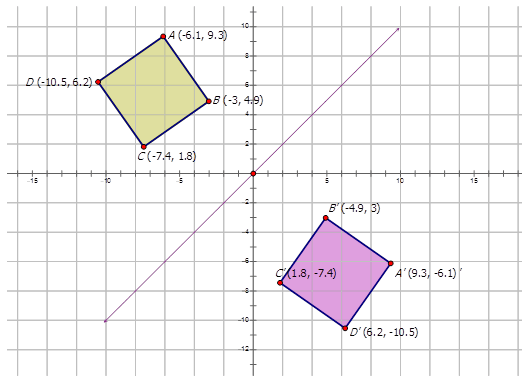
Ejemplo\(\PageIndex{1}\)
Anteriormente, se le dieron las coordenadas de Triángulo\(A\),,, y\(E(−5,−5)\)\(F(2,−6)\), y\(G(−2,0)\), y se le pidió que reflejara la imagen a través del\(y\) eje -eje. ¿Cuáles son las coordenadas del triángulo reflejado?
Solución
Las coordenadas de la nueva imagen (\(B\)) son\(E′(5,−5)\),\(F′(2,−6)\) y\(G′(2,0)\).
Ejemplo\(\PageIndex{2}\)
La línea\(\overline{ST}\) trazada de\((-3, 4)\) a se\((-3, 8)\) ha reflejado a través de la línea\(y=-x\). Dibuja la preimagen y la imagen y etiquete adecuadamente cada una.
Solución
Ejemplo\(\PageIndex{3}\)
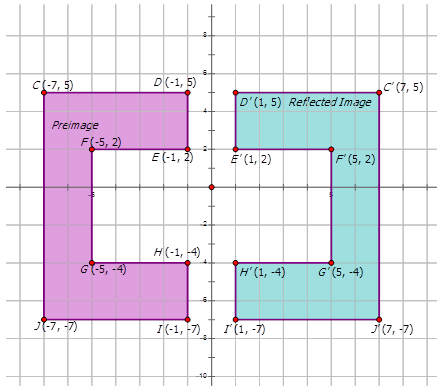
El polígono de abajo se ha reflejado a través del\(y\) eje. Dibuja la imagen reflejada y etiquete adecuadamente cada una.
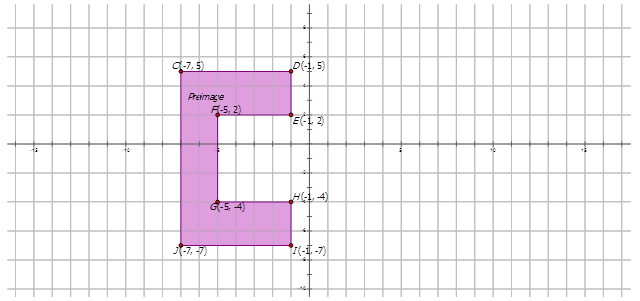
Solución
Ejemplo\(\PageIndex{4}\)
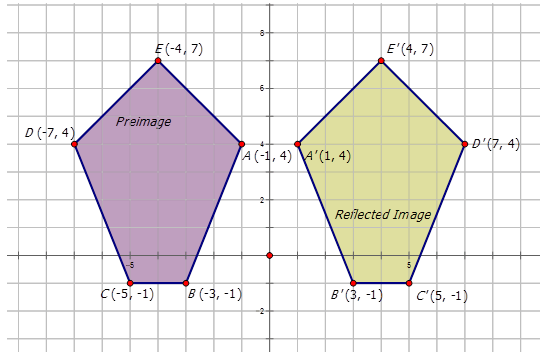
El pentágono púrpura se refleja a través del\(y\) eje para hacer la nueva imagen. Encuentra las coordenadas del pentágono púrpura. En el diagrama, dibuje y etiquete el pentágono reflejado.
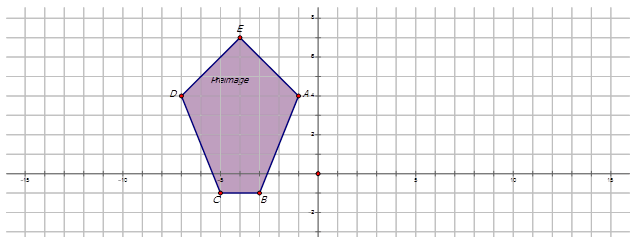
Solución
Opiniones
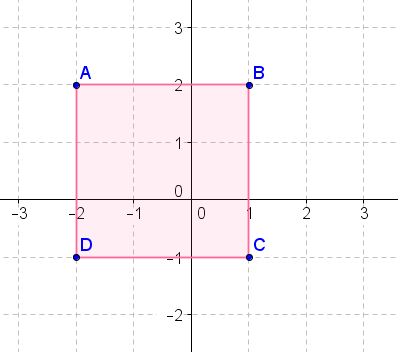
- Refleja la figura anterior a través del\(x\) eje.
- Refleja la figura anterior a través del\(y\) eje.
- Refleja la cifra anterior a través de la línea\(y=x\).
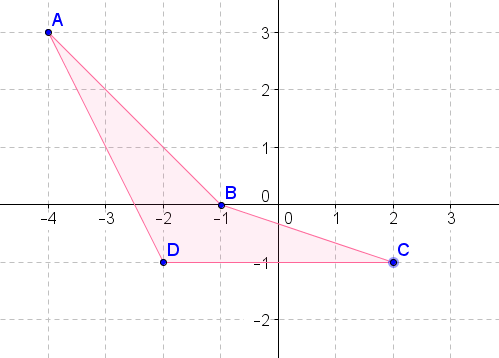
- Refleja la figura anterior a través del\(x\) eje.
- Refleja la figura anterior a través del\(y\) eje.
- Refleja la cifra anterior a través de la línea\(y=x\).
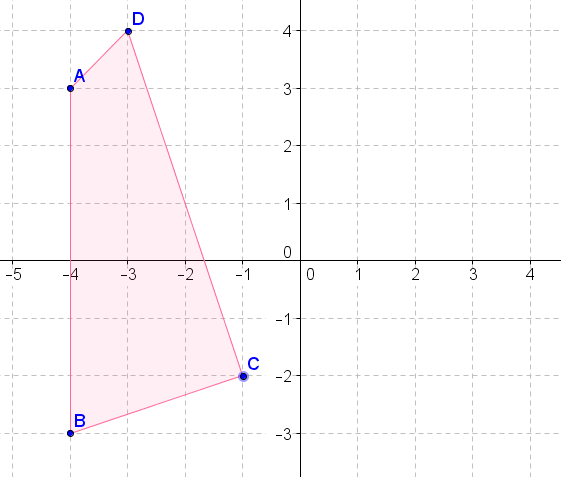
- Refleja la figura anterior a través del\(x\) eje.
- Refleja la figura anterior a través del\(y\) eje.
- Refleja la cifra anterior a través de la línea\(y=x\).
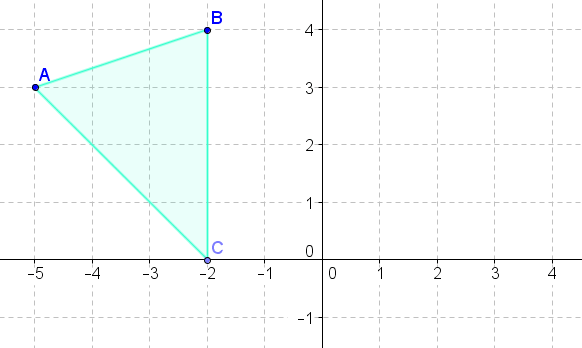
- Refleja la figura anterior a través del\(x\) eje.
- Refleja la figura anterior a través del\(y\) eje.
- Refleja la cifra anterior a través de la línea\(y=x\).
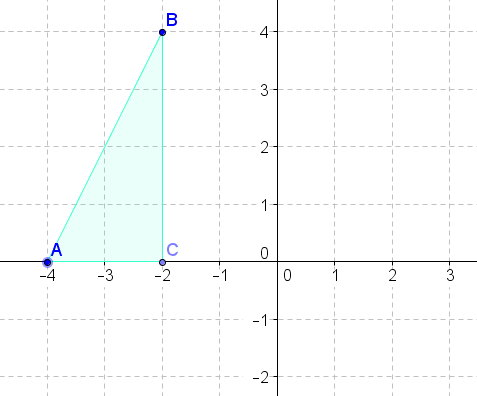
- Refleja la figura anterior a través del\(x\) eje.
- Refleja la figura anterior a través del\(y\) eje.
- Refleja la cifra anterior a través de la línea\(y=x\).
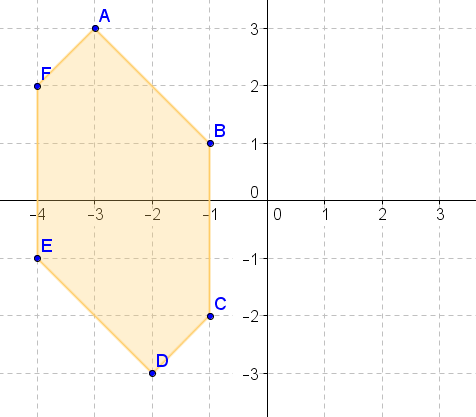
- Refleja la figura anterior a través del\(x\) eje.
- Refleja la figura anterior a través del\(y\) eje.
- Refleja la cifra anterior a través de la línea\(y=x\).
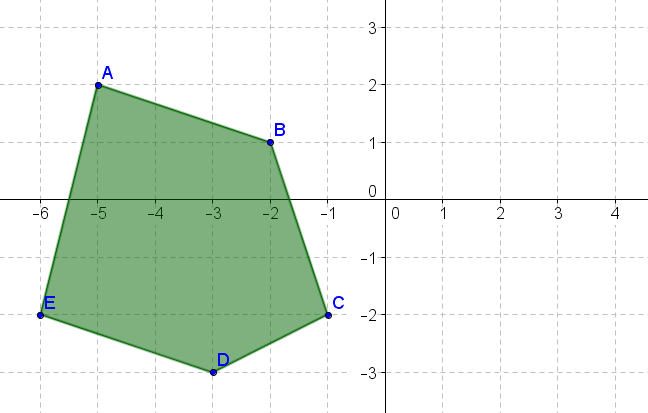
- Refleja la figura anterior a través del\(x\) eje.
- Refleja la figura anterior a través del\(y\) eje.
- Refleja la cifra anterior a través de la línea\(y=x\).
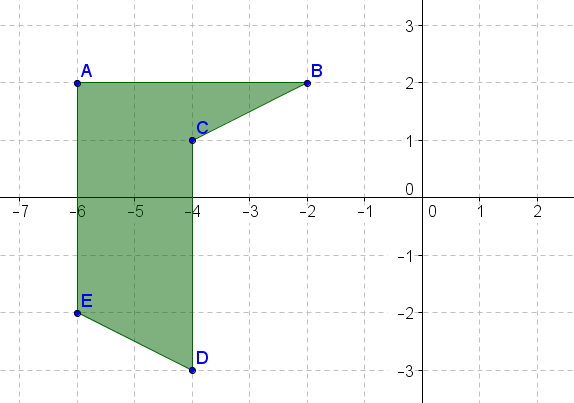
- Refleja la figura anterior a través del\(x\) eje.
- Refleja la figura anterior a través del\(y\) eje.
- Refleja la cifra anterior a través de la línea\(y=x\).
Reseña (Respuestas)
Para ver las respuestas de Revisar, abra este archivo PDF y busque la sección 10.5.
Recursos adicionales
Elemento Interactivo
Práctica: Software de geometría para reflejos