8.9: Notación de traducción
( \newcommand{\kernel}{\mathrm{null}\,}\)
Introducción gráfica a las traducciones de imágenes. Aprende a usar la notación para describir reglas de mapeo, y graficar imágenes dadas preimagen y traducción
Reglas para traducciones
Jack describe una traducción como un punto que se mueve deJ(−2,6) aJ′(4,9). Escribe la regla de mapeo para describir esta traducción para Jack.
En geometría, una transformación es una operación que mueve, voltea o cambia una forma (llamada preimagen) para crear una nueva forma (llamada imagen). Una traslación es un tipo de transformación que mueve cada punto de una figura a la misma distancia en la misma dirección. Las traducciones a menudo se denominan diapositivas. Se puede describir una traducción usando palabras como “movido hacia arriba 3 y más de 5 a la izquierda” o con notación. Hay dos tipos de notación que hay que conocer.
- Parece una notaciónT(3,5). Esta notación le indica que agregue 3 a losx valores y agregue 5 a losy valores.
- La segunda notación es una regla de mapeo de la forma(x,y)→(x−7,y+5). Esta notación le dice que lasy coordenadasx y se traducen ax−7 yy+5.
La notación de reglas de mapeo es la más común.
Elemento Interactivo
Escribamos una regla de mapeo para cada una de las siguientes traducciones:
- Sarah describe una traducción como un punto queP se mueve deP(−2,2) aP′(1,−1).
En general,P(x,y)→P′(x+a,y+b).
En este caso,P(−2,2)→P′(−2+a,2+b) oP(−2,2)→P′(1,−1)
Por lo tanto:
\ (\ begin {array} {rr}
-2+a=1 &\ text {y}\ quad 2+b=-1\
a=3 &\ quad b=-3
\ end {array}\)
La regla es:(x,y)→(x+3,y−3)
- Mikah describe una traducción como punto D en un diagrama que se mueve deD(1,−5) aD′(−3,1).
En general,P(x,y)→P′(x+a,y+b).
En este caso,D(1,−5)→D′(1+a,−5+b) oD(1,−5)→D′(−3,1)
Por lo tanto:
\ (\ begin {array} {rrr}
1+a=-3 &\ text {y} & -5+b=1\\
a=-4 & & b=6
\ end {array}\)
La regla es:(x,y)→(x−4,y+6)
- La traducción de la preimagenA a la imagen traducidaJ en el siguiente diagrama:
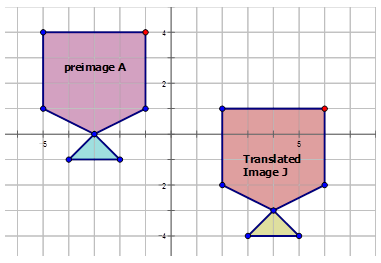
Primero, elige un punto en el diagrama para usar para ver cómo se traduce.

D:(−1,4)D′:(6,1)
D(x,y)→D′(x+a,y+b)
Entonces:D(−1,4)→D′(−1+a,4+b) oD(−1,4)→D′(6,1)
Por lo tanto:
\ (\ begin {array} {rrr}
-1+a=6 &\ text {y} & 4+b=1\\
a=7 & & b=-3
\ end {array}\)
La regla es:(x,y)→(x+7,y−3)
Ejemplo8.9.1
Anteriormente, le dijeron que Jack describió una traducción como un punto queJ se mueve deJ(−2,6) aJ′(4,9). ¿Cuál es la regla de mapeo que describe esta traducción?
Solución
(x,y)→(x+6,y+3)
Ejemplo8.9.2
Escribe la regla de mapeo que representa la traducción del triángulo rojo al triángulo verde traducido en el diagrama de abajo.
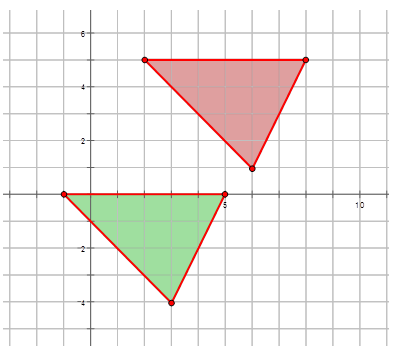
Solución
(x,y)→(x−3,y−5)
Ejemplo8.9.3
El siguiente patrón es parte del papel pintado que se encuentra en el lobby de un hotel. Escriba la regla de mapeo que representa la traducción de un trapecio azul a un trapecio azul traducido que se muestra en el diagrama a continuación.
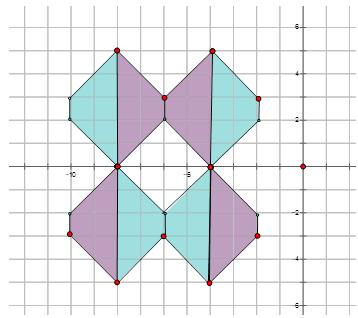
Solución
Si miras de cerca el diagrama a continuación, hay dos pares de trapecios que son traducciones entre sí. Por lo tanto puedes elegir un trapecio azul que sea una traslación del otro y escoger un punto para averiguar cuánto se ha movido la forma para llegar a la posición traducida.
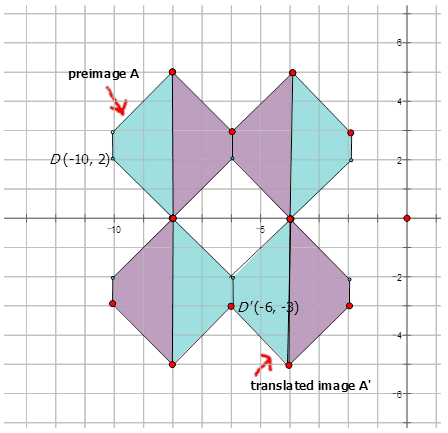
Para esos dos trapecios:(x,y)→(x+4,y−5)
Revisar
Escribe la regla de mapeo para describir el movimiento de los puntos en cada una de las traducciones a continuación.
- S(1,5)→S′(2,7)
- W(−5,−1)→W′(−3,1)
- Q(2,−5)→Q′(−6,3)
- M(4,3)→M′(−2,9)
- B(−4,−2)→B′(2,−2)
- A(2,4)→A′(2,6)
- C(−5,−3)→C′(−3,4)
- D(4,−1)→D′(−4,2)
- Z(7,2)→Z′(−3,6)
- L(−3,−2)→L′(4,−1)
Escribe la regla de mapeo que representa la traducción de la preimagen a la imagen para cada diagrama a continuación.
-
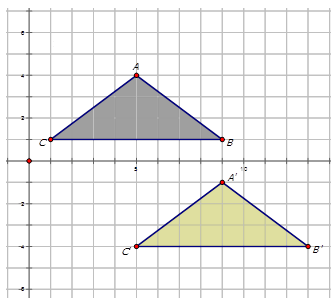
Figura8.9.6 -

Figura8.9.7 -
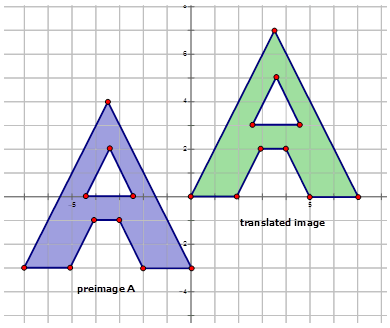
Figura8.9.8 -
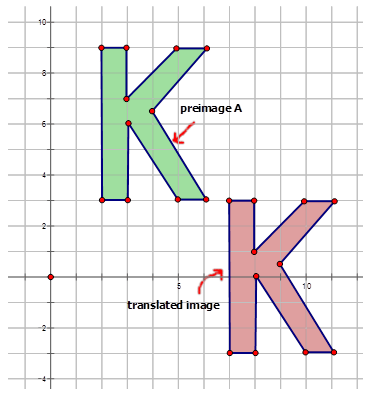
Figura8.9.9 -
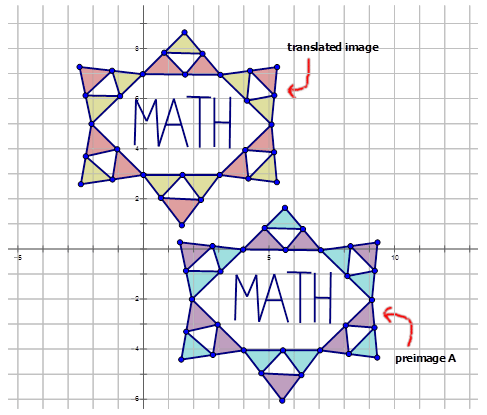
Figura8.9.10
Reseña (Respuestas)
Para ver las respuestas de Revisar, abra este archivo PDF y busque la sección 10.3.
Recursos adicionales
Elemento Interactivo
Video: Cuadrantes de plano de coordenadas
Práctica: Notación de traducción

