1.5: Multiplicar y dividir enteros
- Page ID
- 110411
Al final de esta sección, podrás:
- Multiplicar enteros
- Dividir enteros
- Simplificar expresiones con enteros
- Evaluar expresiones variables con números enteros
- Traducir frases en inglés a expresiones algebraicas
- Usar enteros en aplicaciones
Una introducción más completa a los temas tratados en esta sección se puede encontrar en el capítulo de Preálgebra, Enteros.
Multiplicar enteros
Dado que la multiplicación es una taquigrafía matemática para la adición repetida, nuestro modelo se puede aplicar fácilmente para mostrar la multiplicación de números enteros. Veamos este modelo concreto para ver qué patrones notamos. Usaremos los mismos ejemplos que usamos para sumar y restar. Aquí, usaremos el modelo solo para ayudarnos a descubrir el patrón.
Recordamos que eso\(a\cdot b\) significa sumar\(a,\, b\) tiempos. Aquí, estamos usando el modelo solo para ayudarnos a descubrir el patrón.
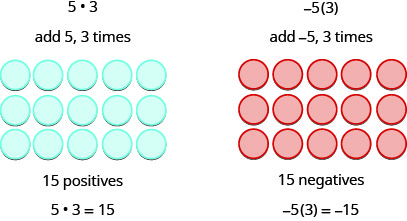
Los siguientes dos ejemplos son más interesantes.
¿Qué significa multiplicar\(5\) por\(−3\)? Significa restar\(5, 3\) tiempos. Mirar a la resta como “quitar”, significa quitar\(5, 3\) tiempos. Pero no hay nada que quitar, así que empezamos por agregar pares neutrales en el espacio de trabajo. Entonces nos llevamos\(5\) tres veces.
En resumen:
\[\begin{array} {ll} {5 \cdot 3 = 15} &{-5(3) = -15} \\ {5(-3) = -15} &{(-5)(-3) = 15} \end{array}\]
Observe que para la multiplicación de dos números firmados, cuando:
- los signos son los mismos, el producto es positivo.
- los signos son diferentes, el producto es negativo.
Vamos a poner todo esto junto en la tabla a continuación.
Para multiplicar dos números firmados:
| Mismos signos | Producto | Ejemplo |
|---|---|---|
| Dos positivos | Positivo | \(7\cdot 4 = 28\) |
| Dos negativos | Positivo | \(-8(-6) = 48\) |
| Distintas señales | Producto | Ejemplo |
|---|---|---|
| Positivos\(\cdot\) negativos | Negativo | \(7(-9) = -63\) |
| \(\cdot\)Positivos negativos | Negativo | \(-5\cdot 10= -50\) |
Multiplicar:
- \(-9\cdot 3\)
- \(-2(-5)\)
- \(4(-8)\)
- \(7\cdot 6\)
- Contestar
-
- \[\begin{array} {ll} {} &{-9\cdot 3} \\ {\text{Multiply, noting that the signs are different, so the product is negative.}} &{-27} \end{array}\]
- \[\begin{array} {ll} {} &{-2(-5)} \\ {\text{Multiply, noting that the signs are same, so the product is positive.}} &{10} \end{array}\]
- \[\begin{array} {ll} {} &{4(-8)} \\ {\text{Multiply, with different signs.}} &{-32} \end{array}\]
- \[\begin{array} {ll} {} &{7\cdot 6} \\ {\text{Multiply, with different signs.}} &{42} \end{array}\]
Multiplicar:
- \(-6\cdot 8\)
- \(-4(-7)\)
- \(9(-7)\)
- \(5\cdot 12\)
- Contestar
-
- \(-48\)
- \(28\)
- \(-63\)
- \(60\)
Multiplicar:
- \(-8\cdot 7\)
- \(-6(-9)\)
- \(7(-4)\)
- \(3\cdot 13\)
- Contestar
-
- \(-56\)
- \(54\)
- \(-28\)
- \(39\)
Cuando multiplicamos un número por\(1\), el resultado es el mismo número. ¿Qué pasa cuando multiplicamos un número por\(−1\)? Multipliquemos un número positivo y luego un número negativo por\(−1\) para ver qué obtenemos.
\[\begin{array} {lll} {} &{-1\cdot 4} &{-1(-3)}\\ {\text{Multiply.}} &{-4} &{3} \\ {} &{-4\text{ is the opposite of 4.}} &{3\text{ is the opposite of } -3} \end{array}\]
Cada vez que multiplicamos un número por\(−1\), ¡obtenemos su opuesto!
MULTIPLICACIÓN POR −1
\[−1a=−a\]
Multiplicar un número por\(−1\) da su opuesto.
Multiplicar:
- \(-1 \cdot 7\)
- \(-1(-11)\)
- Contestar
-
- \[\begin{array} {ll} {} &{-1\cdot 7} \\ {\text{Multiply, noting that the signs are different}} &{-7} \\ {\text{so the product is negative.}} &{-7\text{ is the opposite of 7.}} \end{array}\]
- \[\begin{array} {ll} {} &{-1(-11)} \\ {\text{Multiply, noting that the signs are different}} &{11} \\ {\text{so the product is positive.}} &{11\text{ is the opposite of -11.}} \end{array}\]
Multiplicar:
- \(-1\cdot 9\)
- \(-1\cdot(-17)\)
- Contestar
-
- \(-9\)
- \(17\)
Multiplicar:
- \(-1\cdot 8\)
- \(-1\cdot(-16)\)
- Contestar
-
- \(-8\)
- \(16\)
Dividir enteros
¿Qué pasa con la división? La división es la operación inversa de la multiplicación. Entonces,\(15\div 3=5\) porque\(5 \cdot 3 = 15\). En palabras, esta expresión dice que se\(15\) pueden dividir en tres grupos de cinco cada uno porque sumar cinco tres veces da\(15\). Mira algunos ejemplos de multiplicar enteros, para averiguar las reglas para dividir enteros.
\[\begin{array} {ll} {5\cdot 3 = 15\text{ so }15\div 3 = 5} &{-5(3) = -15\text{ so }-15\div 3 = -5} \\ {(-5)(-3) = 15\text{ so }15\div (-3) = -5} &{5(-3) = -15\text{ so }-15\div (-3) = 5} \end{array}\]
¡La división sigue las mismas reglas que la multiplicación!
Por división de dos números firmados, cuando:
- los signos son los mismos, el cociente es positivo.
- los signos son diferentes, el cociente es negativo.
Y recuerda que siempre podemos verificar la respuesta de un problema de división multiplicando.
Para multiplicación y división de dos números firmados:
- Si los signos son los mismos, el resultado es positivo.
- Si los signos son diferentes, el resultado es negativo.
| Mismos signos | Resultado |
|---|---|
| Dos positivos | Positivo |
| Dos negativos | Positivo |
| Si los signos son los mismos, el resultado es positivo. | |
| Distintas señales | Resultado |
|---|---|
| Positivo y negativo | Negativo |
| Negativo y positivo | Negativo |
| Si los signos son diferentes, el resultado es negativo. | |
- \(-27\div 3\)
- \(-100\div (-4)\)
- Contestar
-
- \[\begin{array} {ll} {} &{-27 \div 3} \\ {\text{Divide, with different signs, the quotient is}} &{-9} \\ {\text{negative.}} &{} \end{array}\]
- \[\begin{array} {ll} {} &{-100 \div (-4)} \\ {\text{Divide, with signs that are the same the}} &{25} \\ {\text{ quotient is negative.}} &{} \end{array}\]
Dividir:
- \(-42\div 6\)
- \(-117\div (-3)\)
- Contestar
-
- \(-7\)
- \(39\)
Dividir:
- \(-63\div 7\)
- \(-115\div (-5)\)
- Contestar
-
- \(-9\)
- \(23\)
Simplificar expresiones con enteros
¿Qué sucede cuando hay más de dos números en una expresión? El orden de operaciones sigue aplicándose cuando se incluyen los negativos. ¿Recuerdas a mi querida tía Sally?
Vamos a probar algunos ejemplos. Simplificaremos las expresiones que utilizan las cuatro operaciones con enteros: suma, resta, multiplicación y división. Recuerda seguir el orden de las operaciones.
Simplificar:
\(7(-2)+4(-7)-6\)
- Contestar
-
\[\begin{array} {ll} {} &{7(-2)+4(-7)-6} \\ {\text{Multiply first.}} &{-14+(-28)-6} \\ {\text{Add.}} &{-42-6} \\{\text{Subtract}} &{-48} \end{array}\]
Simplificar:
\(8(-3)+5(-7)-4\)
- Contestar
-
\(-63\)
Simplificar:
\(9(-3)+7(-8)-1\)
- Contestar
-
\(-84\)
Simplificar:
- \((-2)^{4}\)
- \(-2^{4}\)
- Contestar
-
- \[\begin{array} {ll} {} &{(-2)^{4}} \\ {\text{Write in expanded form.}} &{(-2)(-2)(-2)(-2)} \\ {\text{Multiply}} &{4(-2)(-2)} \\{\text{Multiply}} &{-8(-2)} \\{\text{Multiply}} &{16} \end{array}\]
- \[\begin{array} {ll} {} &{-2^{4}} \\ {\text{Write in expanded form. We are asked to find the opposite of }2^{4}.} &{-(2\cdot 2\cdot 2 \cdot 2)} \\ {\text{Multiply}} &{-(4\cdot 2\cdot 2)} \\{\text{Multiply}} &{-(8\cdot 2)} \\{\text{Multiply}} &{-16} \end{array}\]
Observe la diferencia en las partes (1) y (2). En la parte (1), el exponente significa elevar lo que está entre paréntesis, el\((−2)\) al\(4^{th}\) poder. En la parte (2), el exponente significa elevar solo el\(2\) a la\(4^{th}\) potencia y luego tomar lo contrario.
Simplificar:
- \((-3)^{4}\)
- \(-3^{4}\)
- Contestar
-
- \(81\)
- \(-81\)
Simplificar:
- \((-7)^{2}\)
- \(-7^{2}\)
- Contestar
-
- \(49\)
- \(-49\)
El siguiente ejemplo nos recuerda simplificar primero entre paréntesis.
Simplificar:
\(12-3(9 - 12)\)
- Contestar
-
\[\begin{array} {llll} {} &{12-3(9 - 12)} \\ {\text{Subtract parentheses first}} &{12-3(-3)} \\ {\text{Multiply.}} &{12-(-9)} \\{\text{Multiply}} &{-(8\cdot 2)} \\{\text{Subtract}} &{21} \end{array}\]
Simplificar:
\(17 - 4(8 - 11)\)
- Contestar
-
\(29\)
Simplificar:
\(16 - 6(7 - 13)\)
- Contestar
-
\(52\)
Simplificar:
\(8(-9)\div (-2)^{3}\)
- Contestar
-
\[\begin{array} {ll} {} &{8(-9)\div(-2)^{3}} \\ {\text{Exponents first}} &{8(-9)\div(-8)} \\ {\text{Multiply.}} &{-72\div (-8)} \\{\text{Divide}} &{9} \end{array}\]
Simplificar:
\(12(-9)\div (-3)^{3}\)
- Contestar
-
\(4\)
Simplificar:
\(18(-4)\div (-2)^{3}\)
- Contestar
-
\(9\)
Simplificar:
\(-30\div 2 + (-3)(-7)\)
- Contestar
-
\[\begin{array} {ll} {} &{-30\div 2 + (-3)(-7)} \\ {\text{Multiply and divide left to right, so divide first.}} &{-15+(-3)(-7)} \\ {\text{Multiply.}} &{-15+ 21} \\{\text{Add}} &{6} \end{array}\]
Simplificar:
\(-27\div 3 + (-5)(-6)\)
- Contestar
-
\(21\)
Simplificar:
\(-32\div 4 + (-2)(-7)\)
- Contestar
-
\(6\)
Evaluar expresiones variables con números enteros
Recuerde que evaluar una expresión significa sustituir un número por la variable en la expresión. Ahora podemos usar números negativos así como números positivos.
Cuando\(n=−5\), evalúe:
- \(n+1\)
- \(−n+1\).
- Contestar
-
- \[\begin{array} {ll} {} &{n+ 1} \\ {\text{Substitute}-5\text{ for } n} &{-5+1} \\ {\text{Simplify.}} &{-4} \end{array}\]
- \[\begin{array} {ll} {} &{-n+ 1} \\ {\text{Substitute}-5\text{ for } n} &{-(-5)+1} \\ {\text{Simplify.}} &{-4} \\{\text{Add.}} &{6} \end{array}\]
Cuando\(n=−8\), evalúe:
- \(n+2\)
- \(−n+2\).
- Contestar
-
- \(-6\)
- \(10\)
Cuando\(y=−9\), evalúe:
- \(y+8\)
- \(−y+8\).
- Contestar
-
- \(-1\)
- \(17\)
Evaluar\((x+y)^{2}\) cuándo\(x = -18\) y\(y = 24\).
- Contestar
-
\[\begin{array} {ll} {} &{(x+y)^{2}} \\ {\text{Substitute }-18\text{ for }x \text{ and } 24 \text{ for } y} &{(-18 + 24)^{2}} \\ {\text{Add inside parentheses}} &{(6)^{2}} \\{\text{Simplify.}} &{36} \end{array}\]
Evaluar\((x+y)^{2}\) cuándo\(x = -15\) y\(y = 29\).
- Contestar
-
\(196\)
Evaluar\((x+y)^{3}\) cuándo\(x = -8\) y\(y = 10\).
- Contestar
-
\(8\)
Evaluar\(20 -z \) cuándo
- \(z = 12\)
- \(z = -12\)
- Contestar
-
- \[\begin{array} {ll} {} &{20 - z} \\ {\text{Substitute }12\text{ for }z.} &{20 - 12} \\ {\text{Subtract}} &{8} \end{array}\]
- \[\begin{array} {ll} {} &{20 - z} \\ {\text{Substitute }-12\text{ for }z.} &{20 - (-12)} \\ {\text{Subtract}} &{32} \end{array}\]
Evaluar\(17 - k\) cuándo
- \(k = 19\)
- \(k = -19\)
- Contestar
-
- \(-2\)
- \(36\)
Evaluar\(-5 - b\) cuándo
- \(b = 14\)
- \(b = -14\)
- Contestar
-
- \(-19\)
- \(9\)
Evaluar:
\(2x^{2} + 3x + 8\)cuando\(x = 4\).
- Contestar
-
Sustituto\(4\) de\(x\). Use paréntesis para mostrar la multiplicación.
\[\begin{array} {ll} {} &{2x^{2} + 3x + 8} \\ {\text{Substitute }} &{2(4)^{2} + 3(4) + 8} \\ {\text{Evaluate exponents.}} &{2(16) + 3(4) + 8} \\ {\text{Multiply.}} &{32 + 12 + 8} \\{\text{Add.}} &{52} \end{array}\]
Evaluar:
\(3x^{2} - 2x + 6\)cuando\(x =-3\).
- Contestar
-
\(39\)
Evaluar:
\(4x^{2} - x - 5\)cuando\(x = -2\).
- Contestar
-
\(13\)
Traducir frases a expresiones con enteros
Nuestro trabajo anterior traduciendo el inglés al álgebra también se aplica a frases que incluyen números tanto positivos como negativos.
Traducir y simplificar: la suma de\(8\) y\(−12\), incrementado en\(3\).
- Contestar
-
\[\begin{array} {ll} {} &{\text{the } \textbf{sum} \text{of 8 and -12, increased by 3}} \\ {\text{Translate.}} &{[8 + (-12)] + 3} \\ {\text{Simplify. Be careful not to confuse the}} &{(-4) + 3} \\{\text{brackets with an absolute value sign.}} \\{\text{Add.}} &{-1} \end{array}\]
Traducir y simplificar: la suma de\(9\) y\(−16\), incrementado en\(4\).
- Contestar
-
\((9 + (-16)) + 4 - 3\)
Traducir y simplificar: la suma de\(-8\) y\(−12\), incrementado en\(7\).
- Contestar
-
\((-8 + (-12)) + 7 - 13\)
Cuando introdujimos por primera vez los símbolos de operación, vimos que la expresión puede leerse de varias maneras. Se enumeran en la tabla a continuación.
| \(a−b\) |
|---|
| \ (a−b\)” data-valign="top">\(a\) menos\(b\) la diferencia de\(a\) y\(b\) \(b\) restado de\(a\) \(b\) menos de\(a\) |
¡Ten cuidado de obtener a y b en el orden correcto!
Traducir y luego simplificar
- la diferencia de\(13\) y\(−21\)
- restar\(24\) de\(−19\).
- Contestar
-
- \[\begin{array} {ll} {} &{\text{the } \textbf{difference } \text{of 13 and -21}} \\ {\text{Translate.}} &{13 - (-21)} \\ {\text{Simplify.}} &{34} \end{array}\]
- \[\begin{array} {ll} {} &\textbf{subtract }24 \textbf{ from }-19 \\ {\text{Translate.}} &{-19 - 24} \\ {\text{Remember, subtract b from a means }a - b} &{} \\{\text{Simplify.}} &{-43} \end{array}\]
Traducir y simplificar
- la diferencia de\(14\) y\(−23\)
- restar\(21\) de\(−17\).
- Contestar
-
- \(14 - (-23); 37\)
- \(-17 - 21; -38\)
Traducir y simplificar
- la diferencia de\(11\) y\(−19\)
- restar\(18\) de\(−11\).
- Contestar
-
- \(11 - (-19); 30\)
- \(-11 - 18; -29\)
Una vez más, nuestro trabajo previo al traducir inglés al álgebra se transfiere a frases que incluyen tanto multiplicar como dividir enteros. Recuerda que la palabra clave para multiplicar es “producto” y para división es “cociente”.
Traducir a una expresión algebraica y simplificar si es posible: el producto de\(−2\) y\(14\).
- Contestar
-
\[\begin{array} {ll} {} &{\text{the product of }-2 \text{ and } 14} \\ {\text{Translate.}} &{(-2)(14)} \\{\text{Simplify.}} &{-28} \end{array}\]
Traducir a una expresión algebraica y simplificar si es posible: el producto de\(−5\) y\(12\).
- Contestar
-
\(-5(12); -60\)
Traducir a una expresión algebraica y simplificar si es posible: el producto de\(8\) y\(-13\).
- Contestar
-
\(-8(13); -104\)
Traducir a una expresión algebraica y simplificar si es posible: el cociente de\(−56\) y\(−7\).
- Contestar
-
\[\begin{array} {ll} {} &{\text{the quotient of }-56 \text{ and } -7} \\ {\text{Translate.}} &{-56\div(-7)} \\{\text{Simplify.}} &{8} \end{array}\]
Traducir a una expresión algebraica y simplificar si es posible: el cociente de\(−63\) y\(−9\).
- Contestar
-
\(-63\div (-9); 7\)
Traducir a una expresión algebraica y simplificar si es posible: el cociente de\(−72\) y\(−9\).
- Contestar
-
\(-72\div (-9); 8\)
Usar números enteros en aplicaciones
Vamos a esbozar un plan para resolver aplicaciones. ¡Es difícil encontrar algo si no sabemos lo que estamos buscando o cómo llamarlo! Entonces, cuando resolvemos una aplicación, primero tenemos que determinar cuál es el problema que nos está pidiendo encontrar. Después escribiremos una frase que dé la información para encontrarla. Traduciremos la frase en una expresión y luego simplificaremos la expresión para obtener la respuesta. Por último, resumimos la respuesta en una oración para asegurarnos de que tenga sentido.
Cómo aplicar una estrategia para resolver aplicaciones con números enteros
La temperatura en Urbana, Illinois una mañana era de\(11\) grados. A media tarde, la temperatura había bajado a\(−9\) grados. ¿Cuál fue la diferencia de las temperaturas matutinas y vespertinas?
- Contestar
-
Paso 1. Lee el problema. Asegúrate de que todas las palabras e ideas sean entendidas. Paso 2. Identificar lo que se nos pide encontrar. la diferencia de las temperaturas matutinas y vespertinas Paso 3. Escribe una frase que dé la información para encontrarla. la diferencia de\(11\) y\(-9\) Paso 4. Traducir la frase a una expresión. \(11 - (-9)\) Paso 5. Simplifica la expresión. \(20\) Paso 6. Escribe una oración completa que responda a la pregunta. La diferencia de temperaturas fue de 20 grados.
La temperatura en Anchorage, Alaska una mañana era de\(15\) grados. A media tarde la temperatura había bajado a\(30\) grados bajo cero. ¿Cuál fue la diferencia en las temperaturas matutinas y vespertinas?
- Contestar
-
La diferencia de temperaturas fue de\(45\) grados.
La temperatura en Denver era\(−6\) grados a la hora del almuerzo. Al atardecer la temperatura había bajado a\(−15\) grados. ¿Cuál fue la diferencia en las temperaturas de la hora del almuerzo y del atardecer?
- Contestar
-
La diferencia de temperaturas fue de\(9\) grados.
- Lee el problema. Asegúrese de que todas las palabras e ideas sean entendidas
- Identificar lo que se nos pide encontrar.
- Escribe una frase que dé la información para encontrarla.
- Traducir la frase a una expresión.
- Simplifica la expresión.
- Contesta la pregunta con una oración completa.
El equipo de futbol Mustangs recibió tres penales en el tercer cuarto. Cada penalti les dio una pérdida de quince yardas. ¿Cuál es el número de yardas perdidas?
- Contestar
-
Paso 1. Lee el problema. Asegúrate de que todas las palabras e ideas sean entendidas. Paso 2. Identificar lo que se nos pide encontrar. el número de yardas perdidas Paso 3. Escribe una frase que dé la información para encontrarla. tres veces una penalización\(15\) de yarda Paso 4. Traducir la frase a una expresión. \(3(-15)\) Paso 5. Simplifica la expresión. \(-45\) Paso 6. Escribe una oración completa que responda a la pregunta. El equipo perdió\(45\) yardas.
Los Bears jugaron mal y tuvieron siete penales en el juego. Cada penalti resultó en una pérdida de\(15\) yardas. ¿Cuál es el número de yardas perdidas por penaltis?
- Contestar
-
Los Osos perdieron\(105\) yardas.
Bill usa el cajero automático en el campus porque es conveniente. No obstante, cada vez que lo usa se le cobra una tarifa de $2. El mes pasado utilizó el cajero automático ocho veces. ¿Cuánto fue su tarifa total por usar el cajero automático?
- Contestar
-
Se dedujo de su cuenta corriente una cuota de 16 dólares.
Conceptos clave
- Multiplicación y división de dos números firmados
- Mismas señales: el producto es positivo
- Diferentes señales: el producto es negativo
- Estrategia para Aplicaciones
- Identifique lo que se le pide que encuentre.
- Escribe una frase que dé la información para encontrarla.
- Traducir la frase a una expresión.
- Simplifica la expresión.
- Contesta la pregunta con una oración completa.