7.8: Capítulo 7 Ejercicios de revisión

En los ejercicios 1 - 4, determinar si la afirmación es verdadera o falsa. Justifica tu respuesta con una prueba o un contraejemplo.

1)$\displaystyle ∫e^x\sin(x)\,dx$ no puede ser integrado por partes.

2)$\displaystyle ∫\frac{1}{x^4+1}\,dx$ no se pueden integrar usando fracciones parciales.

Contestar

Falso

3) En la integración numérica, aumentar el número de puntos disminuye el error.

4) La integración por partes siempre puede producir la integral.

Contestar

Falso

En los ejercicios 5 - 10, evaluar la integral utilizando el método especificado.

5)$\displaystyle ∫x^2\sin(4x)\,dx,$ utilizando integración por partes

6)$\displaystyle ∫\frac{1}{x^2\sqrt{x^2+16}}\,dx,$ mediante sustitución trigonométrica

Contestar

$\displaystyle ∫\frac{1}{x^2\sqrt{x^2+16}}\,dx = −\frac{\sqrt{x^2+16}}{16x}+C$

7)$\displaystyle ∫\sqrt{x}\ln x\,dx,$ utilizando integración por partes

8)$\displaystyle ∫\frac{3x}{x^3+2x^2−5x−6}\,dx,$ usando fracciones parciales

Contestar

$\displaystyle ∫\frac{3x}{x^3+2x^2−5x−6}\,dx = \frac{1}{10}\big(4\ln|2−x|+5\ln|x+1|−9\ln|x+3|\big)+C$

9)$\displaystyle ∫\frac{x^5}{(4x^2+4)^{5/2}}\,dx,$ mediante sustitución trigonométrica

10)$\displaystyle ∫\frac{\sqrt{4−\sin^2(x)}}{\sin^2(x)}\cos(x)\,dx,$ usando una tabla de integrales o un CAS

Contestar

$\displaystyle ∫\frac{\sqrt{4−\sin^2(x)}}{\sin^2(x)}\cos(x)\,dx = −\frac{\sqrt{4−\sin^2(x)}}{\sin(x)}−\frac{x}{2}+C$

En los ejercicios 11 - 15, integre usando cualquier método que elija.

11)$\displaystyle ∫\sin^2 x\cos^2 x\,dx$

12)$\displaystyle ∫x^3\sqrt{x^2+2}\,dx$

Contestar

$\displaystyle ∫x^3\sqrt{x^2+2}\,dx = \frac{1}{15}(x^2+2)^{3/2}(3x^2−4)+C$

13)$\displaystyle ∫\frac{3x^2+1}{x^4−2x^3−x^2+2x}\,dx$

14)$\displaystyle ∫\frac{1}{x^4+4}\,dx$

Contestar

$\displaystyle ∫\frac{1}{x^4+4}\,dx = \frac{1}{16}\ln(\frac{x^2+2x+2}{x^2−2x+2})−\frac{1}{8}\tan^{−1}(1−x)+\frac{1}{8}\tan^{−1}(x+1)+C$

15)$\displaystyle ∫\frac{\sqrt{3+16x^4}}{x^4}\,dx$

En los ejercicios 16 - 18, aproxime las integrales usando la regla del punto medio, la regla trapezoidal y la regla de Simpson usando cuatro subintervalos, redondeando a tres decimales.

16) [T]$\displaystyle ∫^2_1\sqrt{x^5+2}\,dx$

Contestar

$M_4=3.312,$
$T_4=3.354,$
$S_4=3.326$

17) [T]$\displaystyle ∫^{\sqrt{π}}_0e^{−\sin(x^2)}\,dx$

18) [T]$\displaystyle ∫^4_1\frac{\ln(1/x)}{x}\,dx$

Contestar

$M_4=−0.982,$
$T_4=−0.917,$
$S_4=−0.952$

En los ejercicios 19 - 20, evaluar las integrales, si es posible.

19) ¿$\displaystyle ∫^∞_1\frac{1}{x^n}\,dx,$para qué valores de$n$ esta integral converge o diverge?

20)$\displaystyle ∫^∞_1\frac{e^{−x}}{x}\,dx$

Contestar

aproximadamente 0.2194

En los ejercicios 21 - 22, considere la función gamma dada por$\displaystyle Γ(a)=∫^∞_0e^{−y}y^{a−1}\,dy.$

21) Demostrar que$\displaystyle Γ(a)=(a−1)Γ(a−1).$

22) Extender para mostrar que$\displaystyle Γ(a)=(a−1)!,$ asumir$a$ es un entero positivo.

El auto más rápido del mundo, el Bugati Veyron, puede alcanzar una velocidad máxima de 408 km/h La gráfica representa su velocidad.

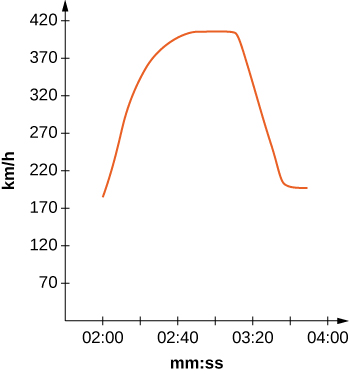
23) [T] Utilice la gráfica para estimar la velocidad cada 20 segundos y ajustar a una gráfica de la forma$v(t)=ae^{bx}\sin(cx)+d.$ (Pista: Considere las unidades de tiempo.)

24) [T] Usando su función del problema anterior, encuentre exactamente hasta dónde viajó el Bugati Veyron en los 1 min 40 seg incluidos en la gráfica.

Contestar

Las respuestas pueden variar. Ej.:$9.405$ km