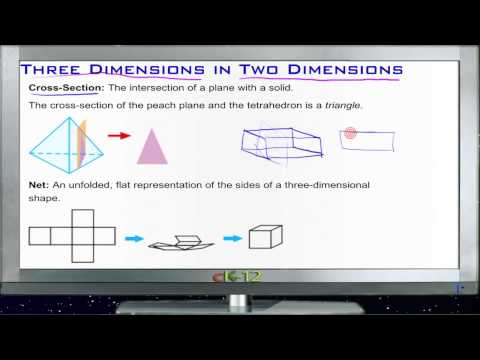
9.3: Secciones transversales y redes
( \newcommand{\kernel}{\mathrm{null}\,}\)
Las secciones transversales son intersecciones de un plano con un sólido. Las redes son representaciones desplegadas y planas de los lados de una forma 3D que permiten encontrar la suma del área de las caras mostrando los bordes y las caras en 2-D.
Si bien nuestro mundo es tridimensional, estamos acostumbrados a modelar y pensar en objetos tridimensionales sobre papel (en dos dimensiones). Hay algunas formas comunes de ayudar a pensar en tres dimensiones en dos dimensiones. Una forma de “ver” una figura tridimensional en un plano bidimensional (como en una hoja de papel o una pantalla de computadora) es usar secciones transversales. Otra forma de “ver” una figura tridimensional en un plano bidimensional es usar una red.
Sección transversal: La intersección de un plano con un sólido.
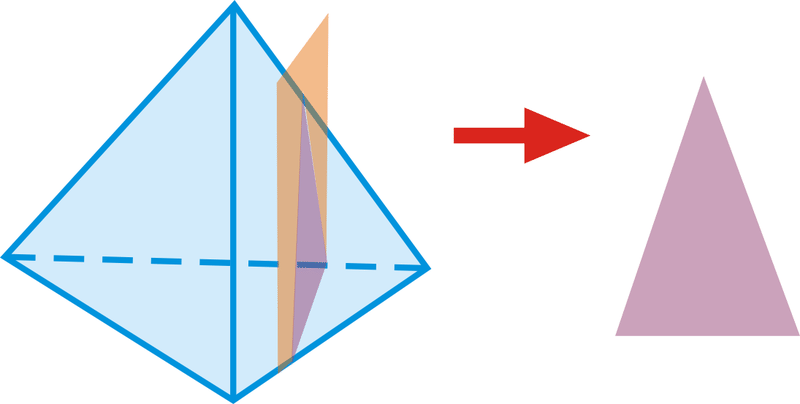
La sección transversal del plano del durazno y el tetraedro es un triángulo.
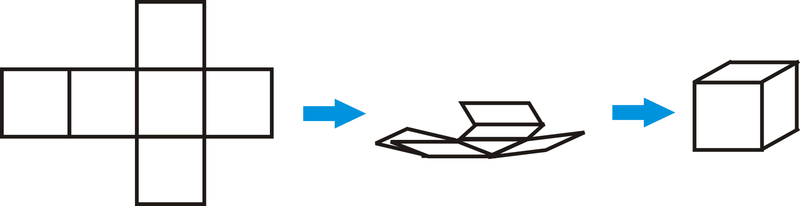
Red: Una representación desplegada y plana de los lados de una forma tridimensional.
Es bueno poder visualizar secciones transversales y redes como los objetos tridimensionales que representan.
¿Y si te dieran una figura tridimensional como una pirámide y quisieras saber cómo sería esa figura en dos dimensiones? ¿Cómo sería una rebanada plana o una representación plana desplegada de ese sólido?
Ejemplo9.3.1
Describir la sección transversal formada por la intersección del plano y el sólido.
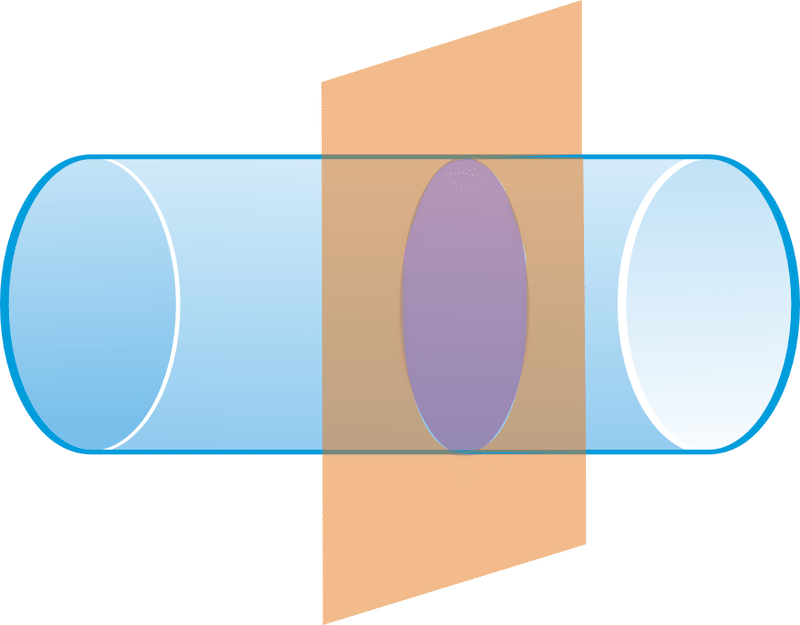
Solución
Círculo
Ejemplo9.3.2
Determinar qué forma está formada por la siguiente red.
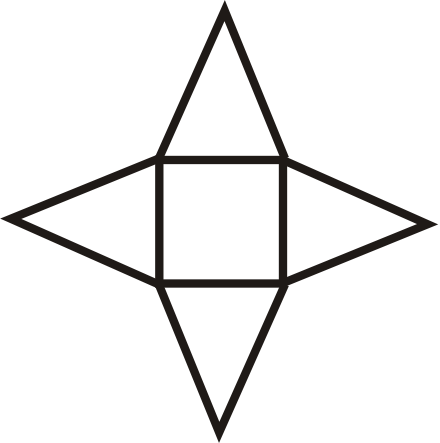
Solución
Pirámide cuadrada
Ejemplo9.3.3
¿Cuál es la forma que forma la intersección del plano y el octaedro regular?
-
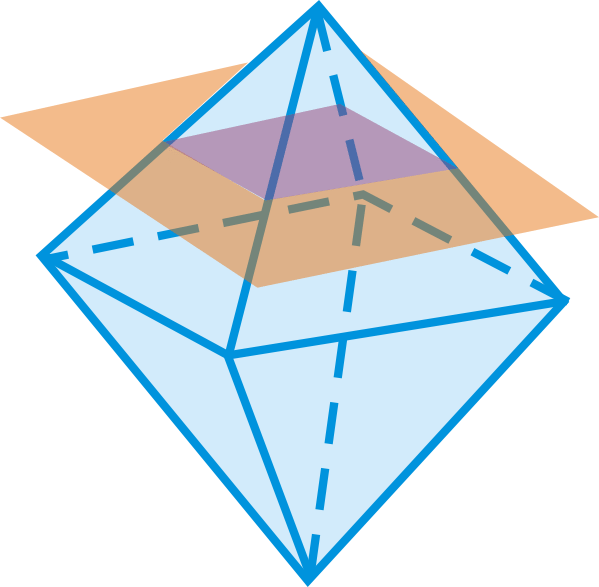
Figura9.3.5 -
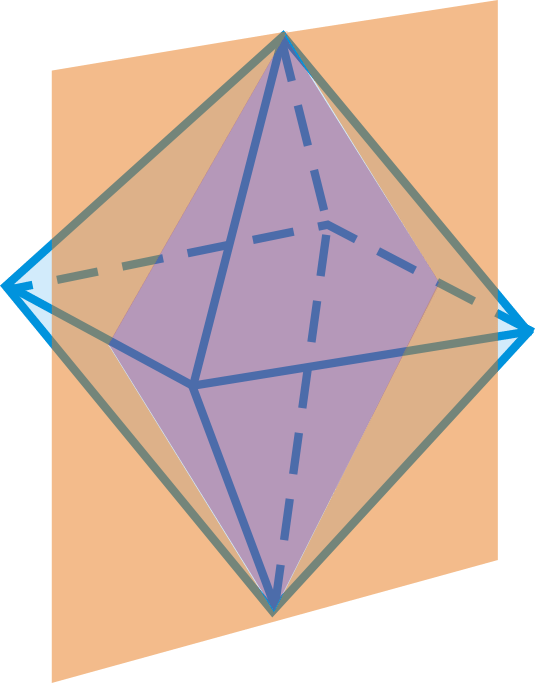
Figura9.3.6 -
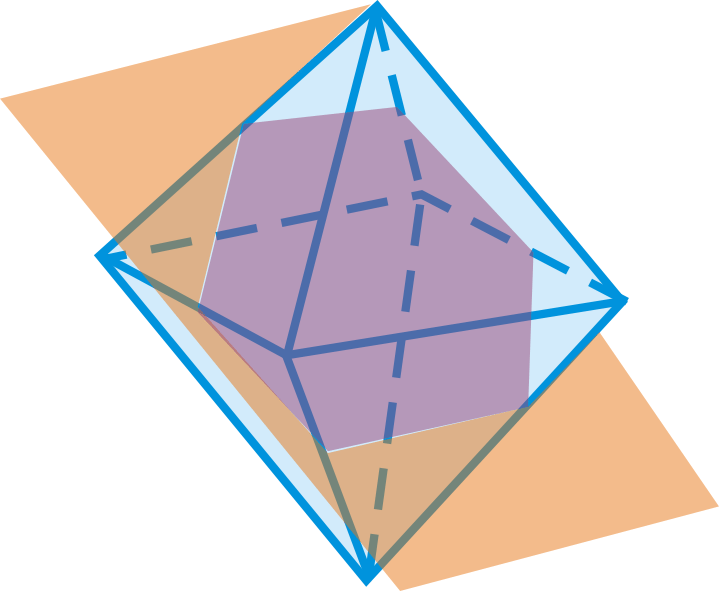
Figura\ (\ pageIndex {7}\
Solución
- Cuadrado
- Rombo
- Hexágono
Ejemplo9.3.4
¿Qué tipo de figura crea esta red?

Solución
La red crea un prisma rectangular.
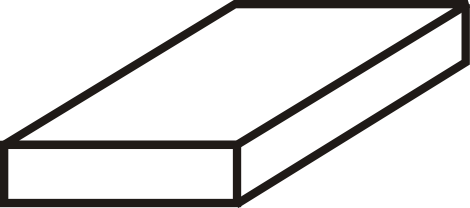
Ejemplo9.3.5
Dibuja una red del prisma triangular derecho debajo.
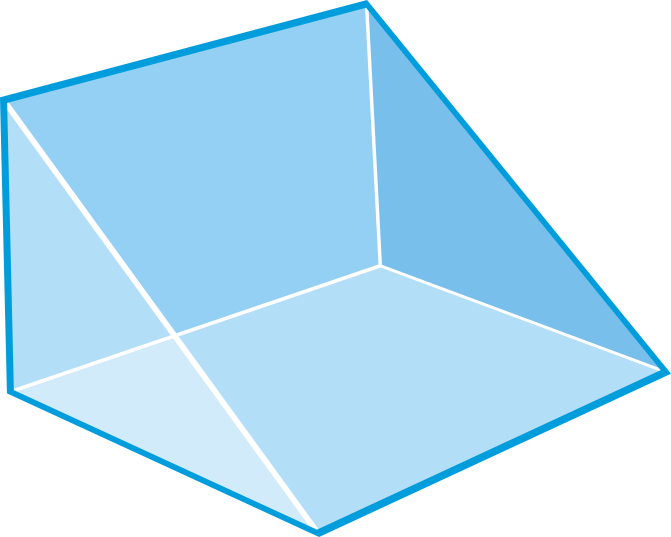
Solución
La red tendrá dos triángulos y tres rectángulos. Los rectángulos son de diferentes tamaños y los dos triángulos son iguales.
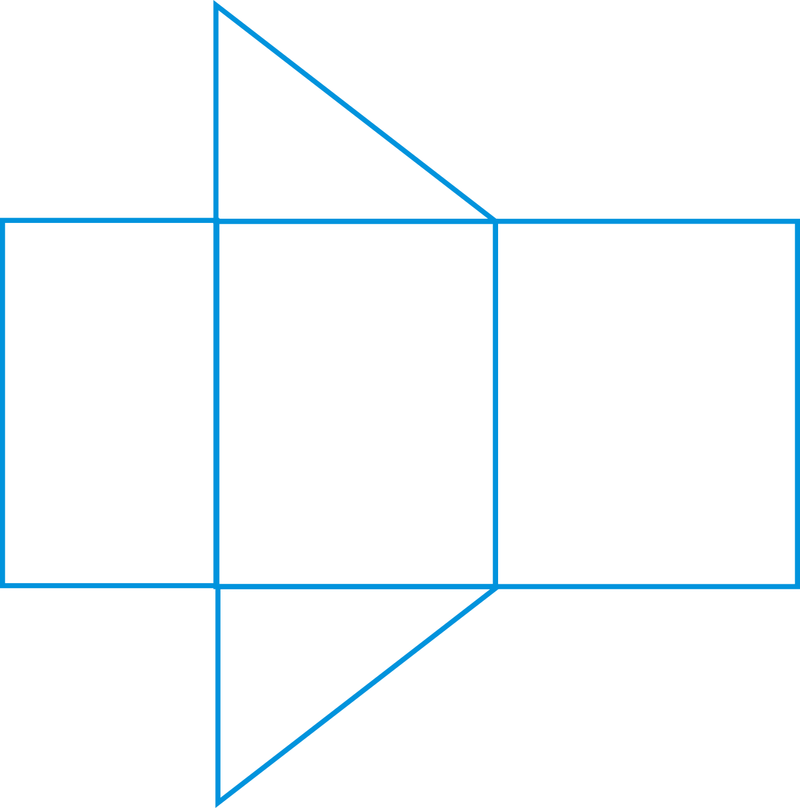
Hay varias redes diferentes de cualquier poliedro. Por ejemplo, esta red podría tener los triángulos en cualquier lugar a lo largo de la parte superior o inferior de los tres rectángulos.
Revisar
Describir la sección transversal formada por la intersección del plano y el sólido.
-
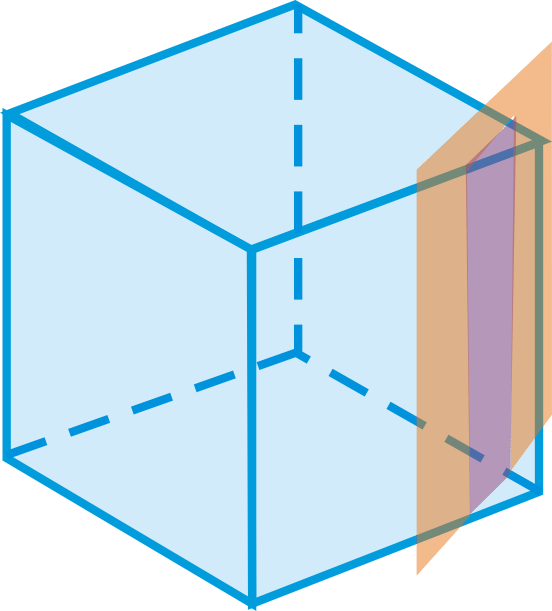
Figura9.3.12 -
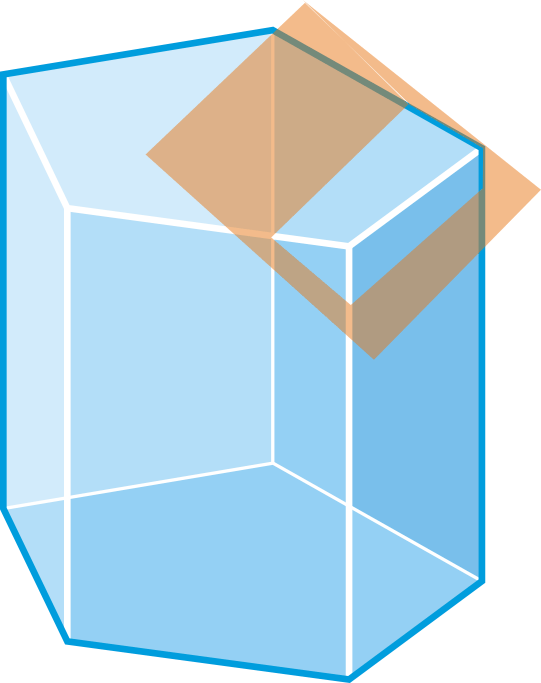
Figura9.3.13
Dibuje la red para los siguientes sólidos.
-
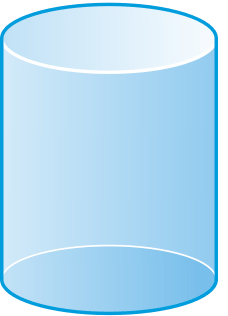
Figura9.3.14 -

Figura9.3.15 -

Figura9.3.16 -
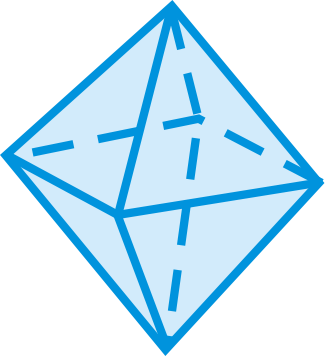
Figura9.3.17 -
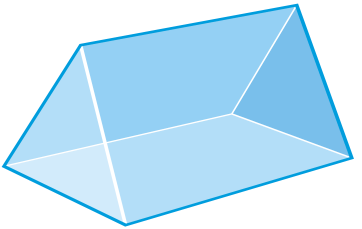
Figura9.3.18 -

Figura9.3.19
Determinar qué forma está formada por las siguientes redes.
-

Figura9.3.20 -

Figura9.3.21 -
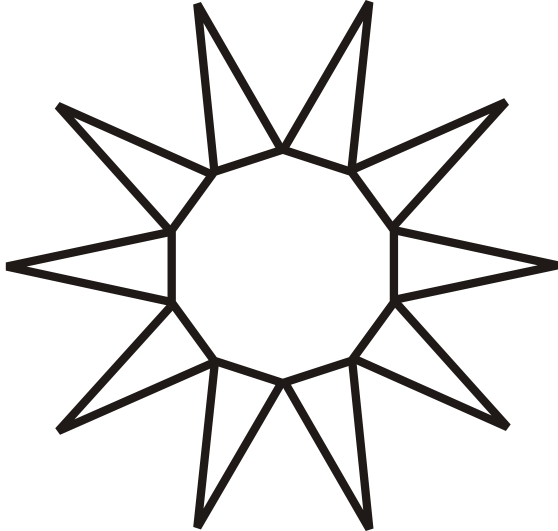
Figura9.3.22 -
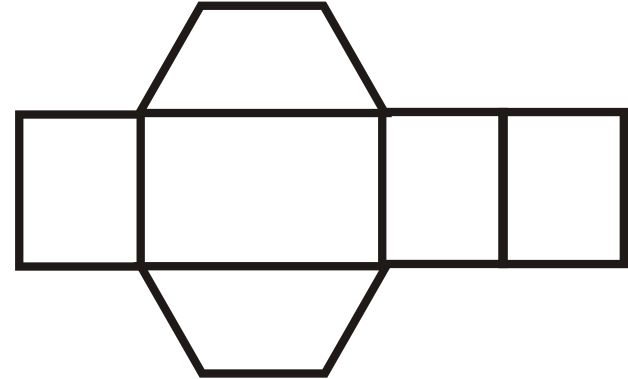
Figura\ (\ pageIndex {23}\
Reseña (Respuestas)
Para ver las respuestas de Revisar, abra este archivo PDF y busque la sección 11.2.
El vocabulario
| Término | Definición |
|---|---|
| sección transversal | La intersección de un plano con un sólido. |
| neto | Una representación desplegada y plana de los lados de una forma tridimensional. |
| sección transversal | Una sección transversal es la intersección de un sólido tridimensional con un plano. |
| Poliedro | Un poliedro es un sólido sin curvas, superficies o aristas. Todas las caras son polígonos y todas las aristas son segmentos de línea. |
| Volumen | El volumen es la cantidad de espacio dentro de los límites de un objeto tridimensional. |