Search

Text Color

Margin Size

Font Type

Enable Dyslexic Font

1.23: Área de Polígonos Regulares

Última actualización

30 oct 2022
Guardar como PDF
- 1.22: Superficie de Sólidos Comunes
- 1.24: Volumen de Sólidos Comunes

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\id}{\mathrm{id}}$ $\newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$ $\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$ $\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\id}{\mathrm{id}}$

$\newcommand{\Span}{\mathrm{span}}$

$\newcommand{\kernel}{\mathrm{null}\,}$

$\newcommand{\range}{\mathrm{range}\,}$

$\newcommand{\RealPart}{\mathrm{Re}}$

$\newcommand{\ImaginaryPart}{\mathrm{Im}}$

$\newcommand{\Argument}{\mathrm{Arg}}$

$\newcommand{\norm}[1]{\| #1 \|}$

$\newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$\newcommand{\Span}{\mathrm{span}}$ $\newcommand{\AA}{\unicode[.8,0]{x212B}}$

$\newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$\newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$\newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vectorC}[1]{\textbf{#1}}$

$\newcommand{\vectorD}[1]{\overrightarrow{#1}}$

$\newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}}$

$\newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}}$

$\newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} }$

$\newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}}$

$\newcommand{\avec}{\mathbf a}$

$\newcommand{\bvec}{\mathbf b}$

$\newcommand{\cvec}{\mathbf c}$

$\newcommand{\dvec}{\mathbf d}$

$\newcommand{\dtil}{\widetilde{\mathbf d}}$

$\newcommand{\evec}{\mathbf e}$

$\newcommand{\fvec}{\mathbf f}$

$\newcommand{\nvec}{\mathbf n}$

$\newcommand{\pvec}{\mathbf p}$

$\newcommand{\qvec}{\mathbf q}$

$\newcommand{\svec}{\mathbf s}$

$\newcommand{\tvec}{\mathbf t}$

$\newcommand{\uvec}{\mathbf u}$

$\newcommand{\vvec}{\mathbf v}$

$\newcommand{\wvec}{\mathbf w}$

$\newcommand{\xvec}{\mathbf x}$

$\newcommand{\yvec}{\mathbf y}$

$\newcommand{\zvec}{\mathbf z}$

$\newcommand{\rvec}{\mathbf r}$

$\newcommand{\mvec}{\mathbf m}$

$\newcommand{\zerovec}{\mathbf 0}$

$\newcommand{\onevec}{\mathbf 1}$

$\newcommand{\real}{\mathbb R}$

$\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$

$\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$

$\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$

$\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$

$\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$

$\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$

$\newcommand{\laspan}[1]{\text{Span}\{#1\}}$

$\newcommand{\bcal}{\cal B}$

$\newcommand{\ccal}{\cal C}$

$\newcommand{\scal}{\cal S}$

$\newcommand{\wcal}{\cal W}$

$\newcommand{\ecal}{\cal E}$

$\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$

$\newcommand{\gray}[1]{\color{gray}{#1}}$

$\newcommand{\lgray}[1]{\color{lightgray}{#1}}$

$\newcommand{\rank}{\operatorname{rank}}$

$\newcommand{\row}{\text{Row}}$

$\newcommand{\col}{\text{Col}}$

$\renewcommand{\row}{\text{Row}}$

$\newcommand{\nul}{\text{Nul}}$

$\newcommand{\var}{\text{Var}}$

$\newcommand{\corr}{\text{corr}}$

$\newcommand{\len}[1]{\left|#1\right|}$

$\newcommand{\bbar}{\overline{\bvec}}$

$\newcommand{\bhat}{\widehat{\bvec}}$

$\newcommand{\bperp}{\bvec^\perp}$

$\newcommand{\xhat}{\widehat{\xvec}}$

$\newcommand{\vhat}{\widehat{\vvec}}$

$\newcommand{\uhat}{\widehat{\uvec}}$

$\newcommand{\what}{\widehat{\wvec}}$

$\newcommand{\Sighat}{\widehat{\Sigma}}$

$\newcommand{\lt}{<}$

$\newcommand{\gt}{>}$

$\newcommand{\amp}{&}$

$\definecolor{fillinmathshade}{gray}{0.9}$

Puede usar una calculadora a lo largo de este módulo.

El edificio del Pentágono abarca $28.7$ acres ( $116,000\text{ m}^2$ ), e incluye $5.1$ acres adicionales ( $21,000\text{ m}^2$ ) como patio central. ^[1] Un pentágono es un ejemplo de un polígono regular.

$El edificio del Pentágono$

Un polígono regular tiene todos los lados de igual longitud y todos los ángulos de igual medida. Debido a esta simetría, un círculo puede ser inscrito, dibujado dentro del polígono tocando cada lado en un punto, o circunscrito, dibujado fuera del polígono que cruza cada vértice. Nos centraremos primero en el círculo inscrito.

Llamemos minúscula al radio del círculo inscrito $r$ ; esta es la distancia desde el centro del polígono perpendicular a uno de los lados. ^[2]

un círculo dentro de un pentágono regular. el círculo toca el centro de los cinco lados del pentágono. — Figura $\PageIndex{1}$ : (izquierda) círculo inscrito (derecha) círculo inscrito con radio.

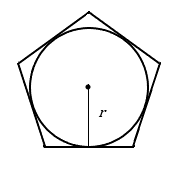
un pentágono regular con un círculo en su interior tocando el punto central de cada lado del pentágono, y un radio dibujado desde el centro recto hacia abajo hasta el centro del lado inferior, etiquetado como r minúscula — Figura $\PageIndex{1}$ : (izquierda) círculo inscrito (derecha) círculo inscrito con radio.

Área de un Polígono Regular (con un radio dibujado al centro de un lado) ^[3]

Para un polígono regular con $n$ lados de longitud $s$ y radio inscrito (interior) $r$ ,

$A=nsr\div2 \nonumber$

Nota: Esta fórmula se deriva de dividir el polígono en triángulos de $n$ igual tamaño y combinar las áreas de esos triángulos.

Ejercicios $\PageIndex{1}$

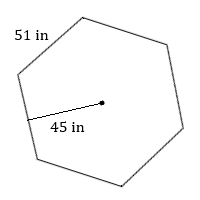
1. Calcular el área de este hexágono regular.

$hexágono regular con lado 51 pulg y radio a un lado 45 in$

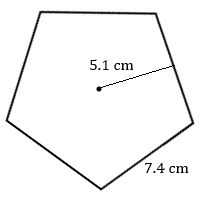
2. Calcular el área de este pentágono regular.

$pentágono regular con lado 7.4 cm y radio a un lado 5.1 cm$

3. Una señal de alto tiene una altura de $30$ inches, and each edge measures $12.5$ inches. Find the area of the sign.

$una señal de alto octogonal$

Contestar

1. $6,900\text{ in}^2$

2. $94\text{ cm}^2$

3. $750\text{ in}^2$

Bien, pero ¿y si conocemos la distancia del centro a una de las esquinas en lugar de la distancia del centro a un borde? Tendremos que imaginar un círculo circunscrito.

Llamemos capital al radio del círculo circunscrito $R$ ; esta es la distancia desde el centro del polígono a uno de los vértices (esquinas).

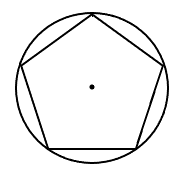
un círculo fuera de un pentágono regular. los cinco puntos de esquina del pentágono tocan el círculo. — Figura $\PageIndex{2}$ : (izquierda) círculo circunscrito y (derecha) círculos circunscritos con círculo mayúscula.

un pentágono regular dentro de un círculo con los cinco puntos de esquina en el círculo, y un radio desde el centro hasta un punto de esquina etiquetado con mayúscula R — Figura $\PageIndex{2}$ : (izquierda) círculo circunscrito y (derecha) círculos circunscritos con círculo mayúscula.

Área de un Polígono Regular (con un radio dibujado en un vértice) ^[4]

Para un polígono regular con $n$ lados de longitud $s$ y radio circunscrito (exterior) $R$ ,

$A=0.25ns\sqrt{4R^2-s^2} \nonumber$

$A=ns\sqrt{4R^2-s^2}\div4 \nonumber$

Nota: Esta fórmula también se deriva de dividir el polígono en triángulos de $n$ igual tamaño y combinar las áreas de esos triángulos. Esta fórmula incluye una raíz cuadrada porque involucra el teorema de Pitágoras.

Ejercicios $\PageIndex{1}$

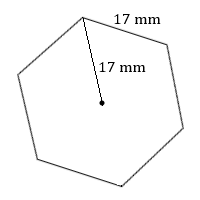
4. Calcular el área de este hexágono regular.

$hexágono regular con lado 17 mm y radio a un vértice de 17 pulg$

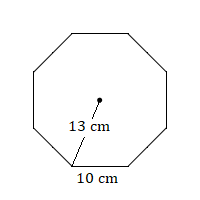
5. Calcular el área de este octágono regular.

$octágono con lado 10 cm y radio a un vértice 13 cm$

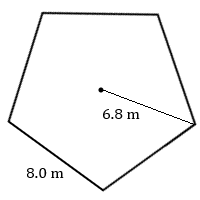
6. Calcular el área de este pentágono regular.

$pentágono regular con lado 8.0 m y radio a un vértice 6.8 m$

Contestar

4. $750\text{ mm}^2$

5. $480\text{ cm}^2$

6. $110\text{ m}^2$

Como sabéis, una figura compuesta es una figura geométrica que se forma uniendo dos o más figuras geométricas básicas. Veamos una figura compuesta formada por un círculo y un polígono regular.

Ejercicio $\PageIndex{1}$

7. La cabeza hexagonal de un perno encaja perfectamente en una tapa circular con un orificio circular con diámetro interior $46\text{ mm}$ as shown in this diagram. Opposite sides of the bolt head are $40\text{ mm}$ apart. Find the total empty area in the hole around the edges of the bolt head.

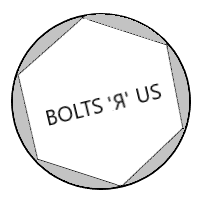
$un hexágono etiquetado como “pernos r us” inscrito en un círculo$

Contestar: $280\text{ mm}^2$ (el área del círculo $\approx1,660\text{ mm}^2$ y el área del hexágono es $1,380\text{ mm}^2$ )

[1]https://en.Wikipedia.org/wiki/The_Pentagon
El radio interior es más comúnmente llamado apotema y etiquetado $a$ , pero estamos tratando de mantener la jerga al mínimo en este libro de texto.
Esta fórmula se escribe más comúnmente como la mitad del apotema multiplicado por el perímetro $A=\dfrac{1}{2}ap$ :
Tu autor creó esta fórmula porque cada otra versión de la misma usa trigonometría, que no estamos cubriendo en este libro de texto.

Ejercicios1.23.1\PageIndex{1}

Ejercicios1.23.1\PageIndex{1}

Ejercicio1.23.1\PageIndex{1}

Support Center

How can we help?

Ejercicios $\PageIndex{1}$

Ejercicios $\PageIndex{1}$

Ejercicio $\PageIndex{1}$