Inicio
Matemáticas
Libro: Cálculo (OpenStax)
3: Derivados
3.10: Capítulo 3 Ejercicios de revisión

3.10: Capítulo 3 Ejercicios de revisión

Última actualización
Guardar como PDF

Page ID: 116631

Edwin “Jed” Herman & Gilbert Strang
OpenStax

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $ $ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $$\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$ $\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$$\newcommand{\AA}{\unicode[.8,0]{x212B}}$

Ejercicios de revisión de capítulos

¿Verdadero o Falso? Justificar la respuesta con una prueba o un contraejemplo.

1) Toda función tiene una derivada.

Contestar: Falso

2) Una función continua tiene una derivada continua.

3) Una función continua tiene una derivada.

Contestar: Falso

4) Si una función es diferenciable, es continua.

En los ejercicios 5 y 6, utilice la definición límite de la derivada para evaluar exactamente la derivada.

5)$f(x)=\sqrt{x+4}$

Contestar: $f'(x) = \dfrac{1}{2\sqrt{x+4}}$

6)$f(x)=\dfrac{3}{x}$

En los ejercicios 7 - 15, encuentra las derivadas de las funciones dadas.

7)$f(x)=3x^3−\dfrac{4}{x^2}$

Contestar: $f'(x) = 9x^2+\frac{8}{x^3}$

9)$f(x)=(4−x^2)^3$

10)$f(x)=e^{\sin x}$

Contestar: $f'(x) = e^{\sin x}\cos x$

11)$f(x)=\ln(x+2)$

12)$f(x)=x^2\cos x+x\tan x$

Contestar: $f'(x) = x\sec^2 x+2x\cos x+\tan x−x^2\sin x $

13)$f(x)=\sqrt{3x^2+2}$

14)$f(x)=\dfrac{x}{4}\sin^{−1}(x)$

Contestar: $f'(x) = \frac{1}{4}\left(\frac{x}{\sqrt{1−x^2}}+\sin^{−1} x\right)$

15)$x^2y=(y+2)+xy\sin x$

En los ejercicios 16 - 18, encuentra las derivadas indicadas de diversos órdenes.

16) Primera derivada de$y=x(\ln x)\cos x$

Contestar: $\dfrac{dy}{dx} = \cos x⋅(\ln x+1)−x(\ln x)\sin x$

17) Tercera derivada de$y=(3x+2)^2$

18) Segunda derivada de$y=4^x+x^2\sin x$

Contestar: $\dfrac{d^2y}{dx^2} = 4^x(\ln 4)^2+2\sin x+4x\cos x−x^2\sin x$

En los ejercicios 19 y 20, encuentra la ecuación de la línea tangente a las siguientes ecuaciones en el punto especificado.

19)$y=\cos^{−1}(x)+x$ en$x=0$

20)$y=x+e^x−\dfrac{1}{x}$ en$x=1$

Contestar: $y = (2+e)x−2$

En los ejercicios 21 y 22, dibuja la derivada de las funciones con las gráficas dadas.

21)

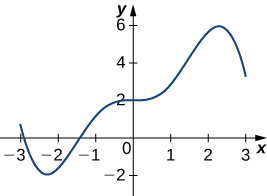
$La función comienza en (−3, 0.5) y disminuye a un mínimo local en (−2.3, −2). Entonces la función aumenta a través de (−1.5, 0) y ralentiza su aumento a través de (0, 2). Luego aumenta lentamente a un máximo local en (2.3, 6) antes de disminuir a (3, 3).$

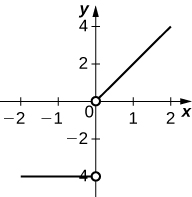
22)

$La función disminuye linealmente desde (−1, 4) hasta el origen, momento en el que aumenta como x^2, pasando por (1, 1) y (2, 4).$

Contestar: $La función es la línea recta y = −4 hasta x = 0, punto en el que se convierte en una línea recta que comienza en el origen con pendiente 2. No hay ningún valor asignado para esta función en x = 0.$

Las preguntas 22 y 23 se refieren al nivel del agua en Ocean City, Nueva Jersey, en enero, que se puede aproximar por$w(t)=1.9+2.9\cos(\frac{π}{6}t),$ dónde$t$ se mide en horas después de la medianoche, y la altura se mide en pies.

22) Encuentra y grafica la derivada. ¿Cuál es el significado físico?

23) Encuentra$w′(3).$ ¿Cuál es el significado físico de este valor?

Contestar: $w′(3)=−\frac{2.9π}{6}$. A las 3 de la mañana la marea está bajando a un ritmo de 1.514 pies/hr.

Las preguntas 24 y 25 consideran las velocidades del viento del huracán Katrina, que afectó a Nueva Orleans, Luisiana, en agosto de 2005. Los datos se muestran en una tabla.

Horas después de la medianoche, 26 de agosto	Velocidad del viento (mph)
1	45
5	75
11	100
29	115
49	145
58	175
73	155
81	125
85	95
107	35

Velocidades del Viento del Huracán KatrinaOrigen: news.nationalgeographic.com/n... _timeline.html.

24) Utilizando la tabla, estimar la derivada de la velocidad del viento a la hora 39. ¿Cuál es el significado físico?

25) Estimar la derivada de la velocidad del viento a la hora 83. ¿Cuál es el significado físico?

Contestar: $−7.5.$La velocidad del viento está disminuyendo a una velocidad de 7.5 mph/hr